Algorithm for Cubic Nonuniform B-Spline Curve Interpolation

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
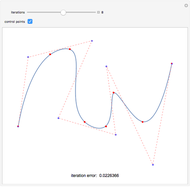
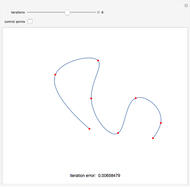
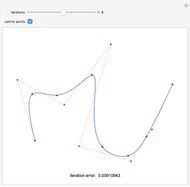
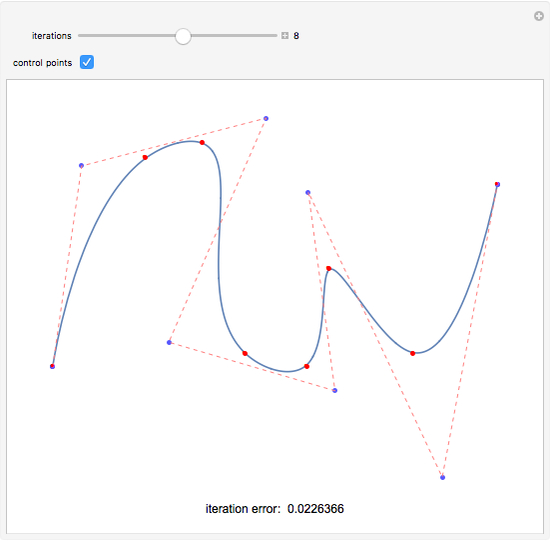
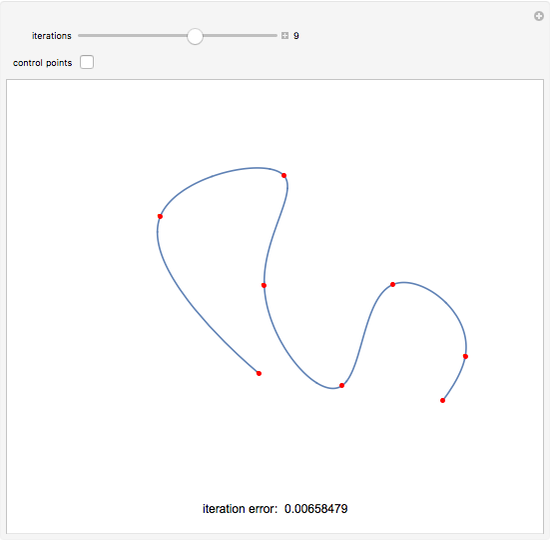
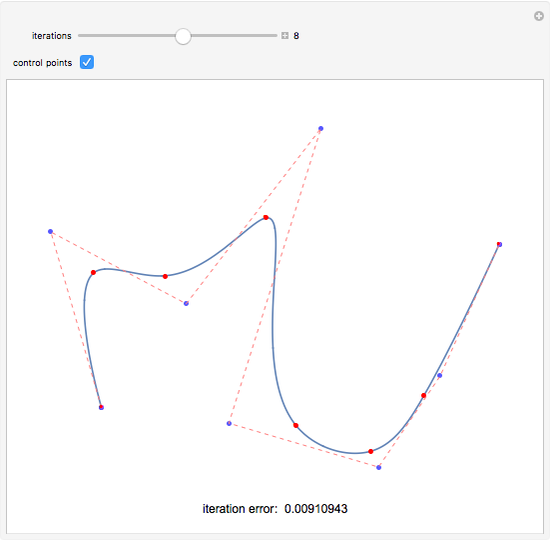
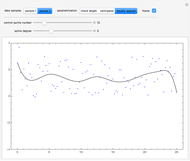
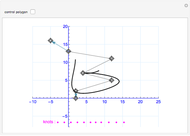
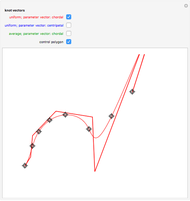
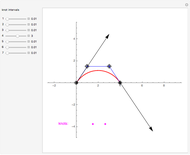
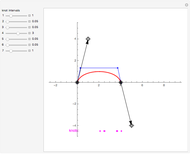
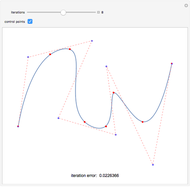
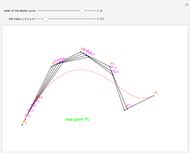
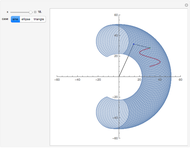
This Demonstration shows how to interpolate a set of points in the plane via a cubic nonuniform B-spline curve and progressive-iterative approximation (PIA) technique. See Details for full implementation details.
Contributed by: Shutao Tang (December 2015)
(Northwestern Polytechnical University, Xi'an, China)
Open content licensed under CC BY-NC-SA
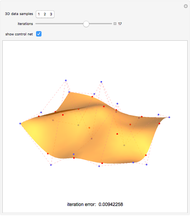
Snapshots
Details
There are three main steps in the PIA algorithm.
1. Compute the knot vector  via the chord-length parametrization
via the chord-length parametrization
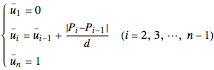
where
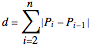 .
.
Then define the knot vector
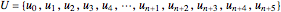 ,
,
where
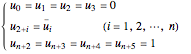
2. Do the iteration.
At the beginning of the iteration, let
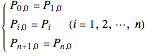
First, generate a cubic nonuniform B-spline curve 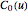 by the control points
by the control points 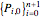 :
:
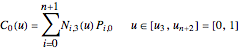 .
.
The first adjustment of the 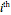 control point is
control point is
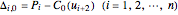 ,
,
then let
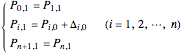
Again, this gives a cubic nonuniform B-spline curve 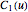 with the control points
with the control points 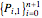 , that is
, that is
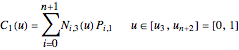 .
.
In general, if the 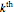 cubic nonuniform B-spline
cubic nonuniform B-spline 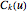 results from iterating
results from iterating  times, let the
times, let the 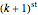 adjustment of the
adjustment of the 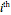 control point be
control point be
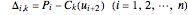 .
.
Also, let
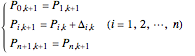
This gives the 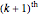 cubic nonuniform B-spline curve
cubic nonuniform B-spline curve 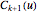 via the control points
via the control points 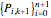 .
.
Finally, the set of curves 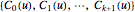 is defined. Lin [1] has proved that these curves converge to the points, namely that
is defined. Lin [1] has proved that these curves converge to the points, namely that
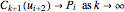 ,
, 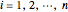
3. The error is given by
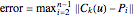 .
.
Reference
[1] 蔺宏伟, 王国瑾, 董辰世. 用迭代非均匀 B-spline 曲线(曲面)拟合给定点集[J]. 中国科学, 2003, 33(10), pp. 912–923.
H. Lin et al., "Use Iterative Non-Uniform B-Spline Curve (Surface) to Fitting Given Point Set [J]." China Science, 33(10), 2003 pp. 912–923 (in Chinese).
Permanent Citation