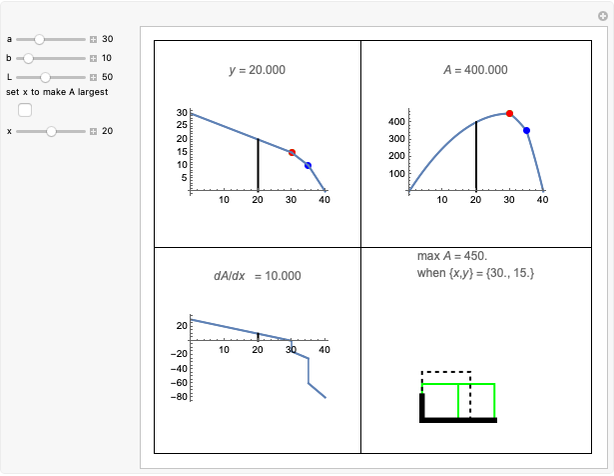
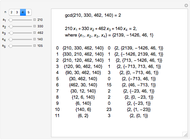
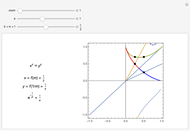
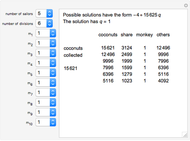
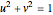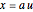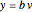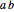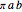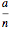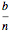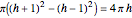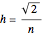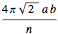Area of an Ellipse
Initializing live version

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
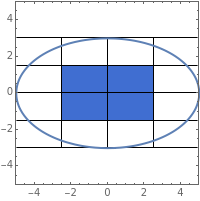
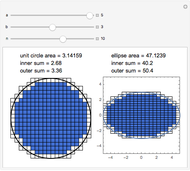
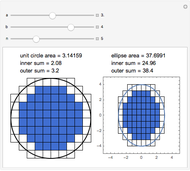
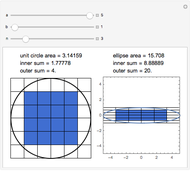
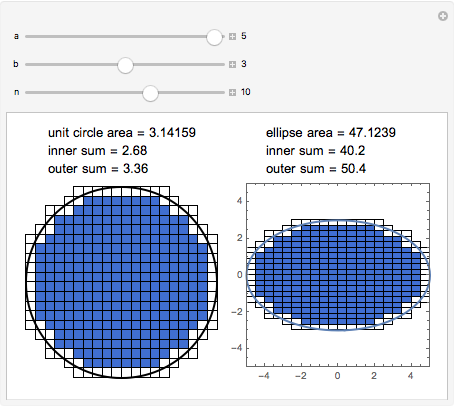
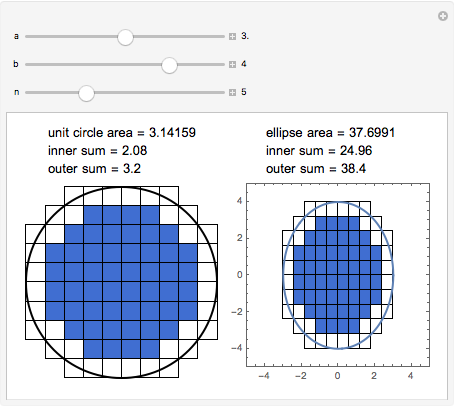
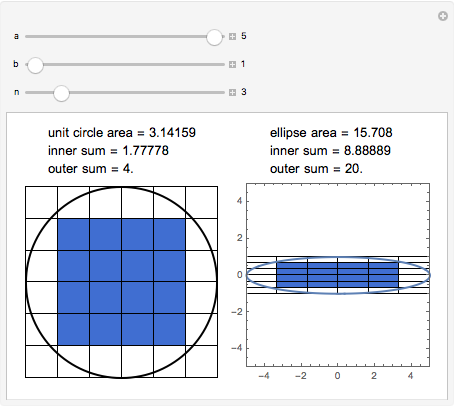
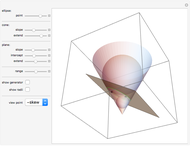
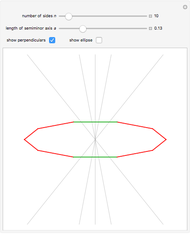
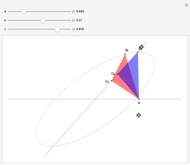
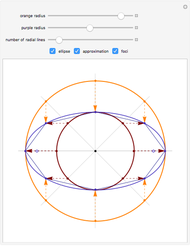
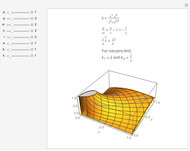
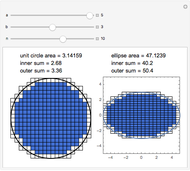
What is the area of an ellipse with equation 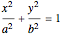 ?
?
Contributed by: Roger B. Kirchner (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
R. B. Kirchner, "The Area of the Ellipse," The American Mathematical Monthly, 68(7), 1961 p. 653.
Permanent Citation