Astronomical CCD Camera Noise
Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
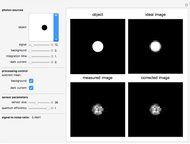
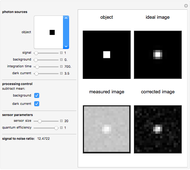
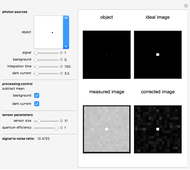
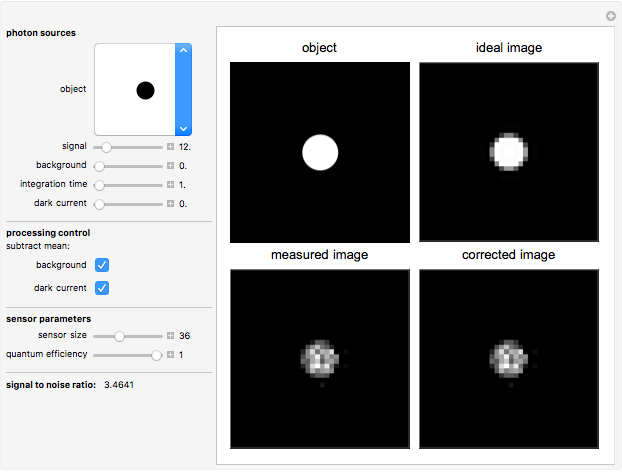
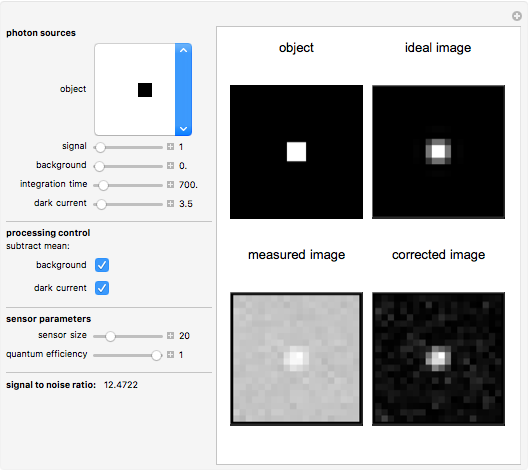
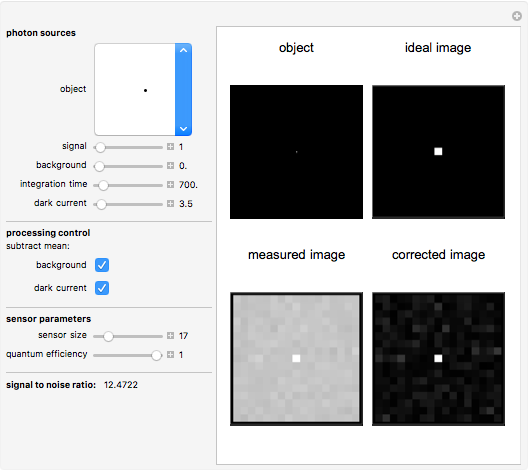
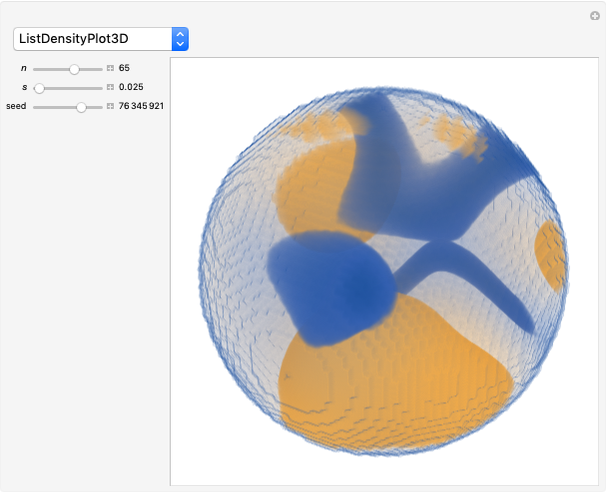
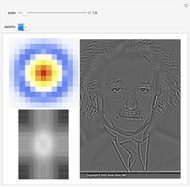
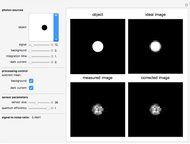
The charged-coupled device (CCD) has become the de facto standard sensor type for astronomical photography. A CCD counts photons; there is noise in counting experiments (an uncertainty in the number of counts measured each time the experiment runs in identical conditions) that scales as the square root of the number of counts. This Demonstration shows CCD noise in the context of astronomical imaging from the ground. The object is what a perfect telescope would see from above the atmosphere, the ideal image is what a CCD would sense if there was no noise and measuring the photons was perfect, the measured image is what a real CCD would measure, and the corrected image is the measured image compensating for the mean values of the background and the dark current.
Contributed by: John Holland (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
There are three noise sources present.
The signal itself has a noise that can be reduced (but never eliminated) by increasing the integration time (you can see the noise on the signal by turning down the background and dark currents to zero; see Snapshot 1).
The sky background is due to the atmosphere and objects behind the target object. It can be reduced by reducing the amount of atmosphere the light has to pass through (and has the added benefit to astronomers of reducing turbulence that distorts light) or increasing the integration time, which increases the signal-to-noise ratio.
The dark current is generated in the sensor itself because the sensor has a nonzero temperature; the energy associated with this temperature can liberate electrons from their lattice in the semiconductor material of which the CCD is composed. Dark current always exists, even when a sensor is in total darkness. It is measured in bits per pixel per second because it is independent of the total quantum efficiency of the system and is not due to radiation from outside.
The noise derived from reading out from the sensor has been ignored but that always exists in real sensors.
Permanent Citation
"Astronomical CCD Camera Noise"
http://demonstrations.wolfram.com/AstronomicalCCDCameraNoise/
Wolfram Demonstrations Project
Published: March 7 2011
Aperture Synthesis with VLA and ALMA Radio Telescope Arrays
Gerald Cecil
Astronomical Clock
Erik Mahieu
Astronomical Data
Don Braffitt
Astronomical Units of Length
Sarah Lichtblau
Frequency-Filtered Random Noise
Enrique Zeleny
Think Big, Really Big!
John Kiehl
Stellar Parallax
Kevin Jiang and John Stucky
Finding the Vanishing Points of Sample Images
Simon Kim
Retina and LGN: Early Vision Using Gaussian Filters
Flip Phillips
Image Histograms of the Four Seasons
Daniel de Souza Carvalho
