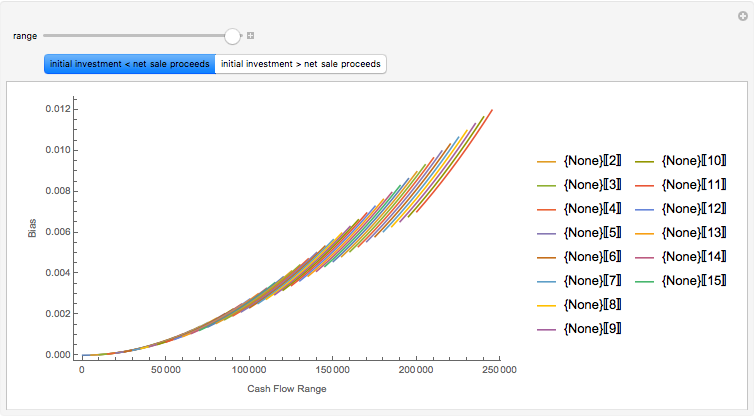
Capitalization Rate Probability

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
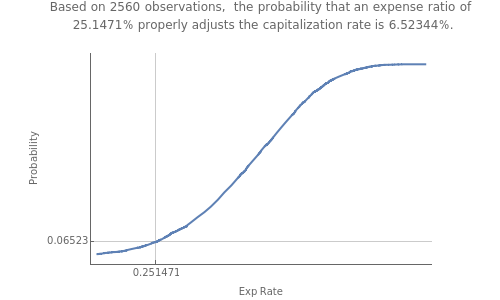
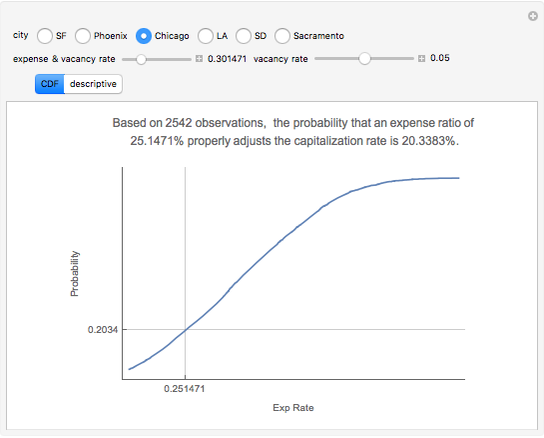
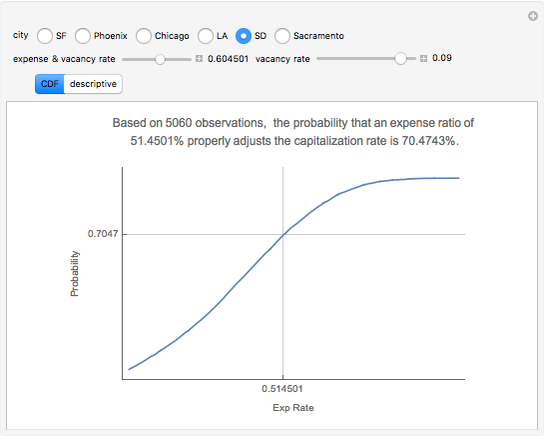
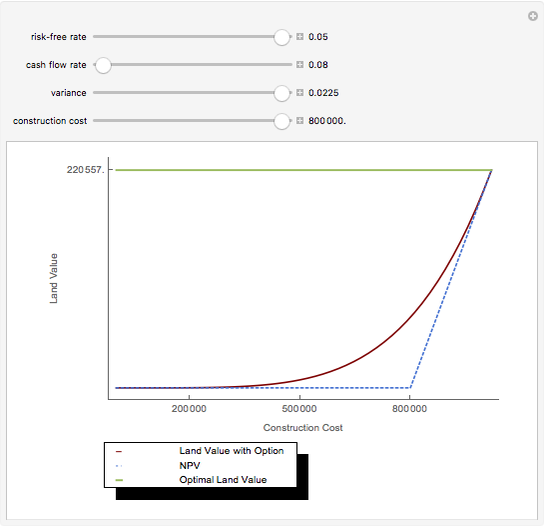
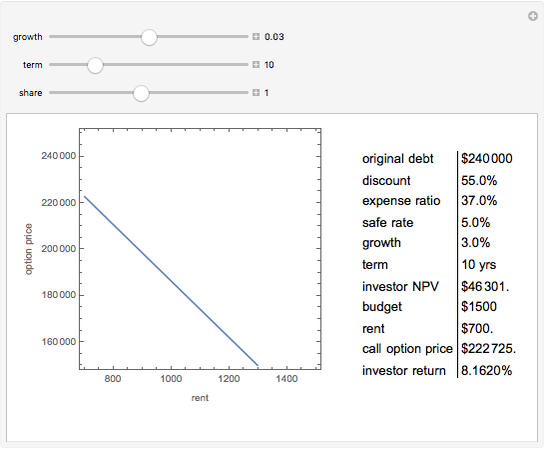
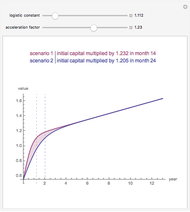
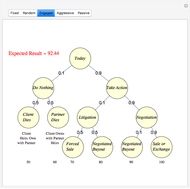
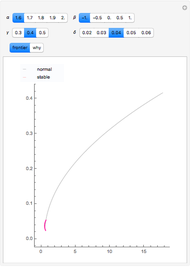
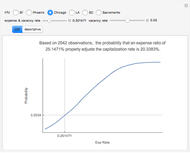
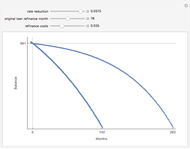
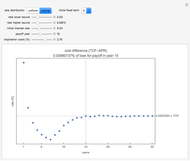
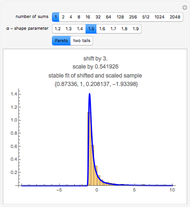
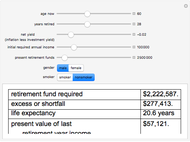
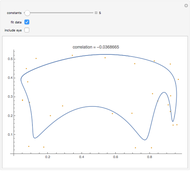
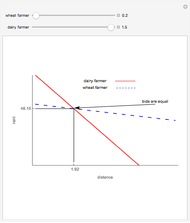
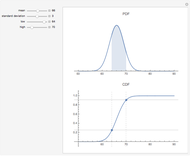
Capitalization rate (CR) is very sensitive to assumptions made about operating expenses when calculating net income. Statistical analysis of a variable, named "expense and vacancy rate" (EVR), can provide guidance. Happily, deriving EVR employs two of the most accurate characteristics in a real estate dataset: gross income and value. These two variables combine to make the gross rent multiplier (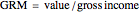 ). EVR is the result of subtracting the product of GRM and the capitalization rate from one, thus
). EVR is the result of subtracting the product of GRM and the capitalization rate from one, thus 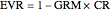 .
.
Contributed by: Roger J. Brown (June 2009)
Reproduced by permission of Academic Press from Private Real Estate Investment ©2005
Open content licensed under CC BY-NC-SA
Snapshots
Details
More information is available in chapter three of [1] and at mathestate.com.
Reference
[1] R. J. Brown, Private Real Estate Investment: Data Analysis and Decision Making, Burlington, MA: Elsevier Academic Press, 2005.
Permanent Citation
"Capitalization Rate Probability"
http://demonstrations.wolfram.com/CapitalizationRateProbability/
Wolfram Demonstrations Project
Published: June 24 2009