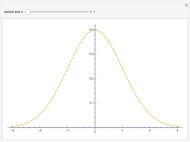
Central Limit Theorem for the Continuous Uniform Distribution

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
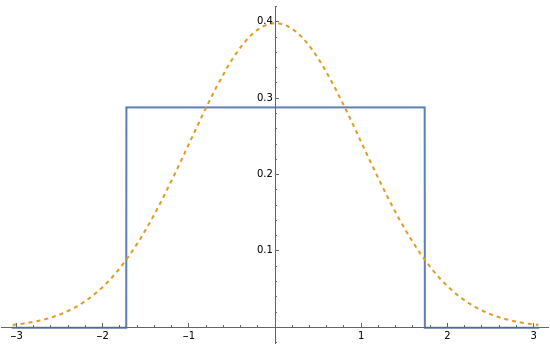
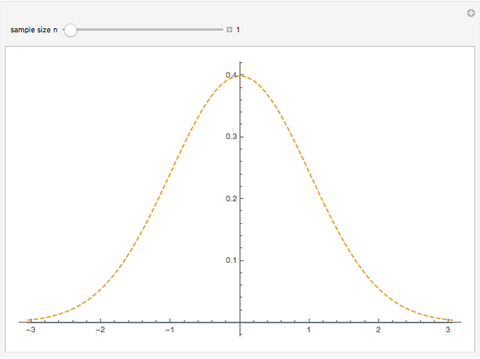
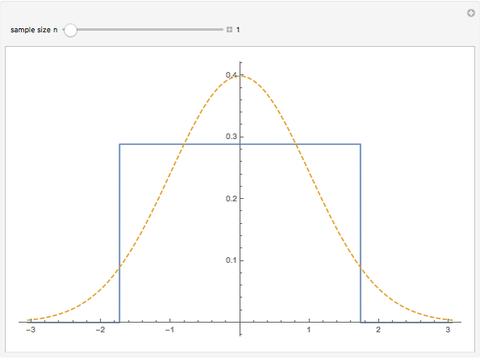
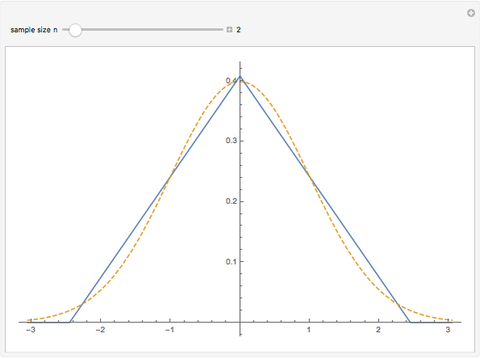
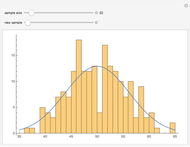
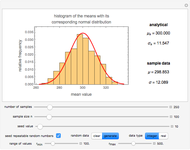
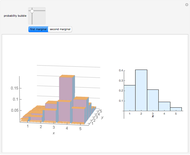
This Demonstration illustrates the central limit theorem for the continuous uniform distribution on an interval. If 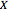 has the uniform distribution on the interval
has the uniform distribution on the interval 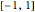 and
and 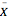 is the mean of an independent random sample of size
is the mean of an independent random sample of size  from this distribution, then the central limit theorem says that the corresponding standardized distribution
from this distribution, then the central limit theorem says that the corresponding standardized distribution 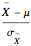 approaches the standard normal distribution as
approaches the standard normal distribution as  . Using operations on the characteristic function of
. Using operations on the characteristic function of  we can compute the PDF of
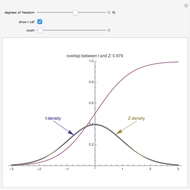
we can compute the PDF of  more easily than we could directly. The blue curve is the PDF of
more easily than we could directly. The blue curve is the PDF of 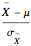 and the dashed curve is the PDF of a standard normal distribution.
and the dashed curve is the PDF of a standard normal distribution.
Contributed by: David K. Watson (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
If 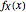 is the PDF of a random variable
is the PDF of a random variable 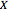 , then
, then 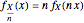 ,
, 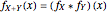 , (the convolution of
, (the convolution of 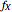 and
and 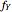 ). This means that finding the PDF of
). This means that finding the PDF of 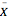 involves computing the
involves computing the  -fold convolution of
-fold convolution of 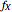 with itself, a computationally intensive operation to do directly even for small
with itself, a computationally intensive operation to do directly even for small  and simple PDF functions. This can be made easier in some cases by using the convolution property of Fourier transforms,
and simple PDF functions. This can be made easier in some cases by using the convolution property of Fourier transforms, 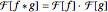 , and the observation that the characteristic function of
, and the observation that the characteristic function of 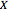 is the same as the Fourier transform
is the same as the Fourier transform 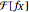 , which means that
, which means that 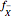 can be computed by rescaling the inverse Fourier Transform of
can be computed by rescaling the inverse Fourier Transform of 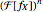 . For the uniform distribution, this lets us compute the distribution for
. For the uniform distribution, this lets us compute the distribution for 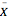 much more quickly than we could directly, though it is still a little slow for larger
much more quickly than we could directly, though it is still a little slow for larger  .
.
Permanent Citation