Closed Form Solutions for Spheroidal Functions

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
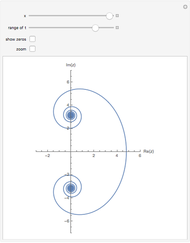
For general parameter values, the spheroidal functions  ,
,  ,
,  , and
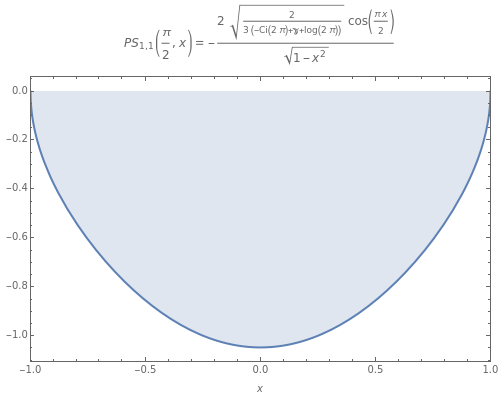
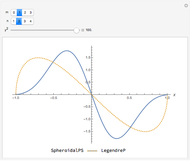
, and  are difficult to compute and are calculated via infinite series representations involving simpler special functions. However, for the special set of parameter values
are difficult to compute and are calculated via infinite series representations involving simpler special functions. However, for the special set of parameter values  ,
,  , and
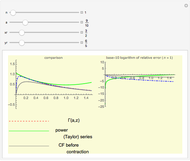
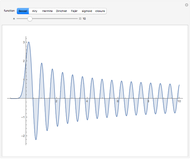
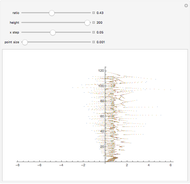
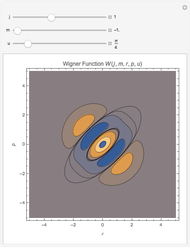
, and  , simple closedâ€Âform solutions can be found involving trigonometric functions. These provide a useful check on the accuracy of numerical solutions. In this Demonstration we illustrate these solutions by showing both the closedâ€Âform expression and plotting over the standard domain of
, simple closedâ€Âform solutions can be found involving trigonometric functions. These provide a useful check on the accuracy of numerical solutions. In this Demonstration we illustrate these solutions by showing both the closedâ€Âform expression and plotting over the standard domain of  .
.
Contributed by: Peter Falloon (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
C. Flammer, Spheroidal Wave Functions, Stanford: Stanford University Press, 1957.
P. E. Falloon, "Theory and Computation of Spheroidal Harmonics with General Arguments," master's thesis, University of Western Australia, 2001.
Permanent Citation
"Closed Form Solutions for Spheroidal Functions"
http://demonstrations.wolfram.com/ClosedFormSolutionsForSpheroidalFunctions/
Wolfram Demonstrations Project
Published: March 7 2011