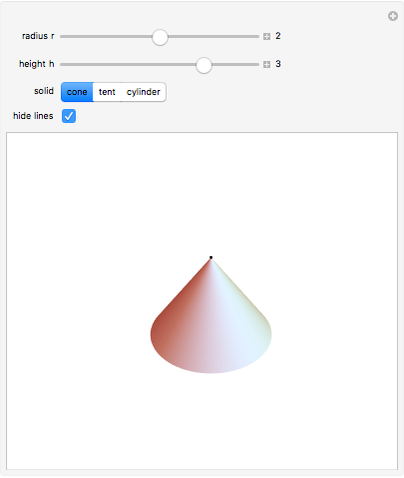
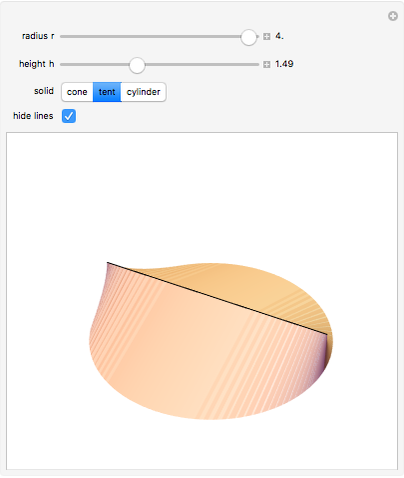
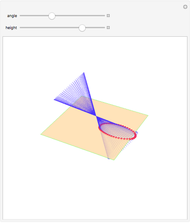
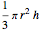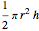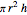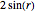Cone, Tent, and Cylinder
Initializing live version

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
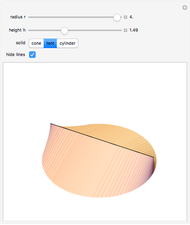
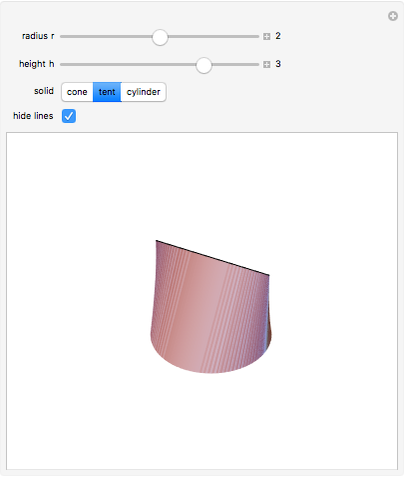
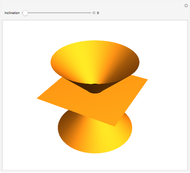
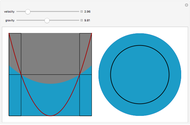
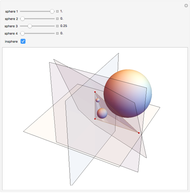
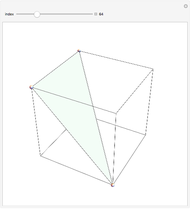
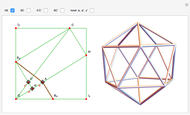
The tent is formed by joining the points along a ridge line to a circle below. The lines joining the ridge to the circle lie in the plane perpendicular to the ridge.
[more]
Contributed by: George Beck (September 2007)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Permanent Citation