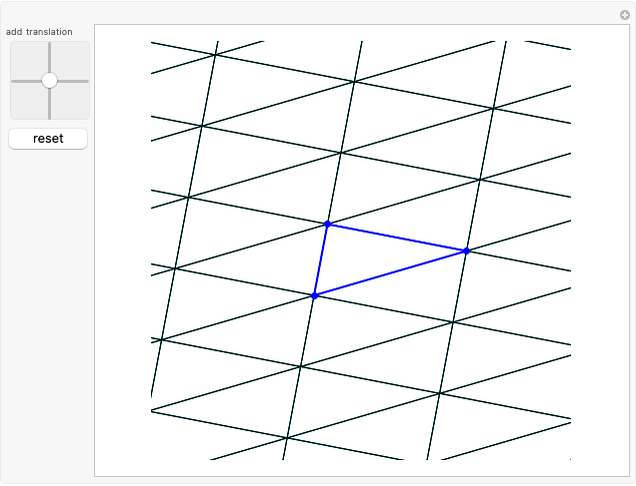
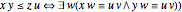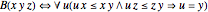Equidistance and Betweenness in Euclidean Plane Geometry

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
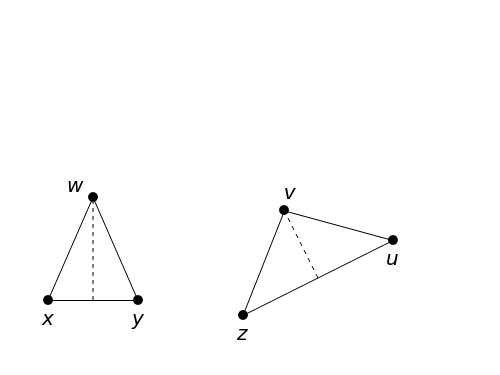
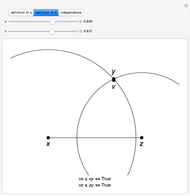
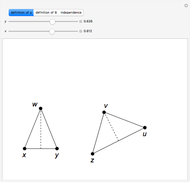
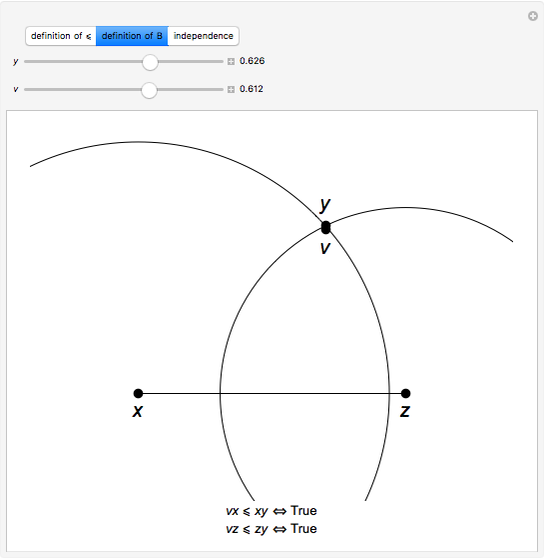
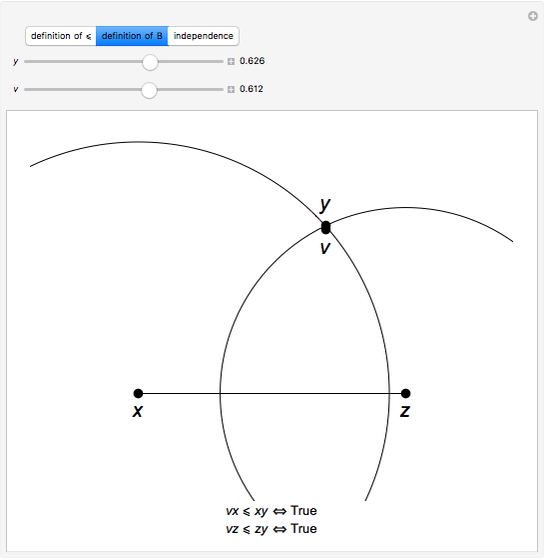
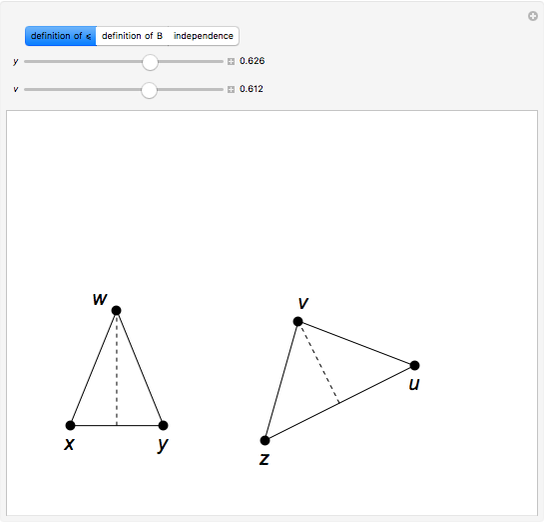
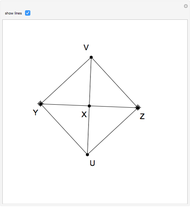
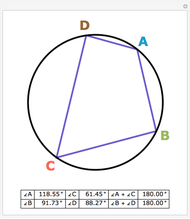
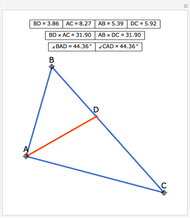
Tarski axiomatized Euclidean plane geometry in first-order logic using two primitive relations: "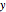 lies between
lies between  and
and  ," written as
," written as 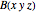 , and "
, and " is as distant from
is as distant from 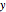 as is
as is  from
from  ," written as
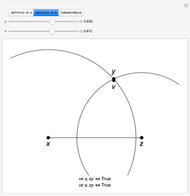
," written as  . This Demonstration shows that in plane Euclidean geometry, the relation
. This Demonstration shows that in plane Euclidean geometry, the relation  is definable using relation
is definable using relation  , but the opposite is not true.
, but the opposite is not true.
Contributed by: Izidor Hafner (April 2018)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Notation and definitions are from [2].
References
[1] A. Tarski, "What Is Elementary Geography?," The Axiomatic Method: With Special Reference to Geometry and Physics (L. Henkin, P. Suppes and A. Tarski, eds.), Amsterdam: North-Holland, 1959 pp. 16–29.
[2] A. Tarski and S. Givant, "Tarski's System of Geometry," Bulletin of Symbolic Logic, 5(2), 1999 pp. 175–214. www.math.ucla.edu/~asl/bsl/0502/0502-002.ps.
Permanent Citation