Feynman's Relativistic Electrodynamics Paradox

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
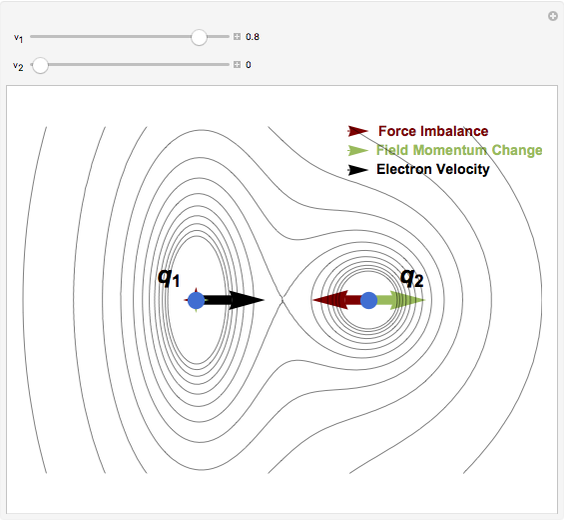
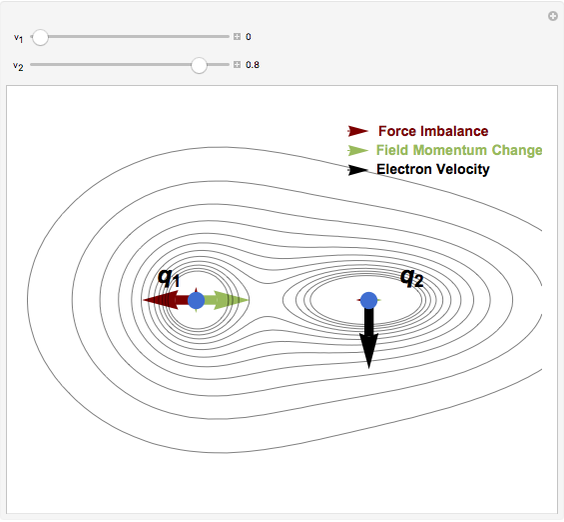
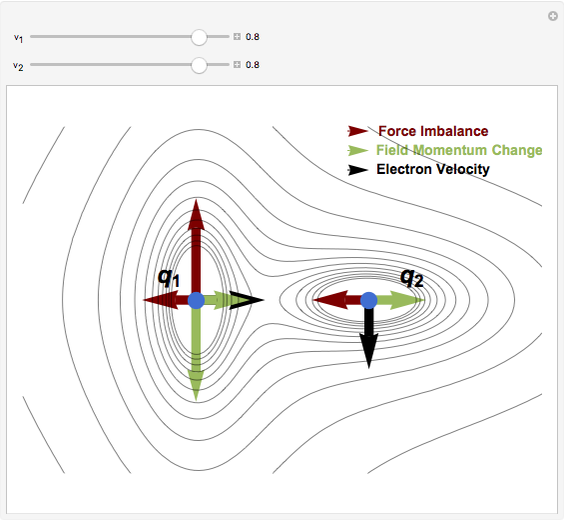
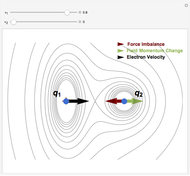
A charged particle is located on the  axis with its velocity pointing along the
axis with its velocity pointing along the  axis. Another charged particle is also located on the
axis. Another charged particle is also located on the  axis, but with its velocity pointing along the negative
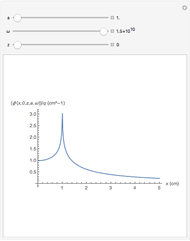
axis, but with its velocity pointing along the negative  axis. The net relativistic electric force on both particles is given by
axis. The net relativistic electric force on both particles is given by 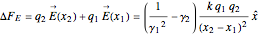 , where
, where  is the Coulomb constant,
is the Coulomb constant, 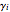 is the factor
is the factor 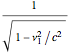 ,
, 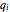 is the charge, and
is the charge, and 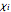 is the position of the
is the position of the 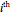 particle. 
The relativistic magnetic force of particle 2 on 1 is
particle. 
The relativistic magnetic force of particle 2 on 1 is 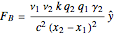 . The magnetic force of particle 1 on 2 is zero. The momentum of the electromagnetic field is given by the time derivative of the vector potential
. The magnetic force of particle 1 on 2 is zero. The momentum of the electromagnetic field is given by the time derivative of the vector potential 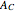 in the Coulomb gauge,
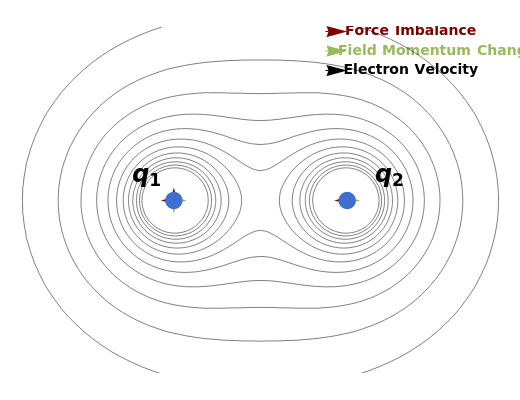
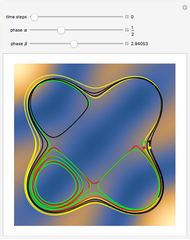
in the Coulomb gauge, 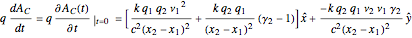 . The sum of all three terms is zero and shows momentum conservation. The contour lines show the relativistic Liénard–Wiechert potential for each charge.
. The sum of all three terms is zero and shows momentum conservation. The contour lines show the relativistic Liénard–Wiechert potential for each charge.
Contributed by: Adam Caprez and Herman Batelaan (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
For more information see A. Caprez and H. Batelaan, "Feynman's Relativistic Electrodynamics Paradox and the Aharonov-Bohm Effect," Foundations of Physics 39(3), 2009 pp. 295–306.
Permanent Citation
"Feynman's Relativistic Electrodynamics Paradox"
http://demonstrations.wolfram.com/FeynmansRelativisticElectrodynamicsParadox/
Wolfram Demonstrations Project
Published: March 7 2011