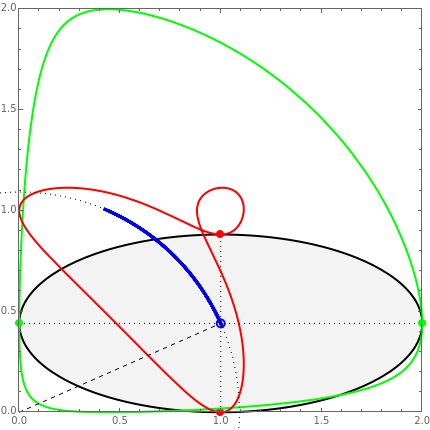
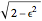Glissettes and the Orthoptic Curve of the Ellipse
Initializing live version

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
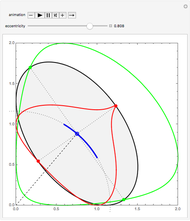
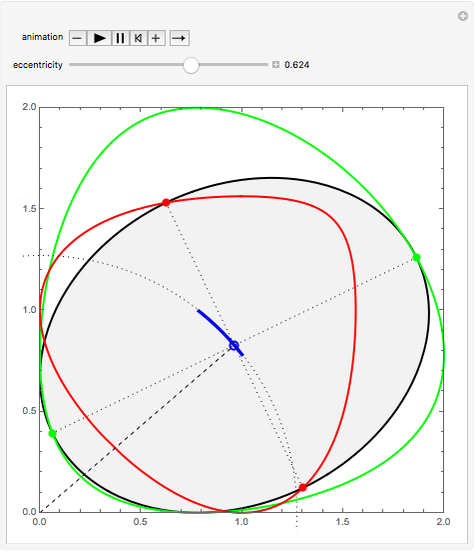
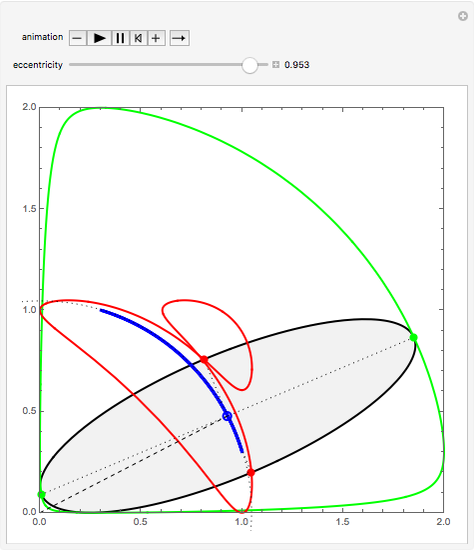
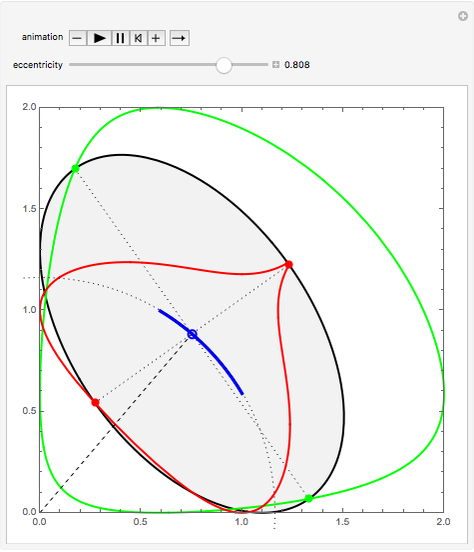
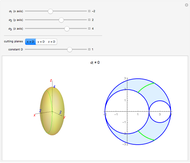
A glissette is the geometrical locus of a point fixed to a curve sliding inside another curve. This Demonstration shows three different glissettes that are formed by points of an ellipse sliding inside a pair of orthogonal lines (the  and
and 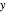 axes).
axes).
Contributed by: Erik Mahieu (February 2012)
Open content licensed under CC BY-NC-SA
Snapshots
Details
An interesting collection of glissettes can be found in [1].
Reference
[1] R. Ferréol. "Encyclopédie des Formes Mathématiques Remarquables." Mathcurve. www.mathcurve.com.
Permanent Citation