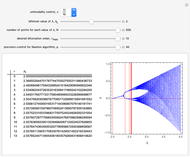
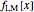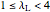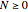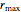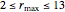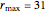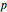High-Precision Newton Algorithm for Generalized Logistic Maps with Unimodality z

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
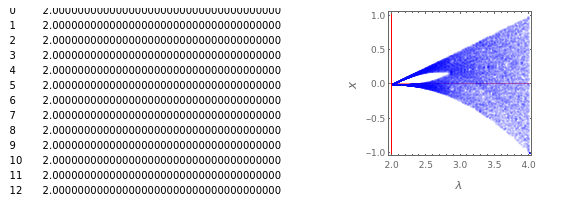
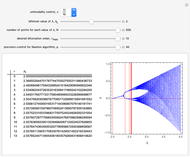
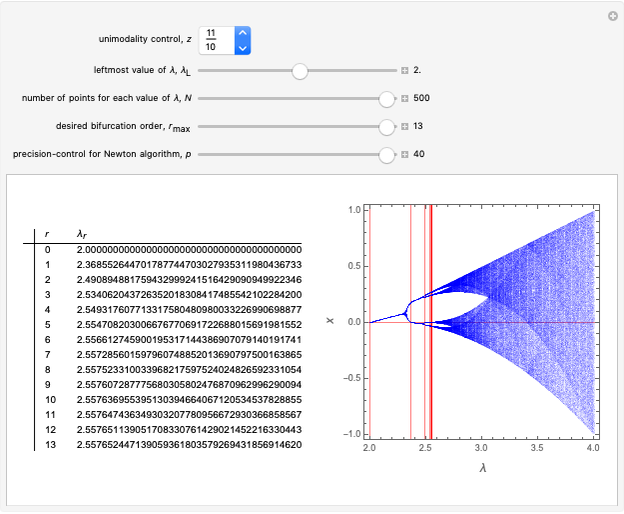
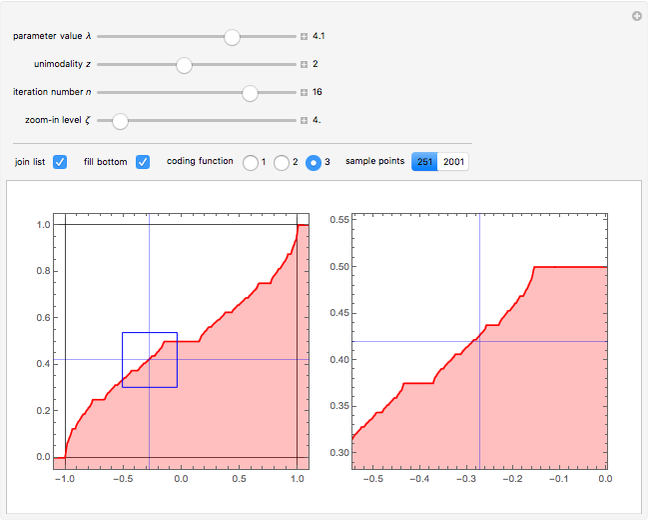
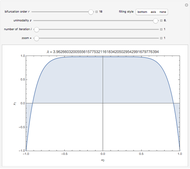
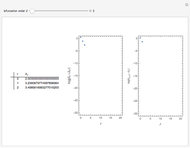
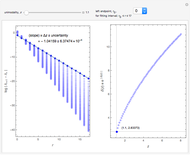
This Demonstration shows a table of superstable parameter values of a period-doubling periodic attractor. The test map is defined as 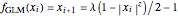 , which generalizes the well-known logistic map
, which generalizes the well-known logistic map 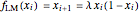 . Here
. Here  is an iteration number,
is an iteration number,  , and
, and  is the unimodality (or the degree) of the local maximum of
is the unimodality (or the degree) of the local maximum of 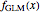 . The superstable parameter values are used for the renormalization group analysis of many low-dimensional dynamical systems with chaotic behavior. See the references [1–4].
. The superstable parameter values are used for the renormalization group analysis of many low-dimensional dynamical systems with chaotic behavior. See the references [1–4].
Contributed by: Ki-Jung Moon (November 2013)
Open content licensed under CC BY-NC-SA
Snapshots
Details
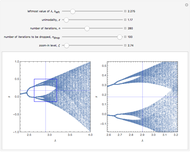
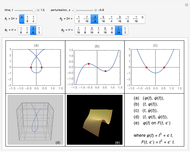
Snapshot 1 for 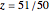 ; snapshot 2 for
; snapshot 2 for 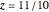 ; snapshot 3 for
; snapshot 3 for 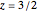 ; snapshot 4 for
; snapshot 4 for  ; snapshot 5 for
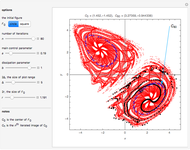
; snapshot 5 for  . The generalized logistic map is particularly convenient for renormalization group analysis because:
. The generalized logistic map is particularly convenient for renormalization group analysis because:
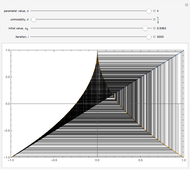
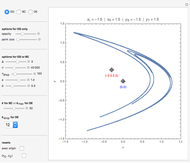
1. The attracting domain (or the basin of attraction) of any periodic/chaotic attractor is strictly confined within 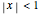 for any
for any 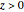 and
and 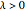 .
.
2. All boundary crises occur at  for any
for any 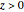 .
.
Mitchell J. Feigenbaum's original renormalization group analysis on the classic logistic map can be found in [1] and [2]. More information on the renormalization group analysis can be found in [3] and [4].
References
[1] M. J. Feigenbaum, "Quantitative Universality for a Class of Non-Linear Transformations," Journal of Statistical Physics, 19, 1978 pp. 25–52.
[2] M. J. Feigenbaum, "The Universal Metric Properties of Nonlinear Transformations," Journal of Statistical Physics, 21, 1979 pp. 669–706.
[3] K.-J. Moon, "Reducible Expansions and Related Sharp Crossovers in Feigenbaum's Renormalization Field," Chaos: An Interdisciplinary Journal of Nonlinear Science, 18, 2008 pp. 023104.
[4] K.-J. Moon, "Erratum: Reducible Expansions and Related Sharp Crossovers in Feigenbaum's Renormalization Field," Chaos: An Interdisciplinary Journal of Nonlinear Science, 20, 2010 pp. 049902.
Permanent Citation