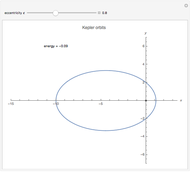
Kepler Orbits
Initializing live version

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
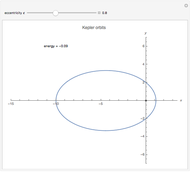
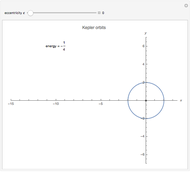
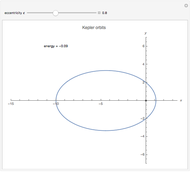
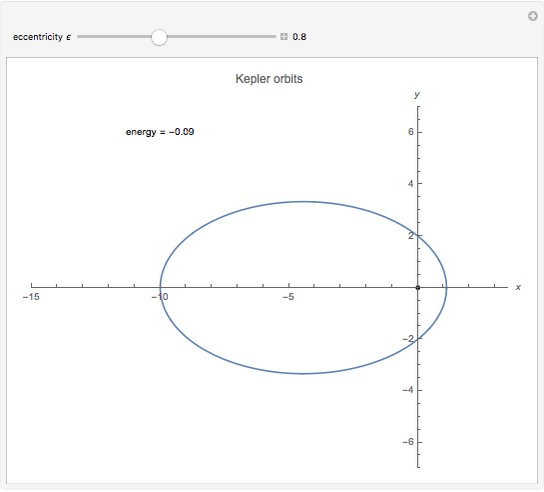
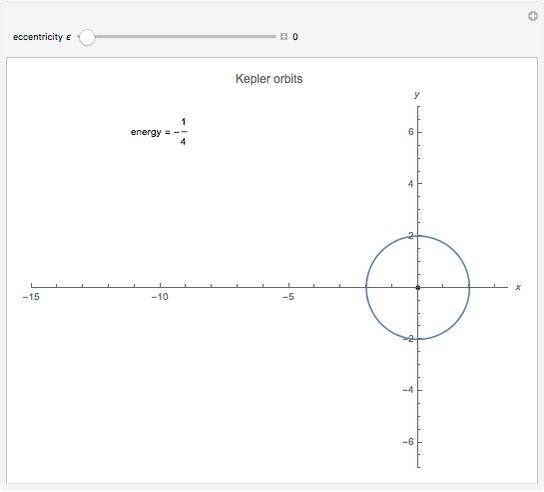
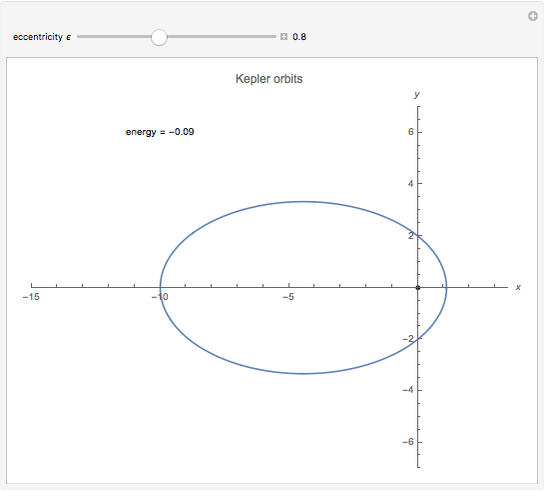
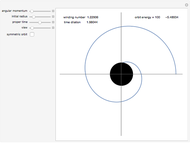
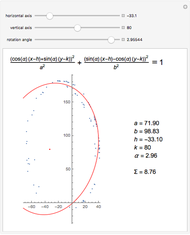
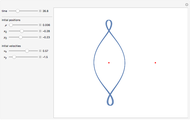
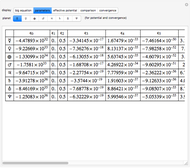
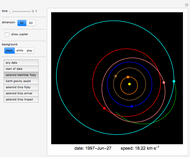
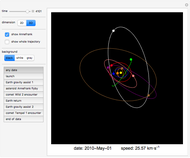
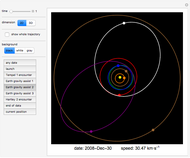
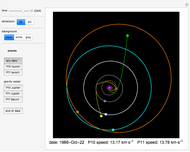
The Kepler orbits for a two-body system under a central gravitational force can be represented by a polar curve that relates the distance between the two bodies with the angle from the  axis. The shape of the orbit depends on its eccentricity, which is determined by the energy of the system.
axis. The shape of the orbit depends on its eccentricity, which is determined by the energy of the system.
Contributed by: Sarbani Chatterjee and Radhika Prasad (August 2018)
Supervised by: Nisha Rani
(Miranda House, University of Delhi)
Open content licensed under CC BY-NC-SA
Details
$FailedSnapshots
Permanent Citation