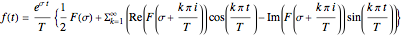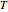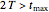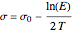Wolfram Demonstrations Project

13,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

Numerical Inversion of the Laplace Transform: The Fourier Series Approximation

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

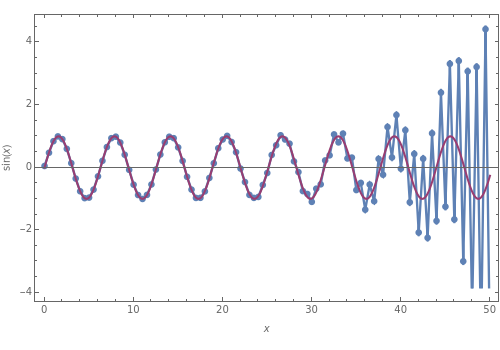
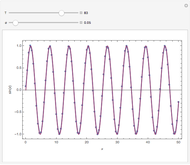
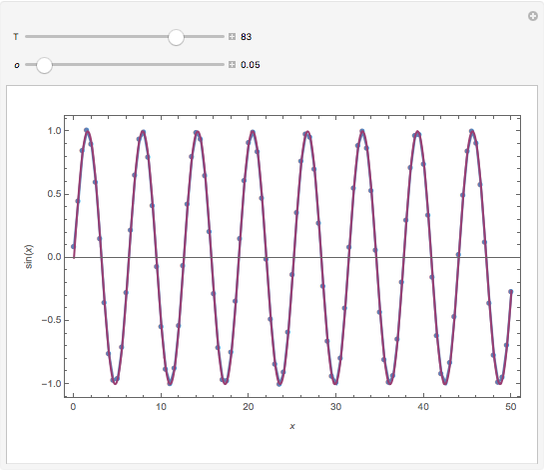
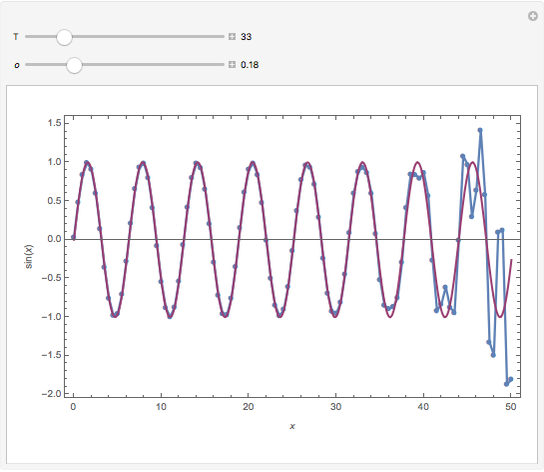
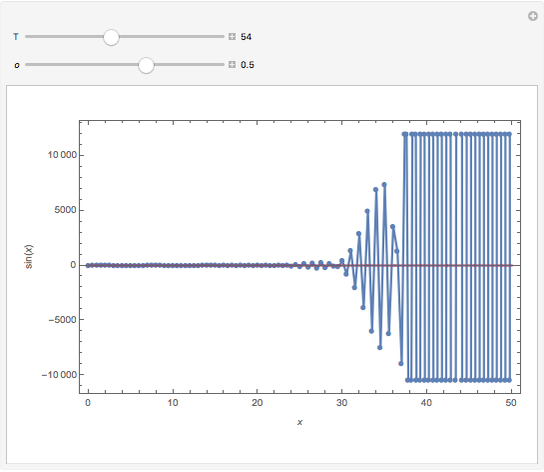
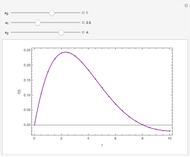
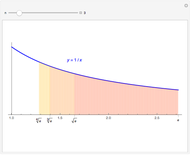
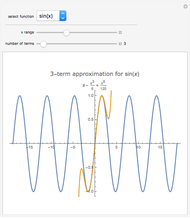
This Demonstration shows how you can numerically compute the inverse of the Laplace transform 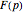of a simple function 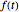: 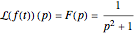and 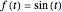. The selected method is the Fourier series approximation. This method uses the following formula in order to perform the inversion of :

[more]

.

You can select the appropriate values of and that give the correct inverse. This choice must be such that and , where is a measure of the maximum relative error and is the exponential order of .

The red curve is the sine function and the blue dots are the selected numerical values of the inverse of .

You can clearly see how this method may fail to give an accurate inverse if the values of and are not correctly selected. The first snapshot presents a correct inversion result. The next two snapshots show situations where the method gives erroneous data.

[less]

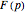Contributed by: Housam Binous (March 2011)
Open content licensed under CC BY-NC-SA

Snapshots

Details

R. G. Rice and D. D. Do, Applied Mathematics and Modeling for Chemical Engineers, New York: Wiley, 1995.

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send