Phenomenological Approximation to Newton's Cradle

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
"Newton's cradle" (never actually mentioned by Newton himself) is an iconic executive desk toy consisting of five identical polished steel balls aligned with one another, each suspended from a frame a by pair of thin wires. When a ball at one end of the line is pulled back and released, it collides with the middle three balls, which remain stationary, while the ball at the other end of the line swings out to mirror the motion of the first ball. Then the motion reverses itself and repeats through a significant number of cycles. The canonical explanation of this phenomenon, commonly shown in many high school and college physics classes, is simply the conservation of momentum 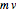 and kinetic energy
and kinetic energy 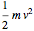 in elastic collisions, these quantities being exchanged between the first and fifth balls through infinitesimal elastic deformations, similar to sound waves. Sometimes Newton's third law of action and reaction is evoked in the explanation.
in elastic collisions, these quantities being exchanged between the first and fifth balls through infinitesimal elastic deformations, similar to sound waves. Sometimes Newton's third law of action and reaction is evoked in the explanation.
Contributed by: S. M. Blinder (June 2011)
After a Demonstration byEnrique Zeleny and detailed critiques by Giovanni Ciriani and Itai Saggev.
Open content licensed under CC BY-NC-SA
Snapshots
Details
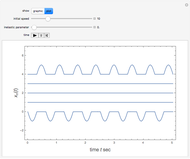
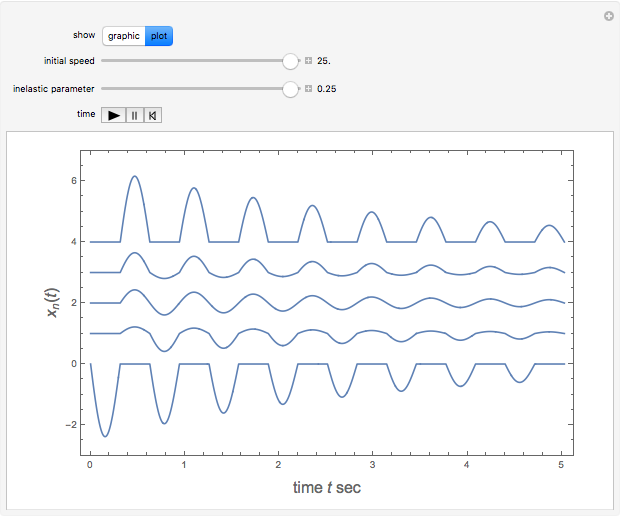
Left-hand ball behaves initially as a simple pendulum, well approximated by the linearized equation 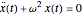 , with
, with 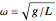 and initial condition
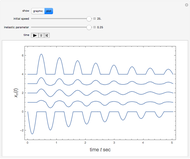
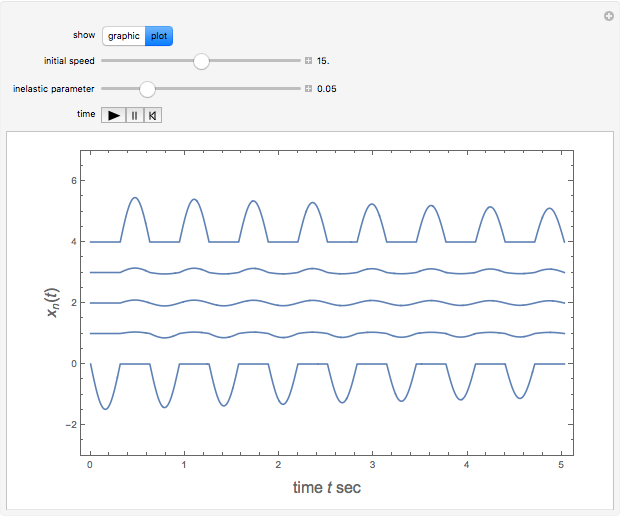
and initial condition 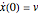 , the speed at which the first ball hits the line of four others. The kinetic energy is partially converted into compression energy, which propagates the impulse along the chain. The function
, the speed at which the first ball hits the line of four others. The kinetic energy is partially converted into compression energy, which propagates the impulse along the chain. The function 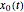 approximates a modulated square wave, in which only negative values of
approximates a modulated square wave, in which only negative values of 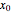 appear. The fifth ball closely follows a complementary positive square-wave sinusoidal motion bounded by
appear. The fifth ball closely follows a complementary positive square-wave sinusoidal motion bounded by 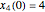 . The approximate functional forms for the
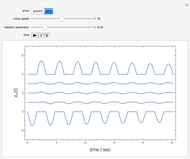
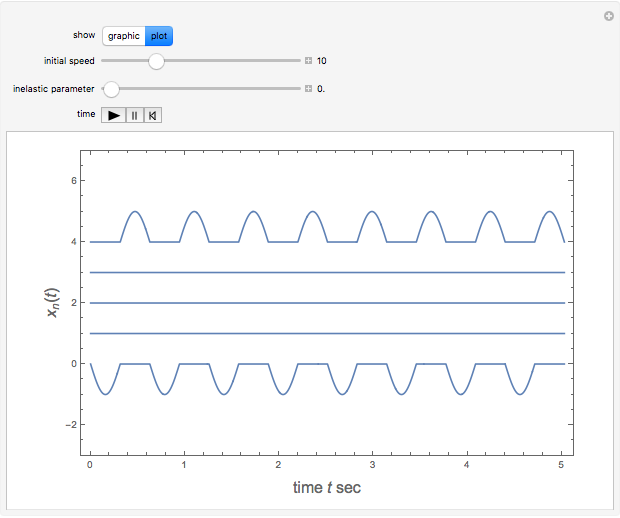
. The approximate functional forms for the  are plotted as functions of time. Snapshot 1 shows the canonical case with
are plotted as functions of time. Snapshot 1 shows the canonical case with 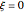 .
.
References
[1] S. Hutzler, G. Delaney, D. Weaire, and F. MacLeod, "Rocking Newton's Cradle," American Journal of Physics, 72(12), 2004 pp. 1508–1516.
[2] C. M. Donahue, C. M. Hrenya, A. P. Zelinskaya, and K. J. Nakagawa, "Newton's Cradle Undone: Experiments and Collision Models for the Normal Collision of Three Solid Spheres," Physics of Fluids, 20(11), 2008, 113301.
[3] C. F. Gauld, "Newton's Cradle in Physics Education," Science & Education, 15(8), 2006 pp. 597–617.
[4] E. J. Hinch and S. Saint–Jean, "The Fragmentation of a Line of Balls by an Impact," in Proceedings of the Royal Society of London, Series A: Mathematical, Physical and Engineering Sciences, 455(1989), 1999 pp. 3201–3220.
[5] V. Ceanga and Y. Hurmuzlu, "A New Look to an Old Problem: Newton’s Cradle," Journal of Applied Mechanics, 68(4), 2001 pp. 575–583.
[6] F. Hermann and M. Seitz, "How Does the Ball and Chain Work?" American Journal of Physics, 50(11), 1981 pp. 977–981.
Permanent Citation