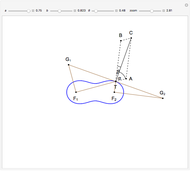
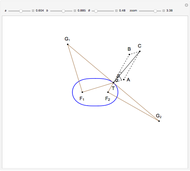
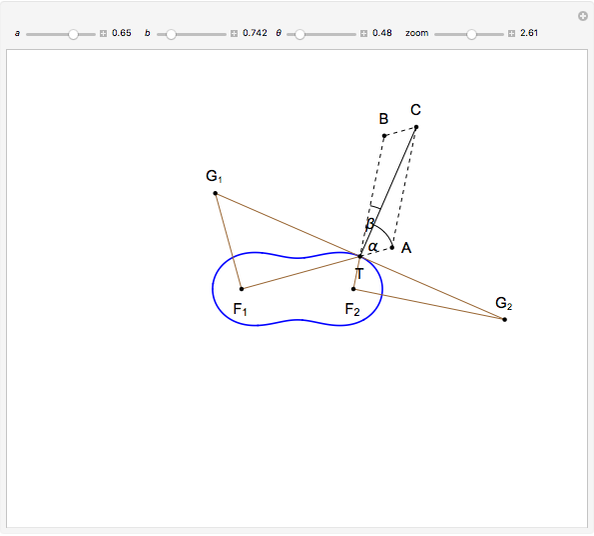
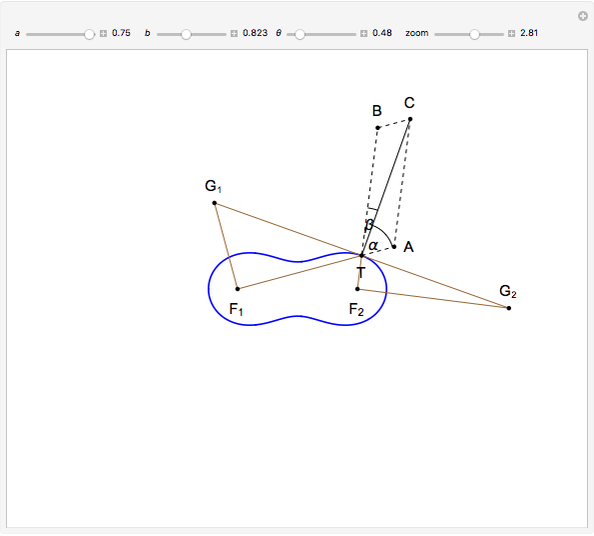
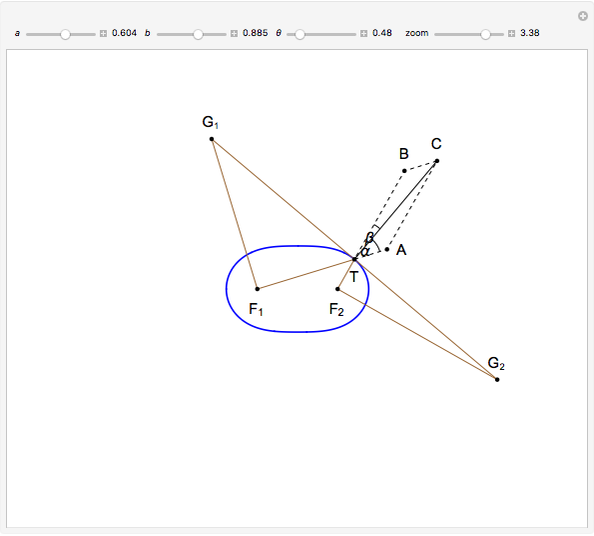
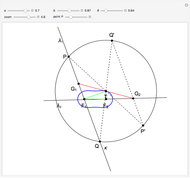
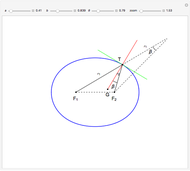
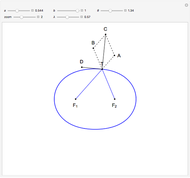
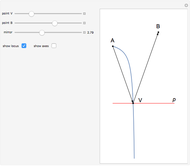
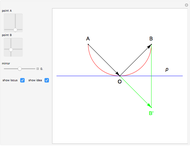
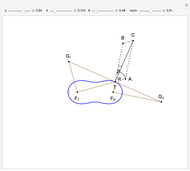
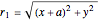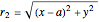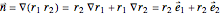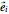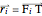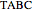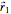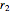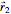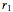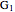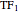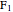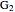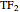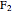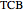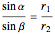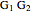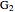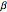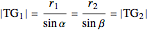Steiner Formula for Cassini Oval
Initializing live version

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
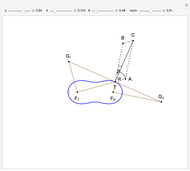
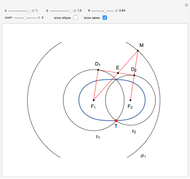
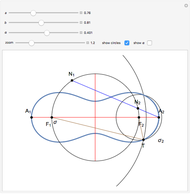
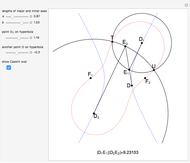
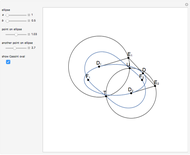
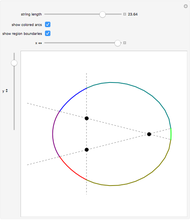
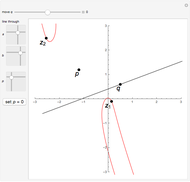
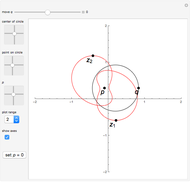
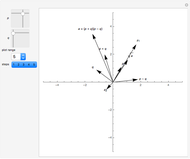
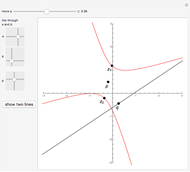
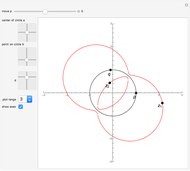
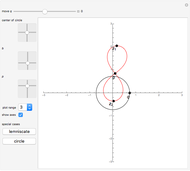
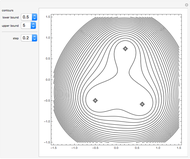
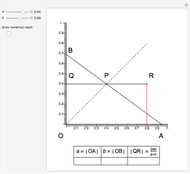
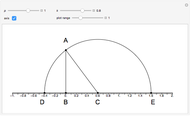
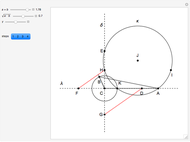
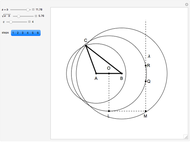
A Cassini oval is the locus of points 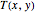 such that
such that 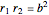 , where
, where 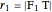 and
and 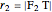 . If the foci
. If the foci 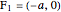 and
and 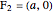 , then
, then
Contributed by: Marko Razpet and Izidor Hafner (August 2018)
Open content licensed under CC BY-NC-SA
Details
Reference
[1] A. Ostermann and G. Wanner, Geometry by Its History, New York: Springer, 2012.
Snapshots
Permanent Citation