Wolfram Demonstrations Project

13,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

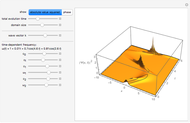
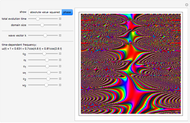
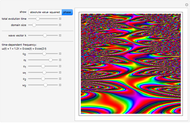
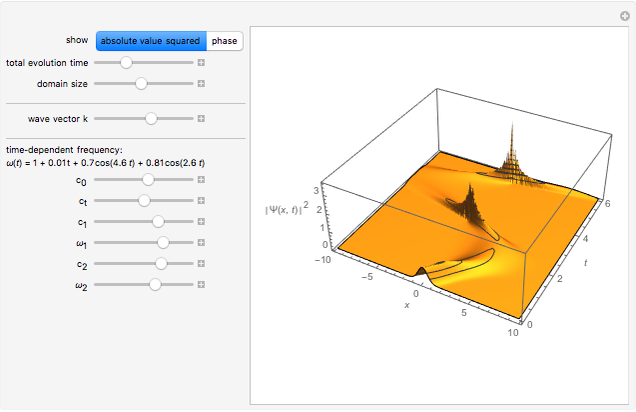
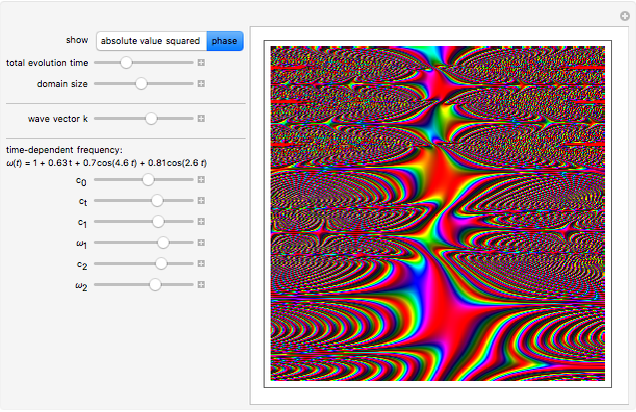
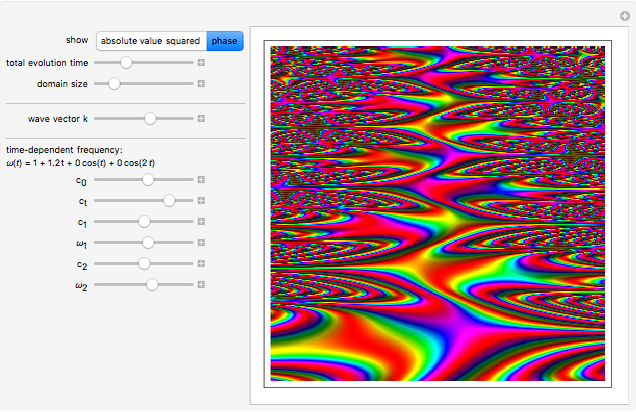
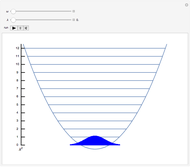
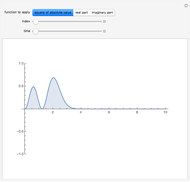
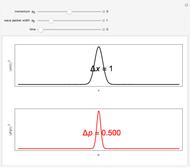
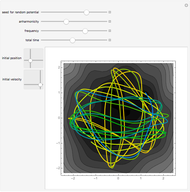
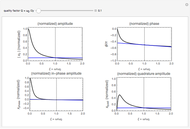
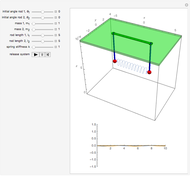
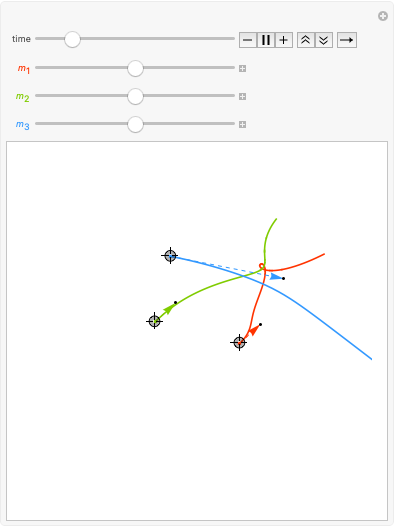
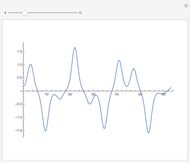
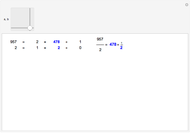
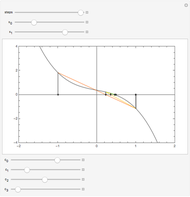
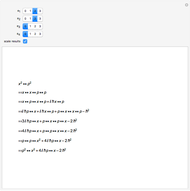
Time Evolution of Quantum-Mechanical Harmonic Oscillator with Time-Dependent Frequency

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

$Failed

Contributed by: Michael Trott with permission of Springer. (March 2011)
From: The Mathematica GuideBook for Numerics, second edition by Michael Trott (© Springer, 2008).
Open content licensed under CC BY-NC-SA

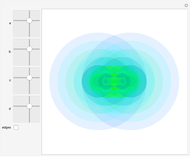
Snapshots

Details

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send