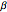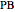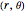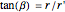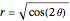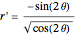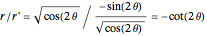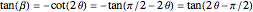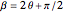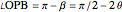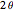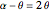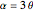Trisecting an Angle Using a Lemniscate
Initializing live version

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
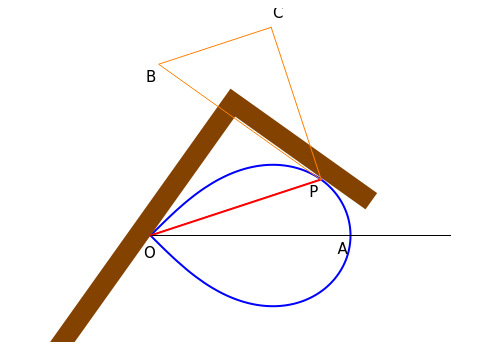
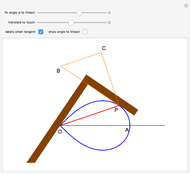
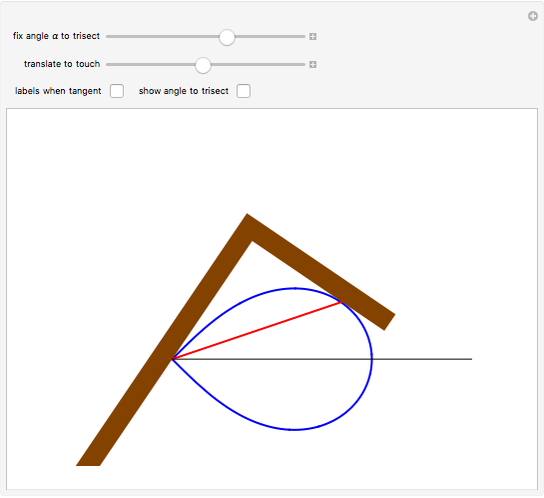
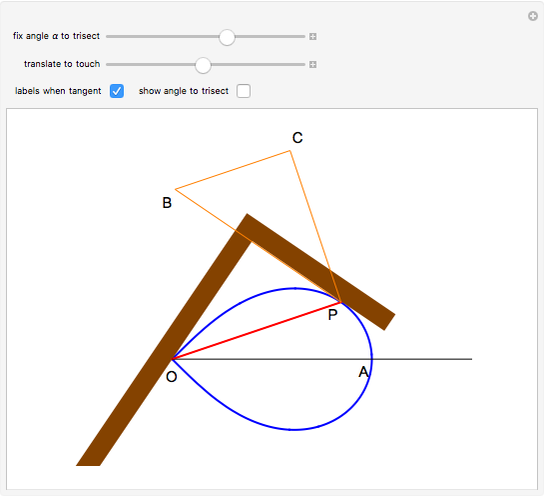
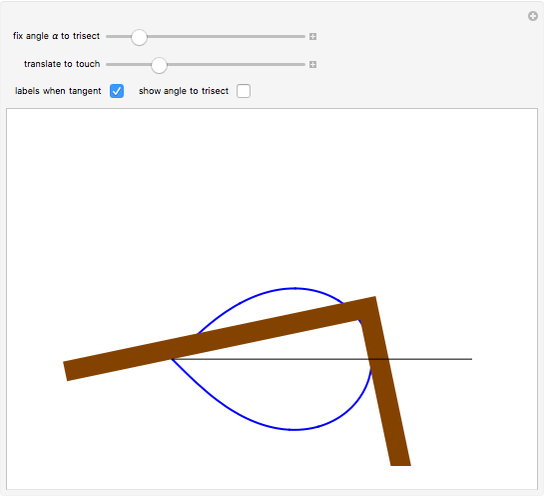
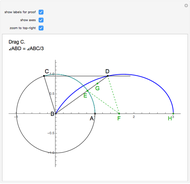
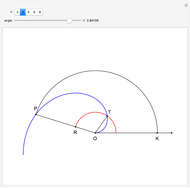
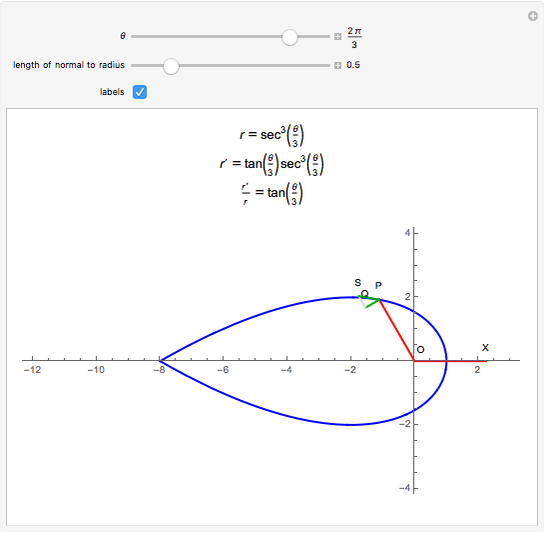
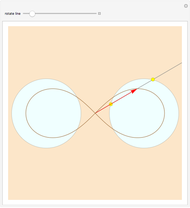
The angle to trisect, say  , is between the longer leg of the carpenter's square (the brown "L" shape) and the polar axis
, is between the longer leg of the carpenter's square (the brown "L" shape) and the polar axis  . Translate the carpenter's square so that it touches the curve at the point
. Translate the carpenter's square so that it touches the curve at the point 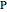 . The angle
. The angle 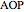 between the radius vector
between the radius vector 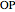 and the polar axis
and the polar axis 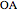 is one-third of the given angle
is one-third of the given angle  .
.
Contributed by: Izidor Hafner (February 2013)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Permanent Citation