A Bitter Chocolate Problem That Satisfies an Inequality

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
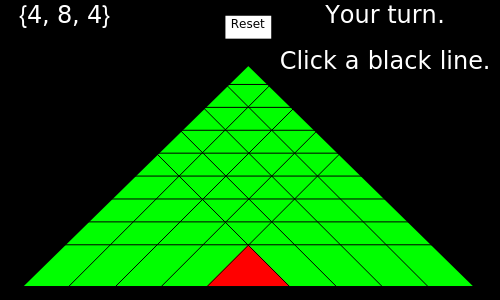
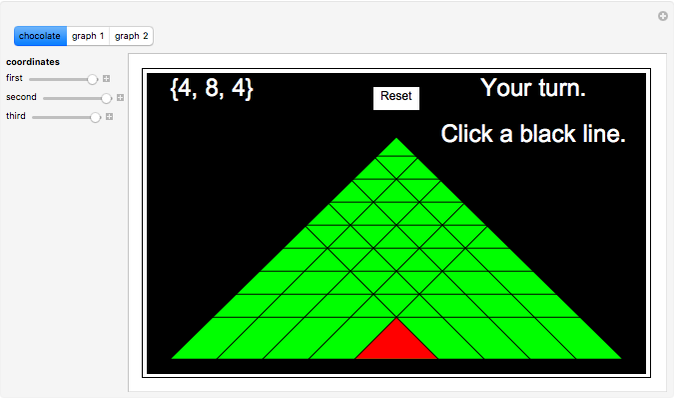
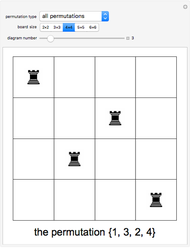
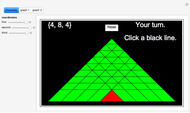
In a piece of chocolate the green parts are sweet and the red part is very bitter. This game is played by two players taking turns. Each player breaks the chocolate (in a straight line along the grooves) and eats the piece broken off. The player to leave the opponent with the single bitter part is the winner.
Contributed by: Taishi Inoue, Takuma Nakaoka, and Ryohei Miyadera (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
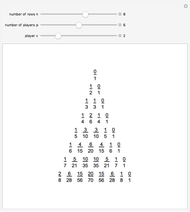
You can cut the chocolate in three ways: (1) from the upper right to the lower left, (2) horizontally, or (3) from the upper left to the lower right. Therefore it is appropriate to represent the game state with three non-negative integers  , which are shown in the upper-left corner. It is easy to see that the coordinates satisfy the inequality
, which are shown in the upper-left corner. It is easy to see that the coordinates satisfy the inequality  .
.
We define two important states: (a)  states, from which we can force a win, as long as we play correctly at every stage, and (b)
states, from which we can force a win, as long as we play correctly at every stage, and (b)  states, from which we will lose however well we play if our opponent plays correctly.
states, from which we will lose however well we play if our opponent plays correctly.
One of the most important tasks of chocolate games is to find all the  and
and  states of a game. Usually the number of
states of a game. Usually the number of  states is small.
states is small.
In this chocolate game it is easy to find  states, since there are formulas for them:
1. The position
states, since there are formulas for them:
1. The position  is an
is an  state if and only if
state if and only if  .
2. The position
.
2. The position  is an
is an  state if and only if
state if and only if  , where
, where  is the bitwise exclusive or operation (the same as BitXor in Mathematica).
is the bitwise exclusive or operation (the same as BitXor in Mathematica).
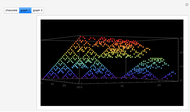
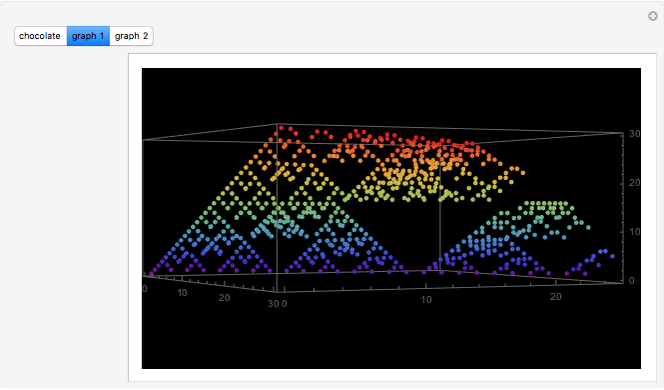
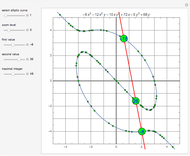
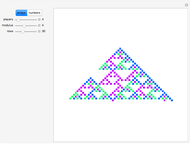
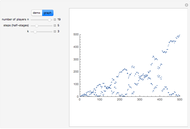
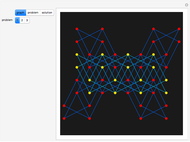
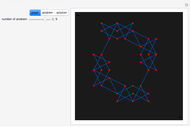
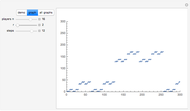
The list of  states makes a beautiful 3D graph. See "graph 1" in the Demonstration. If you rotate the graph around the
states makes a beautiful 3D graph. See "graph 1" in the Demonstration. If you rotate the graph around the  axis by
axis by  and project the list onto the plane made by the
and project the list onto the plane made by the  axis and the
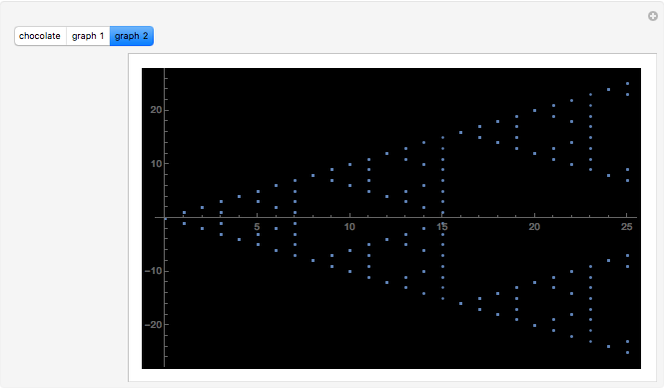
axis and the  axis, then you get a Sierpinski gasket; see "graph 2".
axis, then you get a Sierpinski gasket; see "graph 2".
Once we know the formula for  states, the strategy to win is clear. Suppose that you have a
states, the strategy to win is clear. Suppose that you have a  state; you can choose a move that leads to an
state; you can choose a move that leads to an  state. After that any move by your opponent leads to a
state. After that any move by your opponent leads to a  state, and you can always move to an
state, and you can always move to an  state. Finally you reach
state. Finally you reach  .
.
If you have to play with an  state, you can only win if your opponent makes a mistake.
state, you can only win if your opponent makes a mistake.
See [1, 2] by the authors for a proof of the formula.
References
[1] M. Naito, T. Yamauchi, H. Matsui, T. Inoue, Y. Tomari, K. Nishimura, T. Nakaoka, D. Minematsu, and R. Miyadera, "Combinatorial Games and Beautiful Graphs Produced by Them," Visual Mathematics, 11(3), 2009. http://vismath.tripod.com/pap.htm# n114.
[2] T. Yamauchi, T. Inoue, and Y. Tomari, "Variants of the Game of Nim That Have Inequalities as Conditions," Rose–Hulman Undergraduate Mathematics Journal, 10(2), 2009. http://www.rose-hulman.edu/mathjournal/v10n2.php.
Permanent Citation