Adiabatic Gas Expansion between Two Tanks

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
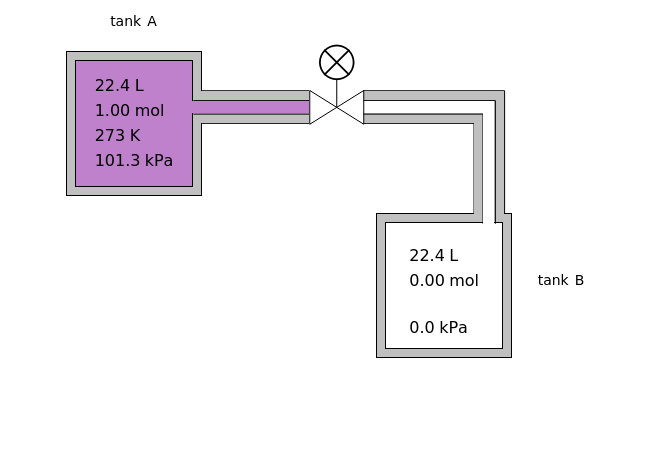
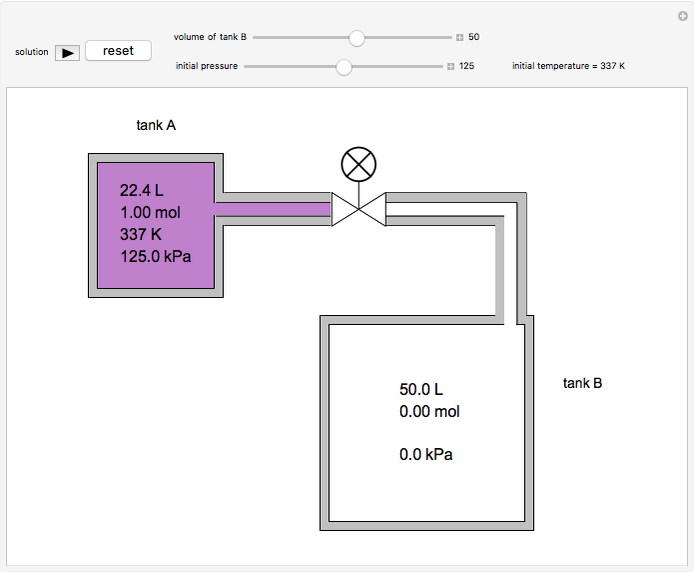
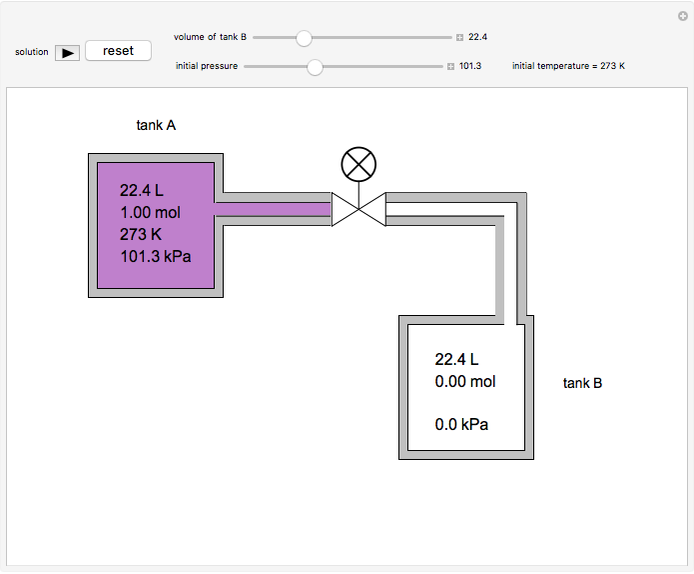
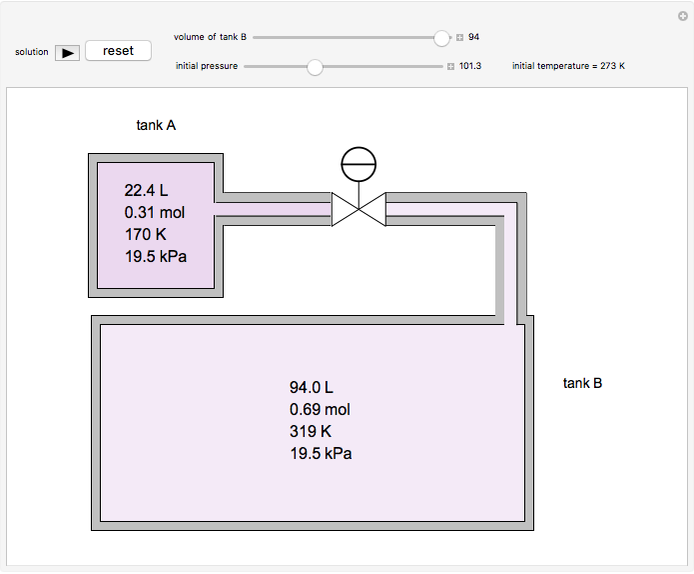
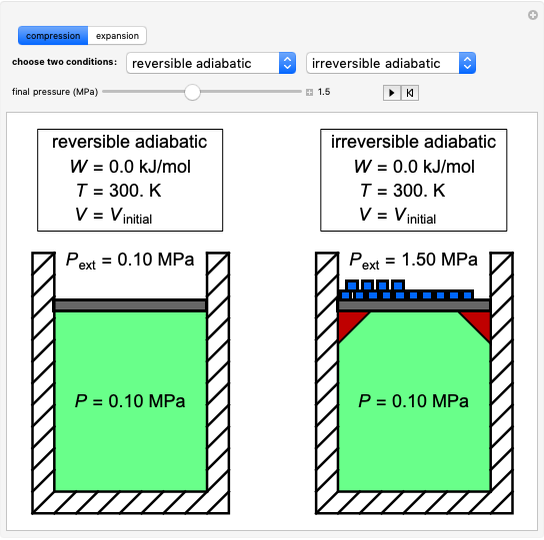
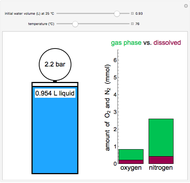
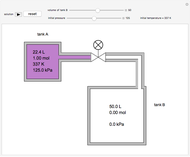
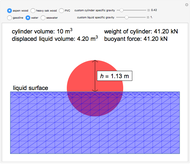
In this Demonstration, one mole of an ideal gas expands when a valve is partly opened (by selecting the play button), from tank A (top) into tank B (bottom), which is initially at vacuum. Both tanks are well insulated. You can change the volume of tank B and the initial pressure of the gas with sliders. This also changes the initial temperature since the volume of tank A and the total number of moles do not change in the process. When the pressure in the two vessels is equal, the valve closes. The expansion of the gas that remains in tank A is modeled as an adiabatic, reversible expansion. Thus, this fraction of the gas does work in pushing the rest of the gas through the valve.
Contributed by: Neil Hendren (March 25)
Additional contributions by: John L. Falconer
(University of Colorado Boulder, Department of Chemical and Biological Engineering)
Open content licensed under CC BY-NC-SA
Details
The first law of thermodynamics relates internal energy 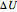 to heat added
to heat added 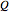 and work added
and work added 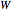 :
:
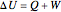 . (1)
. (1)
For an adiabatic system, heat transfer is zero:
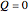 . (2)
. (2)
By the first law, applied to both tanks together, no work is done across the system boundary (the walls of the tanks):
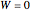 . (3)
. (3)
Therefore:
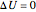 , (4)
, (4)
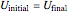 . (5)
. (5)
For an ideal gas:
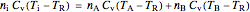 , (6)
, (6)
Equation (6) simplifies to
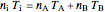 . (7)
. (7)
Equation (7) means the average temperature of the system (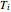 ) does not change, so the final pressure (
) does not change, so the final pressure ( ) is determined from the ideal gas law:
) is determined from the ideal gas law:
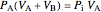 ,
,
where the initial volume of tank A is 22.4 L.
The total moles in the system does not change:
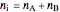 . (8)
. (8)
The expansion of the gas that remains in tank A is modeled as a reversible adiabatic expansion. This gas does work in pushing the rest of the gas out of tank A and through the valve. For an ideal gas, the final temperature in tank A is related to its initial temperature 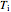 and pressure
and pressure 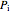 and its final pressure
and its final pressure 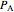 by:
by:
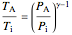 , (9)
, (9)
where 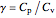 , the heat capacity ratio.
, the heat capacity ratio.
Snapshots
Permanent Citation