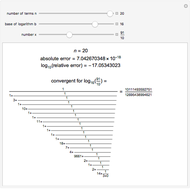
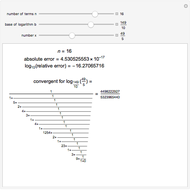
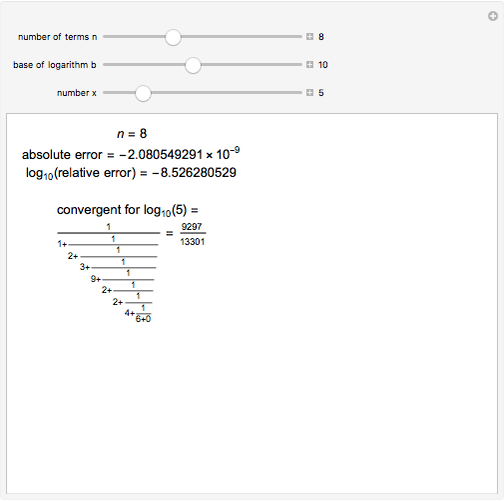
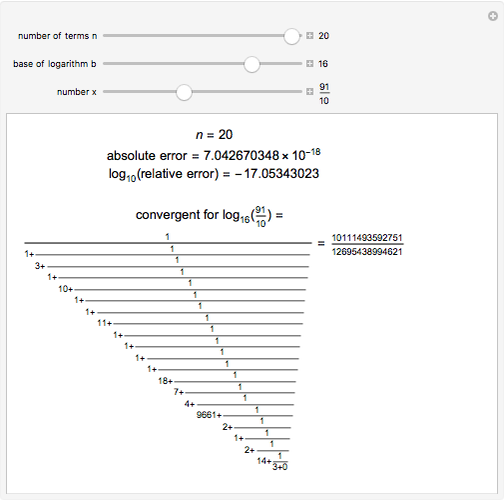
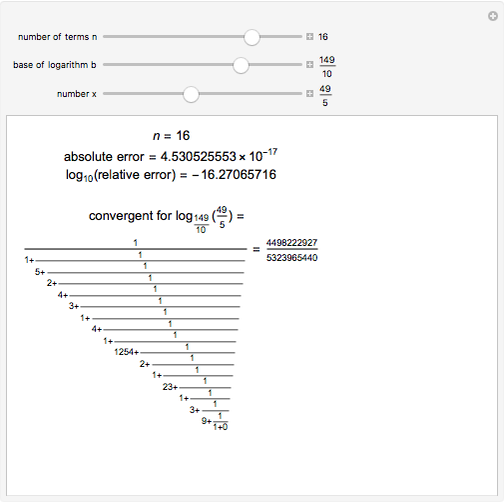
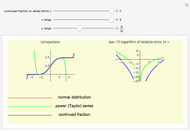
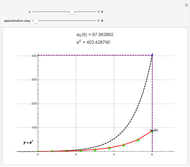
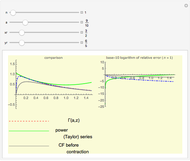
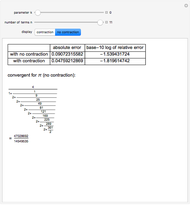
Continued fractions provide a very effective toolset for approximating functions. Usually the continued fraction expansion of a function approximates the function better than its Taylor or Fourier series. This Demonstration shows the high quality of a continued fraction expansion to approximate the logarithm to an arbitrary real base greater than 1. It uses the Shanks method and is very efficient due to its adaptability for high-speed numerical computer code.