Compound Interest Table

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
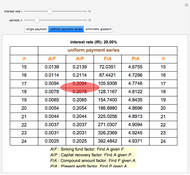
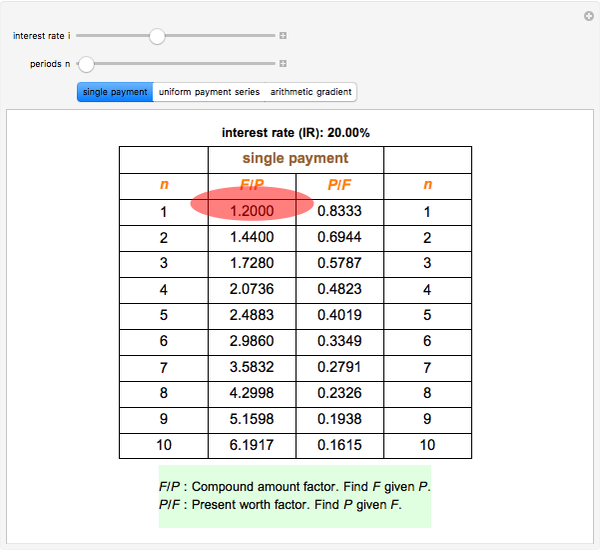
These kinds of tables for looking up compound interest for various factors are very commonly used in engineering economics.
[more]
Contributed by: Vivek J Joshi (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
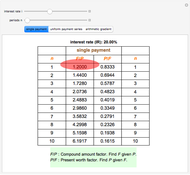
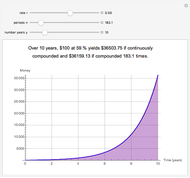
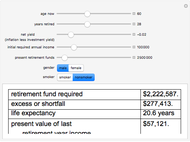
A single payment is a one-time investment of  compounded at interest rate
compounded at interest rate  for
for  periods that will reach a value
periods that will reach a value  . Or you can use the inverse, a discount factor, expressing the portion
. Or you can use the inverse, a discount factor, expressing the portion  of a desired final value
of a desired final value  that must be invested to grow to
that must be invested to grow to  in
in  periods when invested at
periods when invested at  percent.
percent.
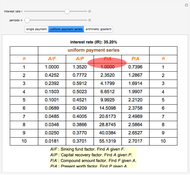
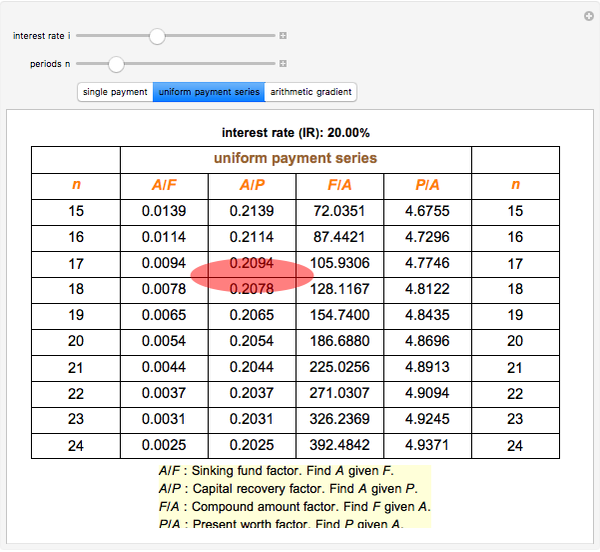
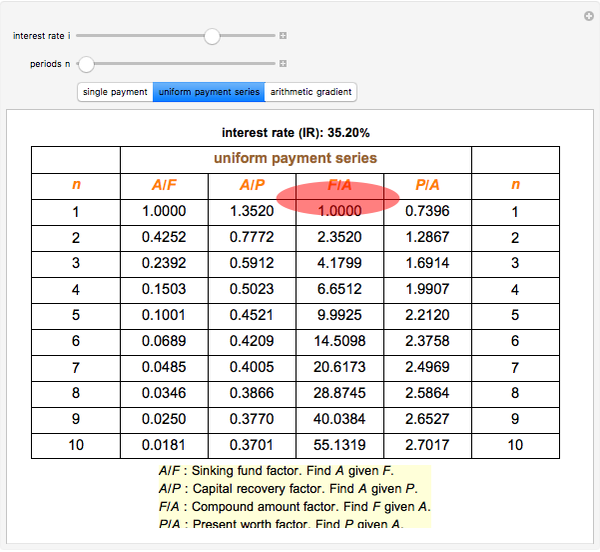
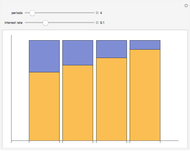
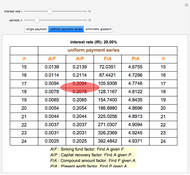
A uniform payment means that instead of a one-time investment, equal amounts are paid into a fund that compounds at interest rate  for each of the periods. "
for each of the periods. " given
given  " gives the portion of the desired future accumulated amount that must be contributed at each time period to reach the desired goal. "
" gives the portion of the desired future accumulated amount that must be contributed at each time period to reach the desired goal. " given
given  " shows the amount that must be paid to amortize an amount
" shows the amount that must be paid to amortize an amount  borrowed over the same period, with interest paid rather than earned on the outstanding balance—for example, a conventional mortgage.
borrowed over the same period, with interest paid rather than earned on the outstanding balance—for example, a conventional mortgage.
The inverses of those quantities are " given
given  " and "
" and " given
given  ". Notice that "
". Notice that " given
given  " shows the value of the accumulated sum of an amount
" shows the value of the accumulated sum of an amount  , saved in each of
, saved in each of  periods and compounding at interest rate
periods and compounding at interest rate  . On the other hand, "
. On the other hand, " given
given  " is the present value
" is the present value  of an amount that can be paid off by making
of an amount that can be paid off by making  payments of amount
payments of amount  .
.
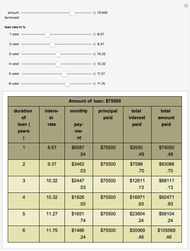
For example, the  factor for 30 years at 5% is 15.3725, so payments of $12,000 a year would pay off a loan of 12000×15.3725 = $184,470 at that rate over that period. The
factor for 30 years at 5% is 15.3725, so payments of $12,000 a year would pay off a loan of 12000×15.3725 = $184,470 at that rate over that period. The  factor of 66.4388 means that the same $12,000 a year contributed to, for example, a retirement savings account earning 5% would grow in 30 years to $797,265.60.
factor of 66.4388 means that the same $12,000 a year contributed to, for example, a retirement savings account earning 5% would grow in 30 years to $797,265.60.
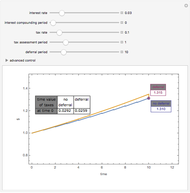
The arithmetic gradient series shows accumulations and amortizations possible by using increasing (rather than level) payments, where the amount of the payment starts at zero, but changes by  dollars per year. Otherwise, this part of the Demonstration should be interpreted like the level payment accumulation and amortization factor series. Note that series of payments or contributions that start above zero and then increase at a fixed rate can be calculated as the sum of two terms—a level payment series for the initial amount and a gradient series for the increases.
dollars per year. Otherwise, this part of the Demonstration should be interpreted like the level payment accumulation and amortization factor series. Note that series of payments or contributions that start above zero and then increase at a fixed rate can be calculated as the sum of two terms—a level payment series for the initial amount and a gradient series for the increases.
Reference: T. G. Eschenbach, D. G. Newnan, and J. P. Lavelle, Engineering Economic Analysis, New York: Oxford Univ. Press, 2004.
Permanent Citation