Wolfram Demonstrations Project

12,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

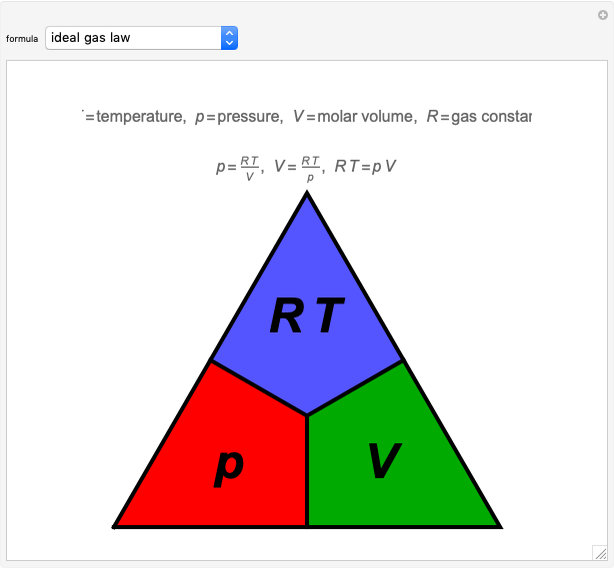
Diagrammatic Representations of Scientific Formulas

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

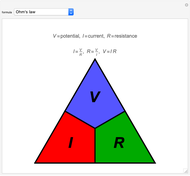
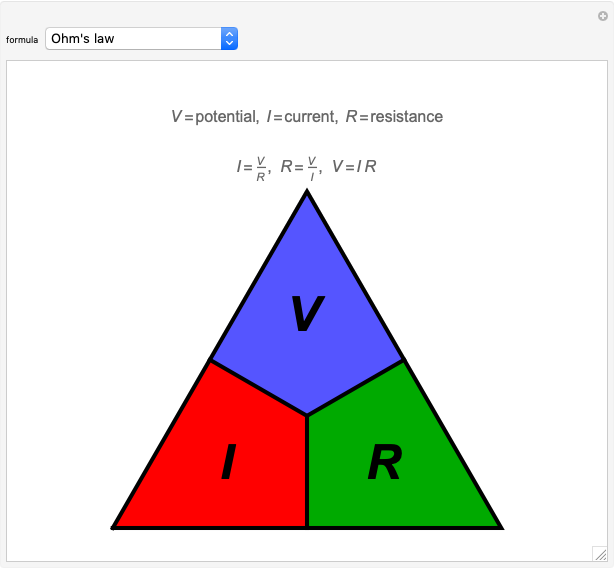
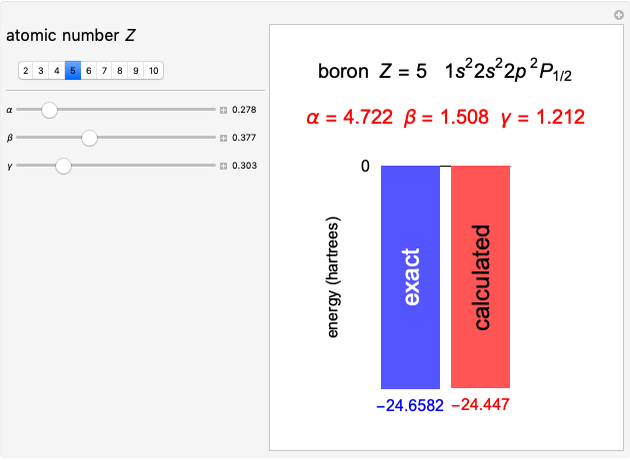
Ohm's law for electrical circuits can be read off a triangular diagram formed from the variables , and :

[more]

The diagram represents Ohm's law, solved for each of the variables, namely:

, and .

Several other formulas in physics and chemistry, in which it is useful to solve for each of its variables, can likewise be represented by analogous diagrams. You can select diagrams pertaining to electrical power, ideal gases, density, speed and distance. A diagram for the Pythagorean theorem is also shown, in which the relationships involve addition and subtraction, rather than multiplication and division.

This Demonstration also shows a diagrammatic representation for the thermodynamic potentials and Maxwell's relations.

Run the tooltip over a symbol in a diagram to show the formula solved for that variable.

[less]

Contributed by: S. M. Blinder (June 2020)
Open content licensed under CC BY-NC-SA

Snapshots

Details

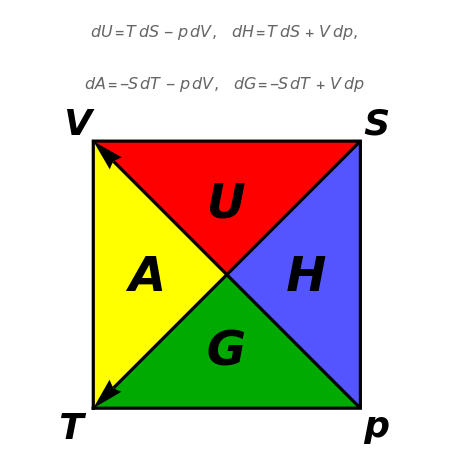
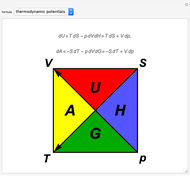
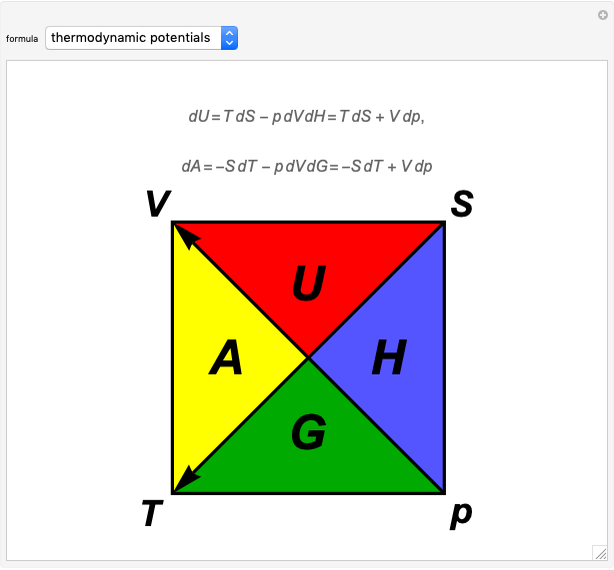
The thermodynamic potentials for a one-component system are energy , enthalpy , Helmholtz free energy and Gibbs free energy , each of which is a natural function of two state variables from among pressure , volume , temperature and entropy . The diagram identifies this functional dependence by the two vertices of the triangles for each of the thermodynamic functions. The fundamental differential relations for the potentials are given by

,

,

,

.

The minus signs in these formulas can be deduced from the directions of the two diagonal arrows in the diagram. The Euler reciprocity conditions for each of the potentials, since they are exact differentials, give the four Maxwell relations:

,

,

,

,

which can also be read off the diagram.

References

[1] Ohm Law. "Ohm's Law Triangle." (Apr 29, 2020) ohmlaw.com/ohms-law-triangle.

[2] Wikipedia. "Thermodynamic Square." (Apr 29, 2020) www.eoht.info/page/Thermodynamic+square.

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send