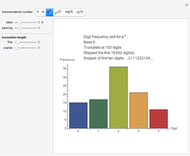
Digit Frequency Plot for Transcendental Numbers

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
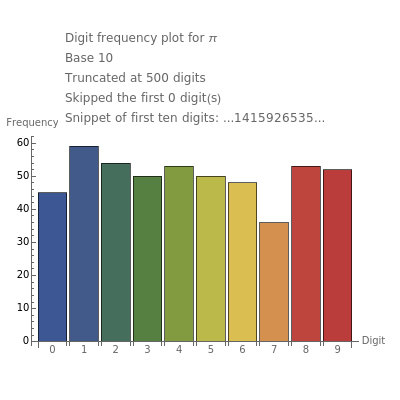
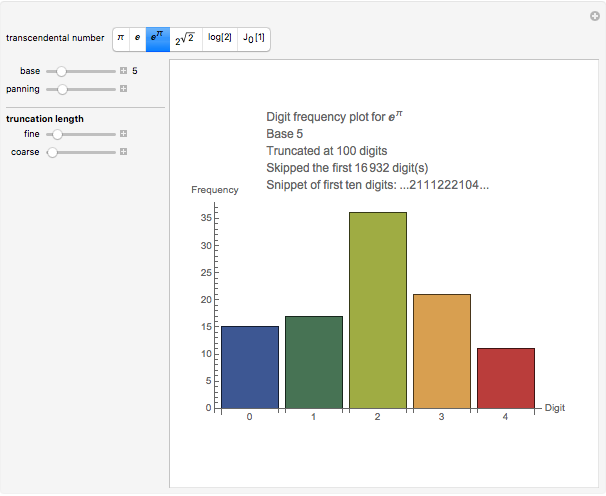
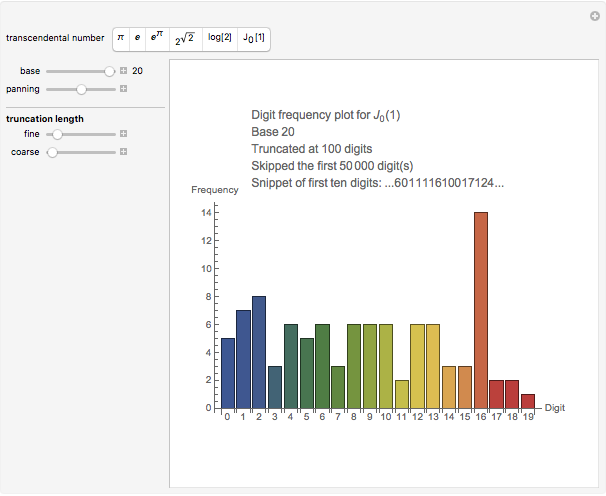
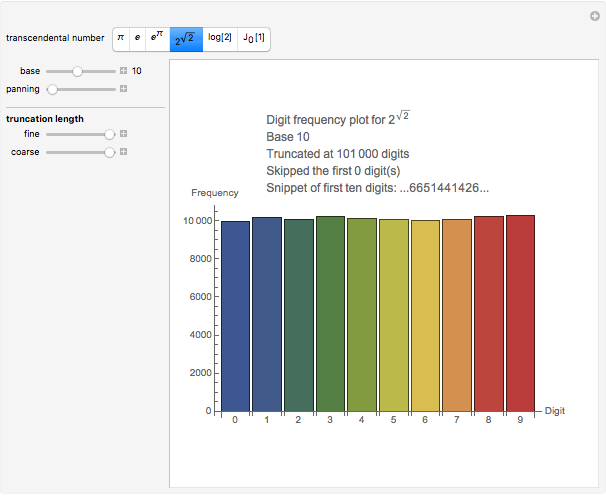
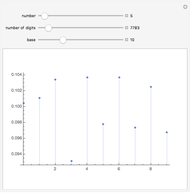
An algebraic number is the solution of a polynomial equation over the integers. A transcendental number is a number that is not algebraic. There are countably many algebraic numbers and although there are uncountably many transcendental numbers, not many have been proved to be so. This Demonstration plots the frequency of the digits in a variety of bases and truncation lengths after the decimal of a selection of transcendental numbers:  ,
,  ,
,  ,
,  ,
,  ,
,  .
.
Contributed by: Owen Barrett (March 2011)
Suggested by: Rob Morris
Open content licensed under CC BY-NC-SA
Snapshots
Details
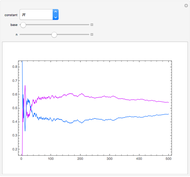
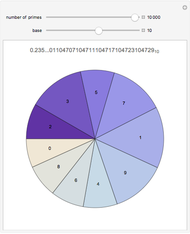
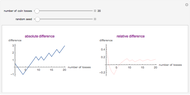
After selecting a transcendental number, choose a base between 2 and 20 in which to represent it. The "panning" slider lets you choose how many digits after the decimal to skip (a value of 10 will skip the first 10 digits after the decimal of the transcendental). The two truncation sliders, titled "fine truncation length" and "coarse truncation length", let you specify how many digits past the digit specified by the panning value you wish to include in the calculation. The Demonstration then tallies the frequency of each digit in the sample you have specified and displays the results in a bar chart.
Permanent Citation
"Digit Frequency Plot for Transcendental Numbers"
http://demonstrations.wolfram.com/DigitFrequencyPlotForTranscendentalNumbers/
Wolfram Demonstrations Project
Published: March 7 2011