Discrete Population Model for Fishery Stocks

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
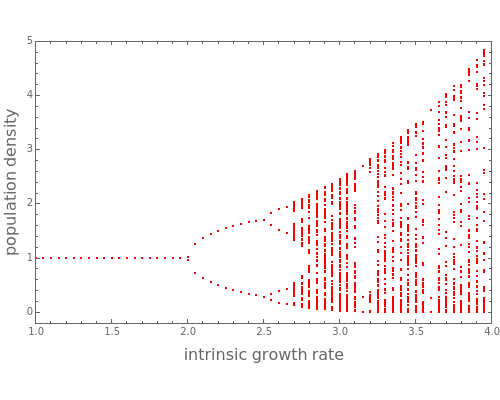
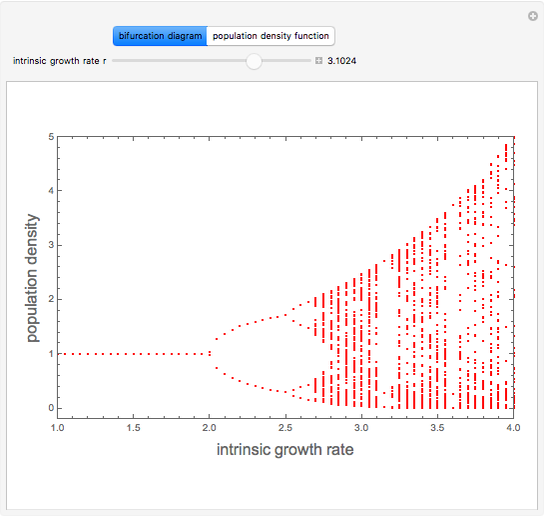
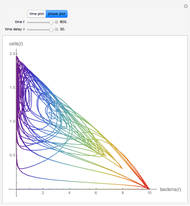
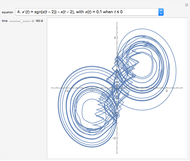
This Demonstration displays the bifurcation diagram for a realistic population dynamic model given by  , where
, where  is the intrinsic growth rate (taken here as a bifurcation parameter),
is the intrinsic growth rate (taken here as a bifurcation parameter),  is the number of fish (or density of population) at generation
is the number of fish (or density of population) at generation  , and
, and  is the population capacity of the environment, set equal to 1 here. This mathematical expression was given by W. E. Ricker (1954), who invented a discrete population model for fishery stocks. The model can be used to predict the number of fish in a fishery. When the cycle of period three appears,
is the population capacity of the environment, set equal to 1 here. This mathematical expression was given by W. E. Ricker (1954), who invented a discrete population model for fishery stocks. The model can be used to predict the number of fish in a fishery. When the cycle of period three appears,  . As expected, for higher values of
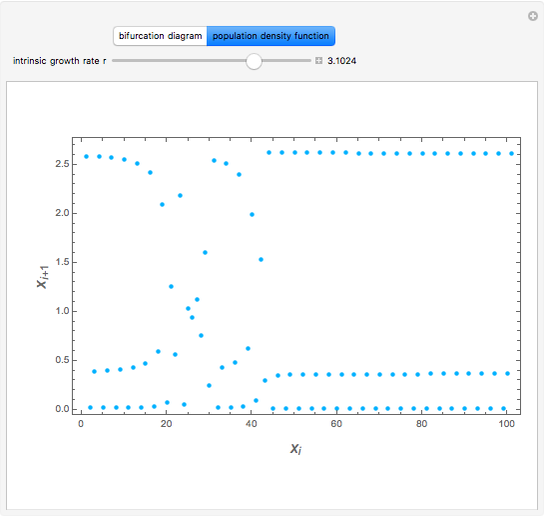
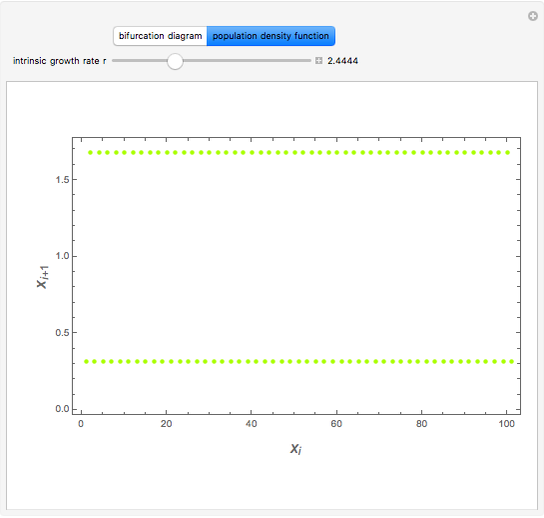
. As expected, for higher values of  , we observe chaotic behavior. Snapshots 2 to 5 present period three
, we observe chaotic behavior. Snapshots 2 to 5 present period three  , period two
, period two  , period four
, period four  , and chaotic behaviors
, and chaotic behaviors  , respectively.
, respectively.
Contributed by: Housam Binous, Brian G. Higgins, and Ahmed Bellagi (January 2013)
Open content licensed under CC BY-NC-SA
Snapshots
Details
References
[1] A. Varma and M. Morbidelli, Mathematical Methods in Chemical Engineering, New York: Oxford University Press, 1997.
[2] W. E. Ricker, "Stock and Recruitment," Journal of the Fisheries Research Board of Canada, 11(5), 1954 pp. 559–623. doi:10.1139/f54-039.
Permanent Citation
"Discrete Population Model for Fishery Stocks"
http://demonstrations.wolfram.com/DiscretePopulationModelForFisheryStocks/
Wolfram Demonstrations Project
Published: January 3 2013