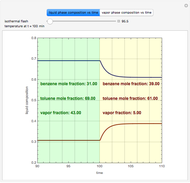
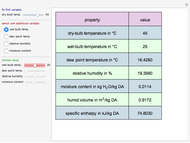
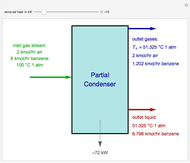
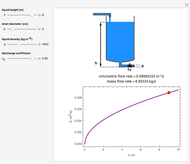
,

,

,

where is the cross-sectional area of a tank and is related to the discharge coefficient for the exit pipes.

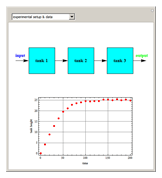
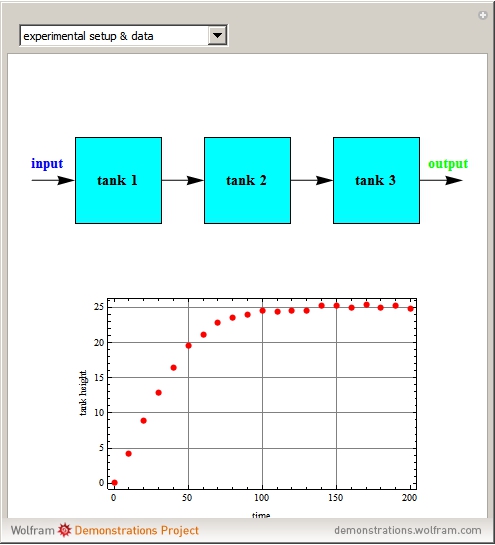
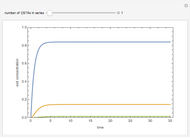
Suppose the height of tank 3 is sampled for a given input function to give the following data list: .

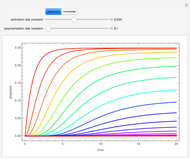
Then the constants and can be estimated using a least-squares optimization method. That is, we define the following objective function

.

Here is the height in tank 3 predicted by the model at time , and is the value of measured at time . The goal then is to determine and such that sum of squares is minimized for spanning the duration of the experiment.

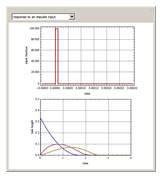
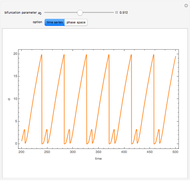
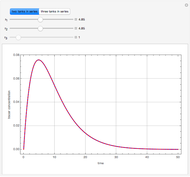
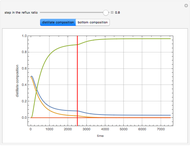
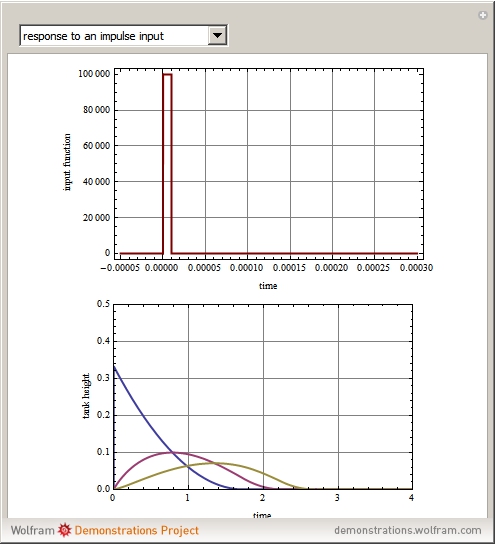
One finds as shown in the second snapshot and . It is possible then to solve the governing equations shown above and determine the height of tanks 1 and 2. The second snapshot presents the height versus time for tanks 1, 2, and 3 in blue, magenta, and brown, respectively.

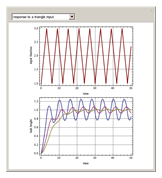
Once and have been determined, one can run simulations for various forms of the input function: impulse input, triangle input, square input, and staircase input. The subsequent snapshots show the responses for all the above mentioned special input functions, which are shown in red in a separate plot.