Early Exercise of American Options

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
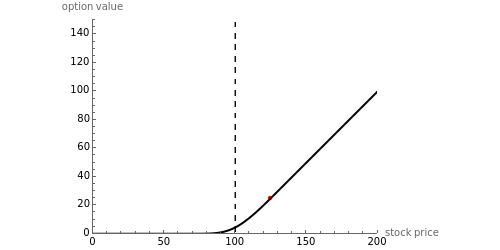
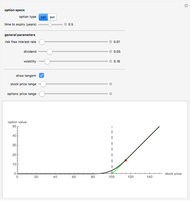
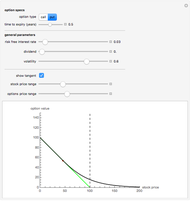
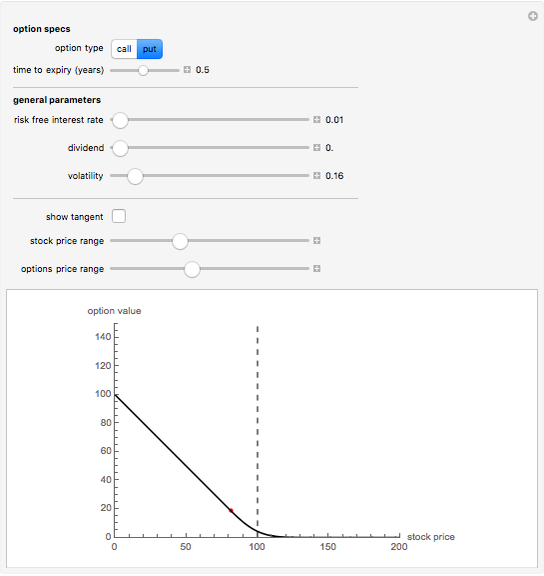
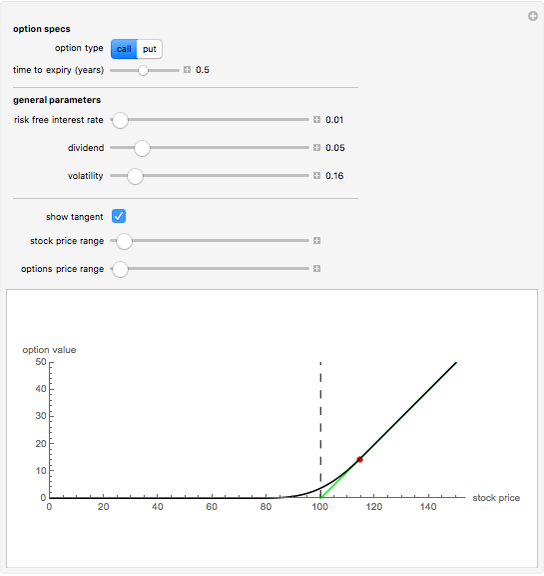
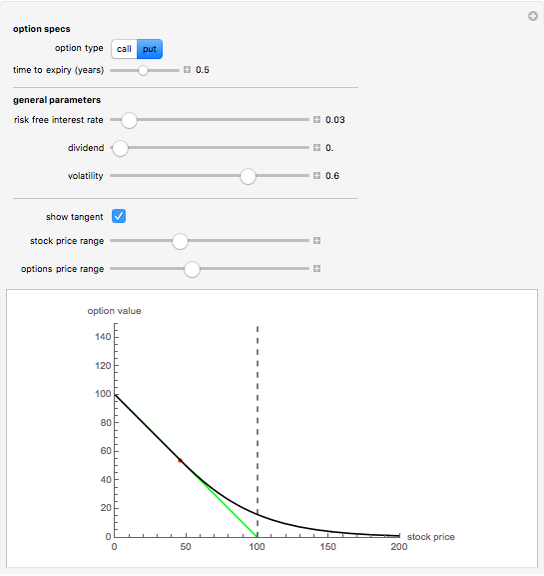
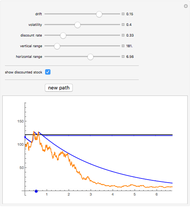
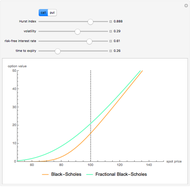
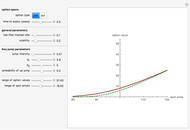
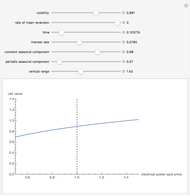
This Demonstration shows the optimal value for the exercise of an American option (call or put) in the Black–Scholes model. Unlike the European option, the American option allows early exercise. One can show that for all put options there is a price of the underlying stock such that when the stock is at (or below) this price, the option should be exercised. For call options on a stock that pays a nonzero continuous dividend, there is a stock price such that the option should be exercised when the stock price is at or above this optimal price. It is never optimal to exercise call options that pay no dividend.
[more]
Contributed by: Andrzej Kozlowski (March 2011)
Open content licensed under CC BY-NC-SA
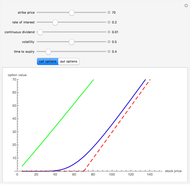
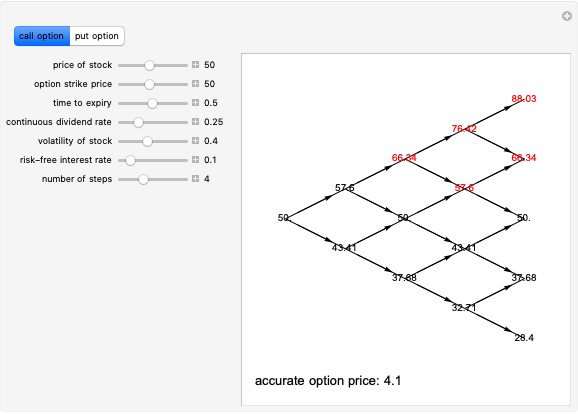
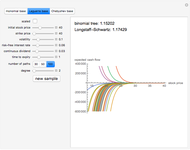
Snapshots
Details
The problem of computing the optimal exercise of an American option is known as a free-boundary problem for the associated Black–Scholes partial differential equations. The function FinancialDerivative in Mathematica 8 can efficiently compute the optimal exercise price of an American option (in the Black–Scholes model) without the need to explicitly set up and solve the corresponding free-boundary problem for the Black–Scholes PDE.
Reference
[1] P. Wilmott and J. Dewynne, Option Pricing, Mathematical Models and Computation, Oxford: Oxford Financial Press, 1993.
Permanent Citation
"Early Exercise of American Options"
http://demonstrations.wolfram.com/EarlyExerciseOfAmericanOptions/
Wolfram Demonstrations Project
Published: March 7 2011