Details

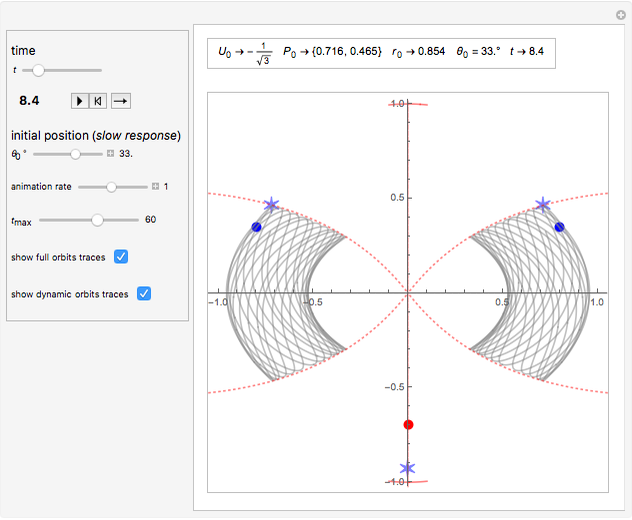
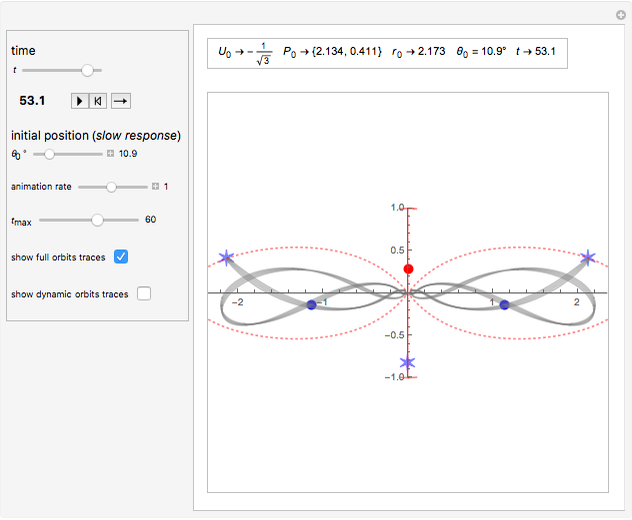
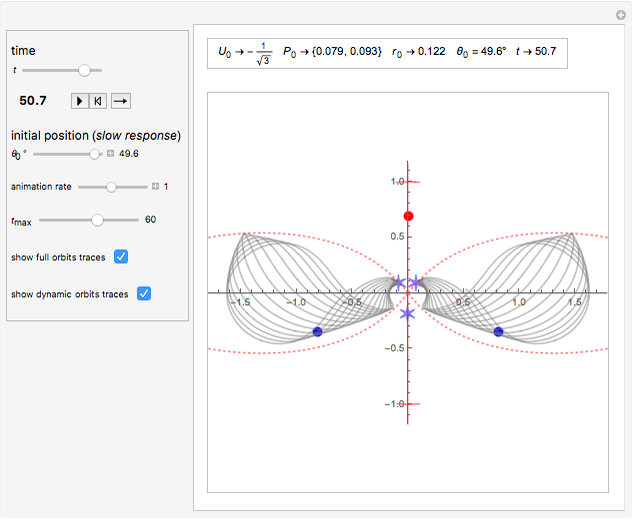
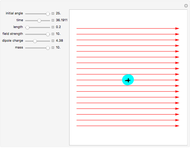
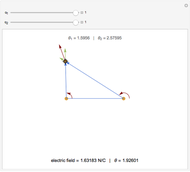
In this Demonstration, the initial position of the rightmost charge is determined just by its angular position with respect to the origin. Given the fixed initial energy of the system and , the radial position is set by the formula:

.

In Cartesian coordinates, the total potential energy is given by

.

Initially, the three charges form an equilateral triangle inscribed in an unitary circle, and with unitary charges, masses and electric constant , it is

,

where is the initial position of the right-hand particle.

is fixed because this factor only changes the scaling of the orbits and not their qualitative shapes. The positions of the other two charges are determined by the position of the first one, since the three must always form an isosceles triangle with the axis as the axis of symmetry and with the center of mass at the origin.

The evolution of the system is then governed by the Coulomb forces acting on the right-hand particle, due to the presence of the other two particles.

Since the Coulomb force is conservative, the total energy is conserved:

.

(The charges have zero kinetic energy at .) A negative initial energy ensures that the system is bound and that the charges will never escape to infinity.

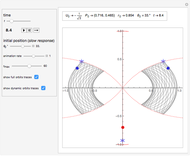
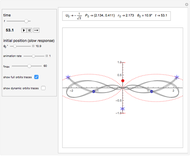
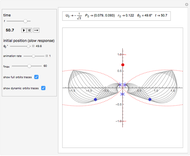
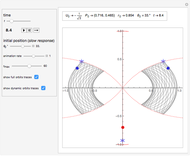
The lines with constant potential are shown as dotted lines and delimit the region where the blue particles can move. At these lines, a particle has zero speed.

The purpose of this Demonstration is to explore the different kinds of trajectories that this system can produce with different initial conditions; that is, with different isosceles triangles, by changing the parameter . Some trajectories look periodic and some look quasiperiodic. For instance, the equilateral triangle setting appears to produce a periodic orbit (with a period of about 39.1 s).

References

[1] S. Strogatz, Nonlinear Dynamics and Chaos: With Applications to Physics, Biology, Chemistry, and Engineering, Reading, MA: Addison-Wesley Pub., 1994.

[2] Wikipedia. "Coulomb's Law." (Jan 4, 2017) en.wikipedia.org/wiki/Coulomb's_law.

[3] L. Moroni, "Electric Dance," Worlds of Math & Physics (blog). (Jan 4, 2017) www.lucamoroni.it/electric-dance-page.

[4] L. Moroni, "Electric Dance,"YouTube video" (Jan 4, 2017) https://youtu.be/nqAcMm2o-kk