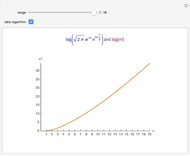
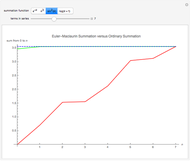
Euler-Maclaurin Summation Formula

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
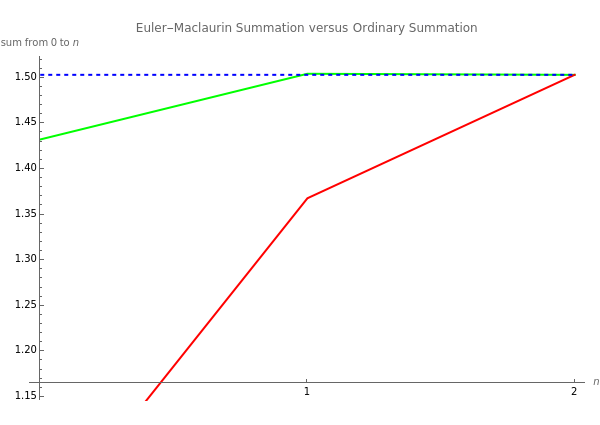
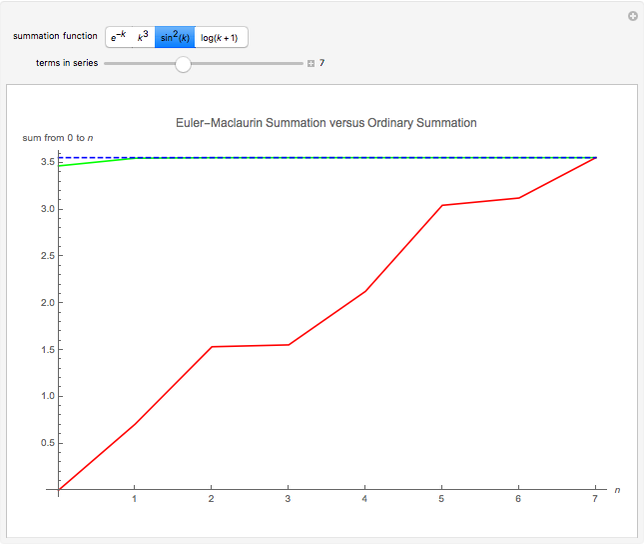
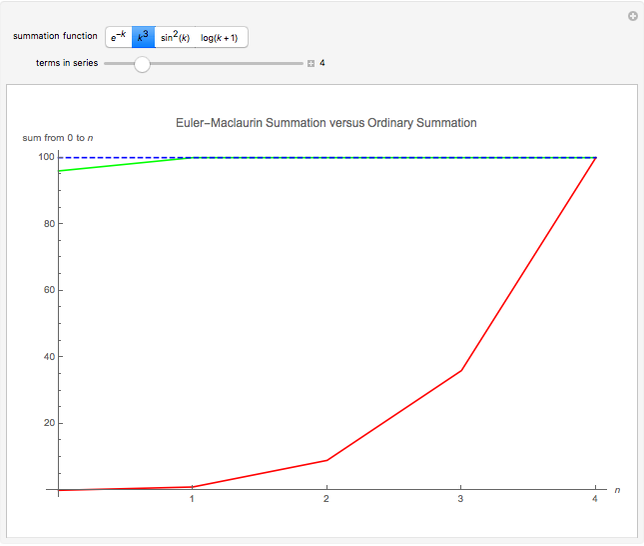
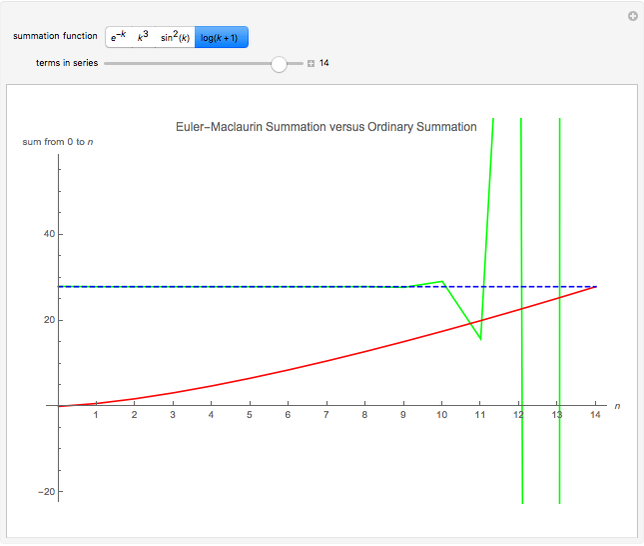
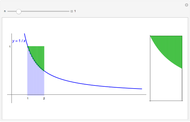
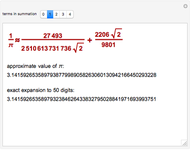
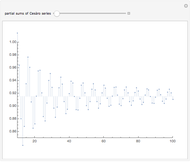
The Euler–Maclaurin summation formula transforms a sum over the values of a function to a sum over the derivatives of a function. Its main advantage is that the new sum usually converges much more quickly than original. One must be careful, though, as the error term may not go to zero as more and more terms in the series are taken, as is shown by 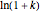 .
.
Contributed by: Sam Nicoll (January 2012)
Open content licensed under CC BY-NC-SA
Snapshots
Details
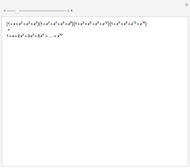
The Euler–Maclaurin formula reads 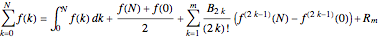 ,
where
,
where 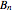 is the
is the 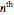 Bernoulli number and
Bernoulli number and 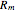 is a remainder term. The power of the Euler–Maclaurin summation formula lies in how quickly the new sum converges, even if the error term is unbounded.
is a remainder term. The power of the Euler–Maclaurin summation formula lies in how quickly the new sum converges, even if the error term is unbounded.
Permanent Citation