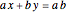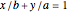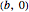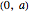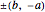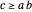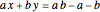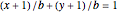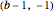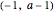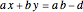Frobenius Equation in Two Variables
Initializing live version

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
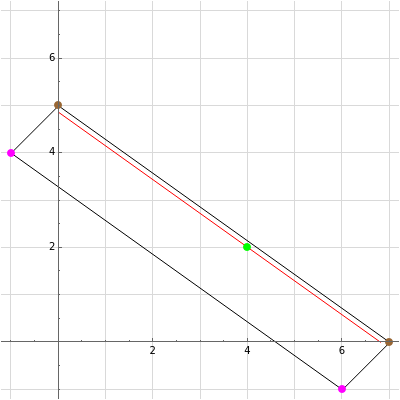
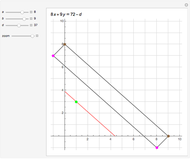
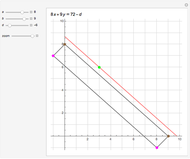
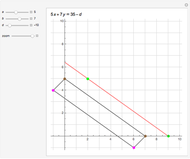
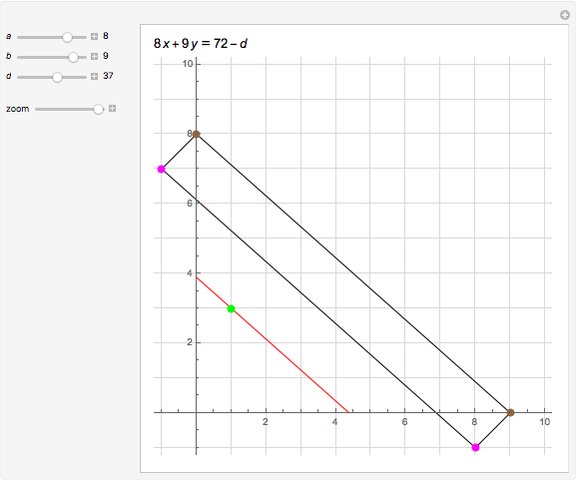
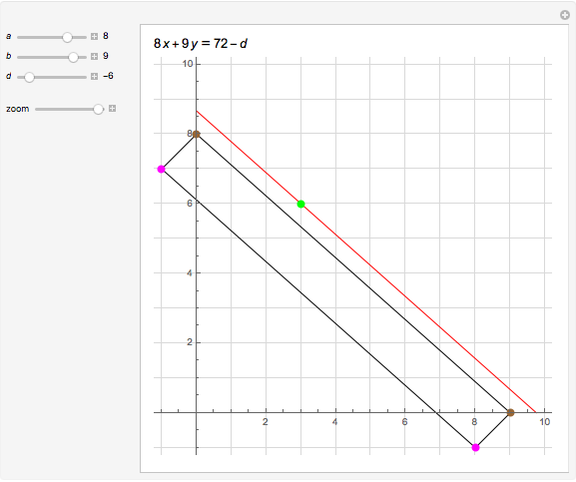
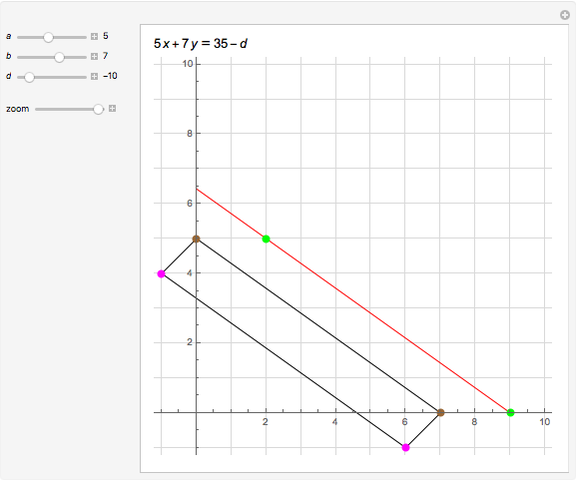
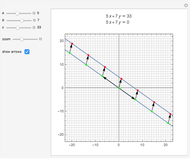
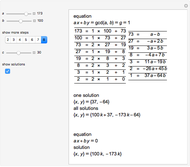
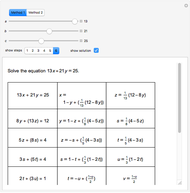
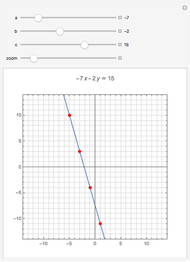
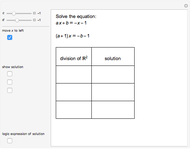
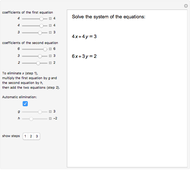
The Frobenius equation in two variables is a Diophantine equation 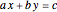 , where
, where 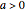 and
and 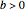 . The Frobenius number of the coefficients
. The Frobenius number of the coefficients  and
and  , where
, where  and
and  are relatively prime, is the largest
are relatively prime, is the largest  for which the equation
for which the equation 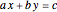 has no non-negative solutions. Sylvester (1884) showed that
has no non-negative solutions. Sylvester (1884) showed that 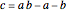 .
.
Contributed by: Izidor Hafner (April 2015)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Permanent Citation