Generating 3D Figures with a Given Symmetry Group

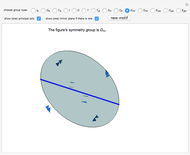
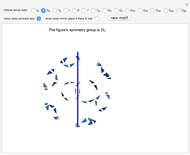
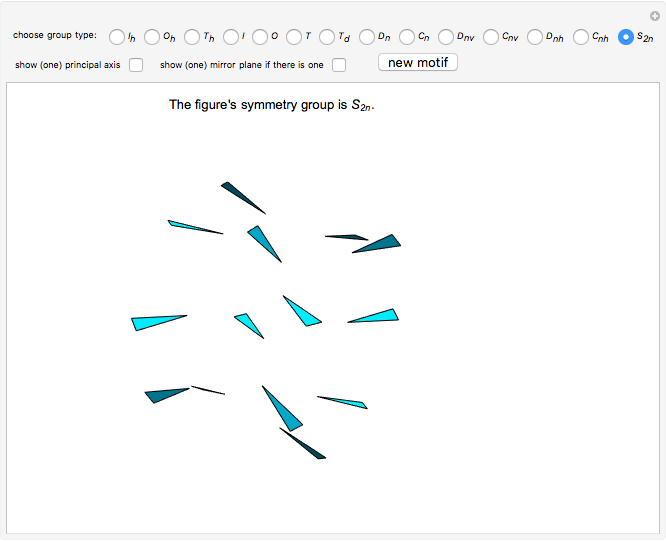
If the figure has only one rotational axis, there are the four possible kinds of symmetries, all cyclic: 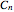(there is an axis of rotation and reflection, but there is no mirror plane), 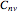(there is a mirror plane, but it is not perpendicular to the axis), 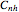(there is a mirror plane that is perpendicular to the axis), and 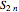(there is a glide reflection).

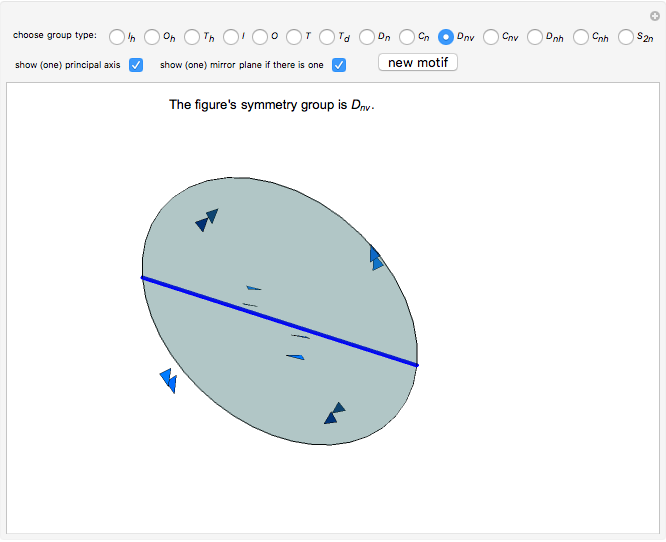
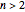If the figure has more than one rotational axis but no more than one -fold axis with , the possibilities are (dihedral symmetries): 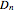(no mirror plane), 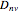(the mirror plane is not perpendicular to the principal axis), 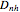(the mirror plane is perpendicular to the principal axis).

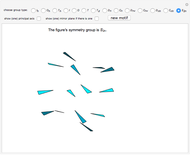
The figure may have more than one 5-fold axis (icosahedral symmetry): 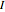(rotations only), 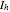(there is a mirror plane).

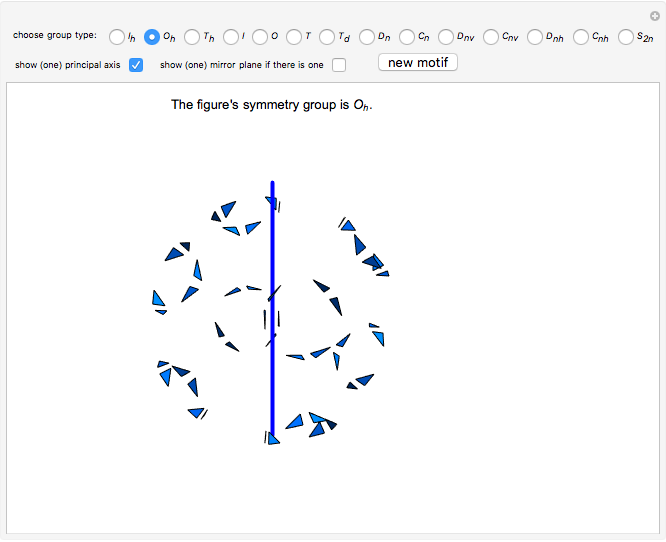
The figure may have more than one 4-fold axis (octahedral symmetry): 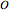(rotations only), 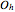(there is a mirror plane).

The figure may have more than one principal 3-fold axis (tetrahedral symmetry): 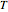(rotations only), 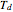(there is a mirror plane, no inversion), 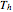(there is a point of inversion).

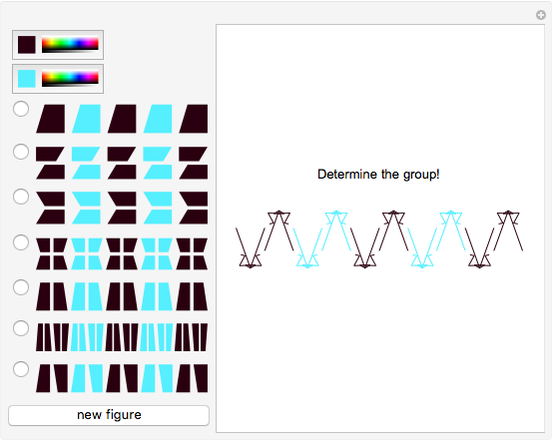
This Demonstration is a guessing game to learn about the 14 types of symmetry groups of figures that have a rotational axis.

Not demonstrated are the three symmetry groups with no rotational symmetry: 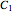(asymmetric ), 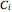(only inversion), and 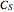(only one mirror plane).

Reference

[1] P. R. Cromwell, Polyhedra, New York: Cambridge University Press, 1999 pp. 289–313. www.liv.ac.uk/~spmr02/book/index.html.