Mathematica MathWorld Wolfram Science More »

Graphical Linear Programming for Two Variables


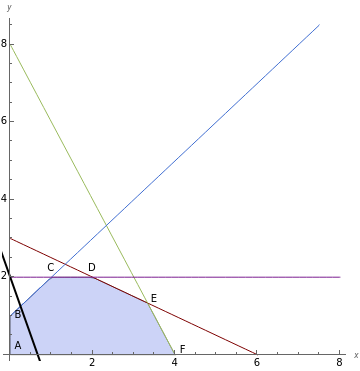
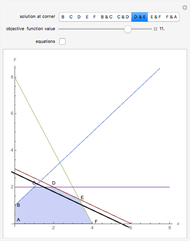
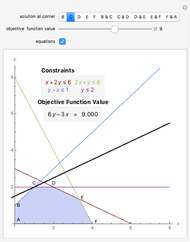
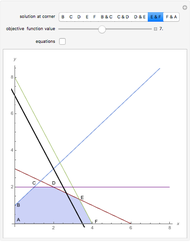
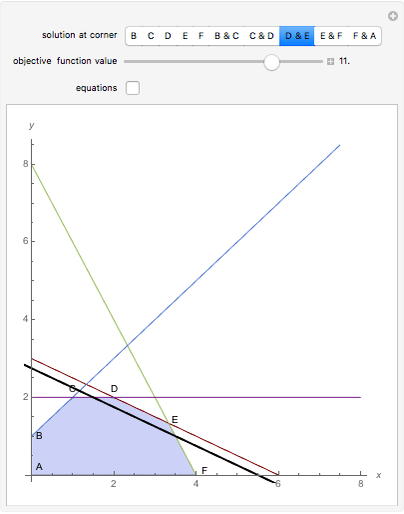
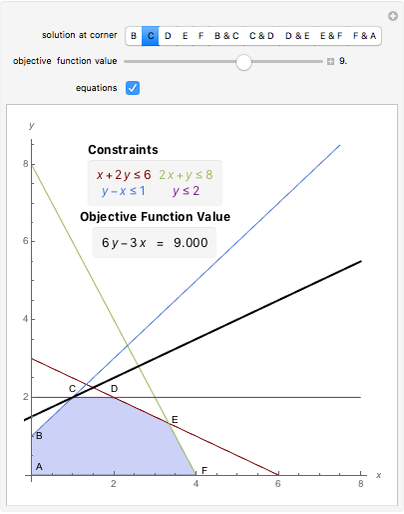
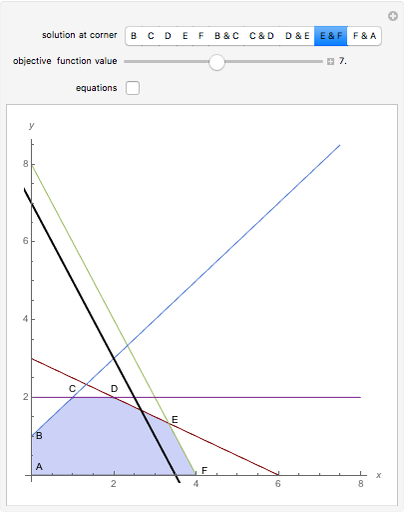
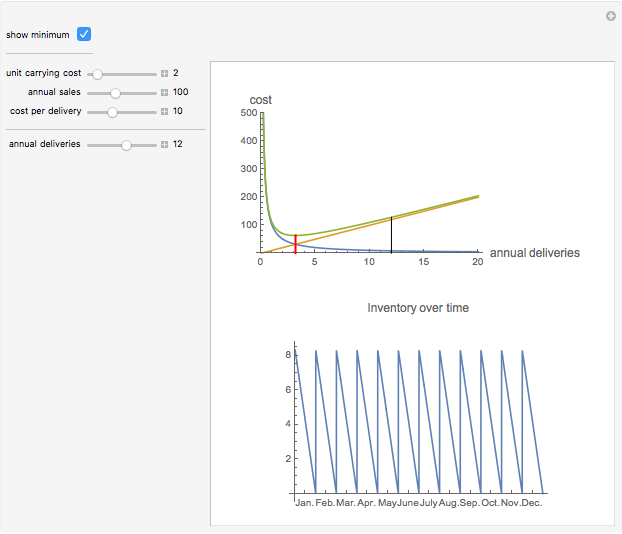
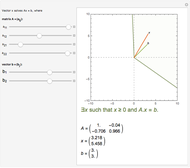
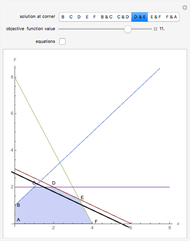
	This Demonstration illustrates the graphical solution to several linear programming problems, all of which have the same set of constraints; you can vary the objective function. When two corner points are optimal, so are all the points on the line segment connecting them. The region shaded in blue is the feasible region and the colored lines correspond to the constraints. The black line represents the chosen objective function set to the slider value.


	Contributed by: Olivia M. Carducci (East Stroudsburg University)
	Show Initialization Code

In this Demonstration, it is not possible to choose an objective function that makes

optimal because such an objective function would either involve a minimization problem or a maximization problem with negative coefficients for

and

. Such problems would require different values for the right hand side and so do not fit well with the slider. You cannot make

and

optimal because such an objective function would be a vertical line.

Contributed by: Olivia M. Carducci (East Stroudsburg University)

	Source page:
	Email	Name (optional)
	Occupation (optional)	Organization (optional)
	I use Mathematica often occasionally never
	Country (optional) Remember me
	Note: Please do not include anything you consider confidential or proprietary. Your message and contact information may be shared with the author of any specific Demonstration for which you give feedback, but will not otherwise be published or distributed. Privacy Policy »

RSS

Atom