Wolfram Demonstrations Project

12,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

Grow an Alexander Horned Sphere

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

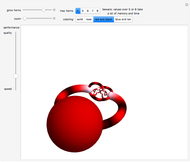
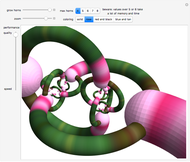
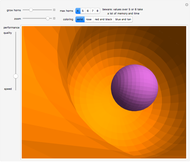
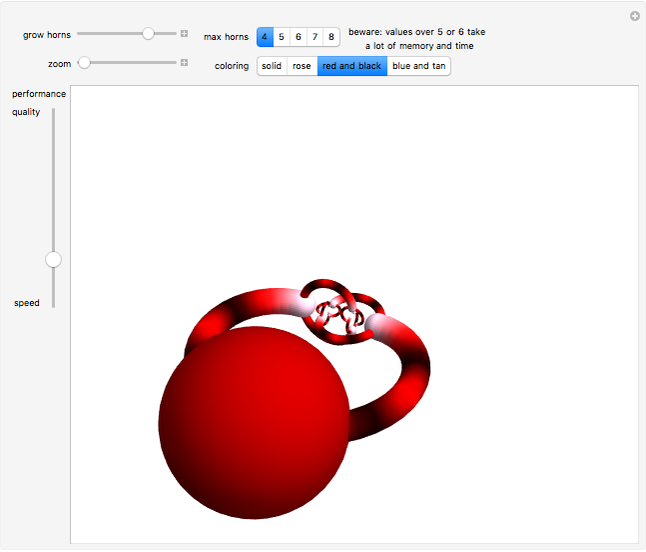
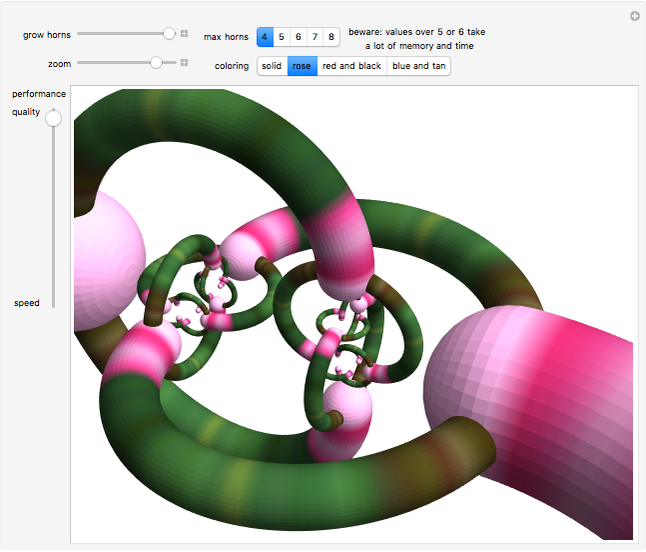
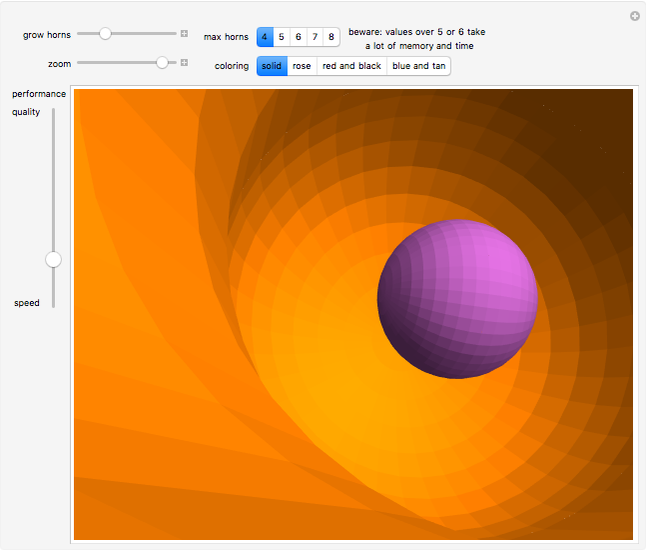
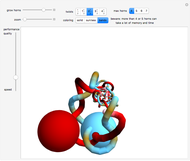
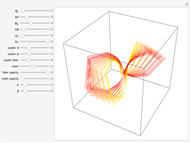
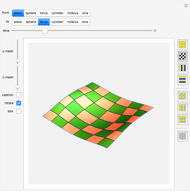
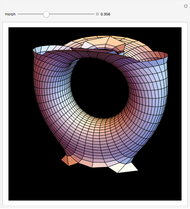
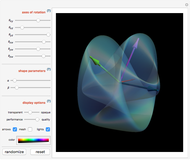
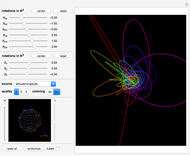
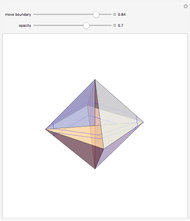
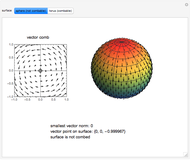
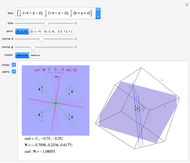
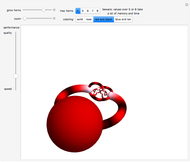
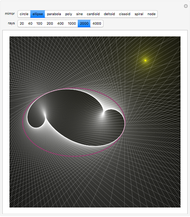
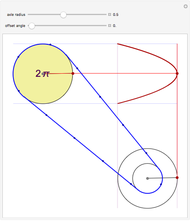
Move the slider to grow the horns on an Alexander horned sphere. Zoom in on the complicated limit point. Control the maximum number of times the horns divide. The performance slider controls how many segments make up each horn. Depending on the number of horns, selecting higher quality can result in slow performance in rendering the image.

Contributed by: Michael Rogers (Oxford College of Emory University) (March 2011)
Open content licensed under CC BY-NC-SA

Snapshots

Details

Alexander's horned sphere is a famous example, due to J. W. Alexander (1924), of a subset of space homeomorphic to a sphere whose exterior is not simply connected. In this construction the sphere is formed by recursively growing interlocking horns, each similar to the others. To get the complete sphere, the construction would have to be carried out to infinity. That is not possible, but this Demonstration can help get you started.

Note: you may rotate the 3D image as usual, but zooming can be done only by the slider. Also, panning does not work as expected, because of the way the program controls the image presented.

J. W. Alexander, "An Example of a Simply Connected Surface Bounding a Region Which Is Not Simply Connected," Proc. N. A. S., 10(1), 1924 pp. 8–10.

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send