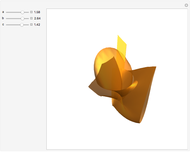
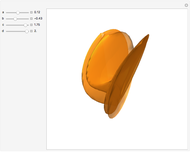
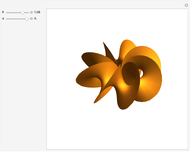
Henneberg's Minimal Surface

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
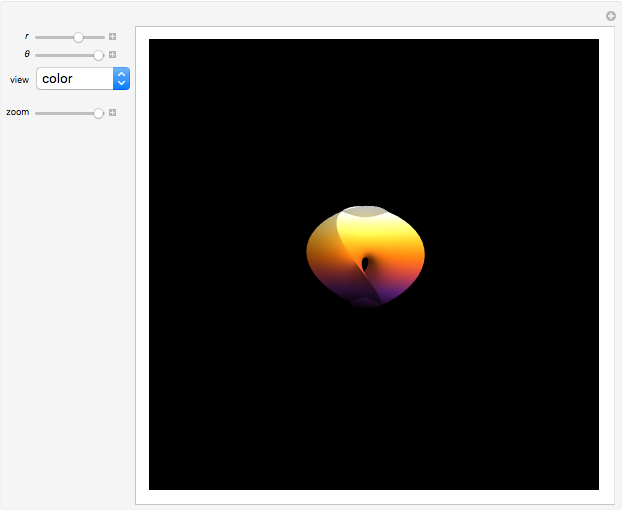
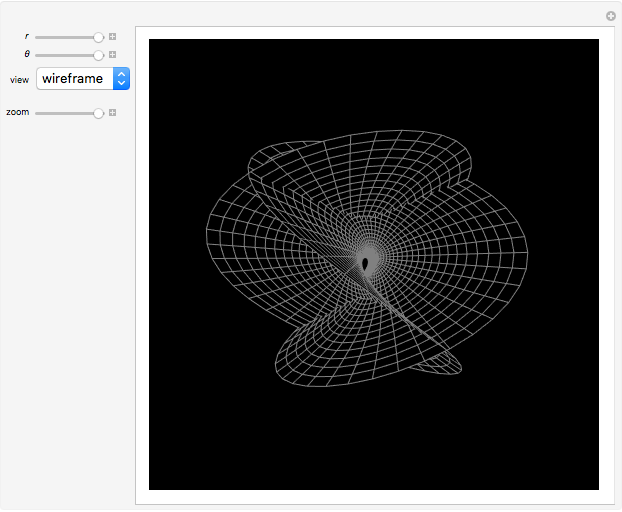
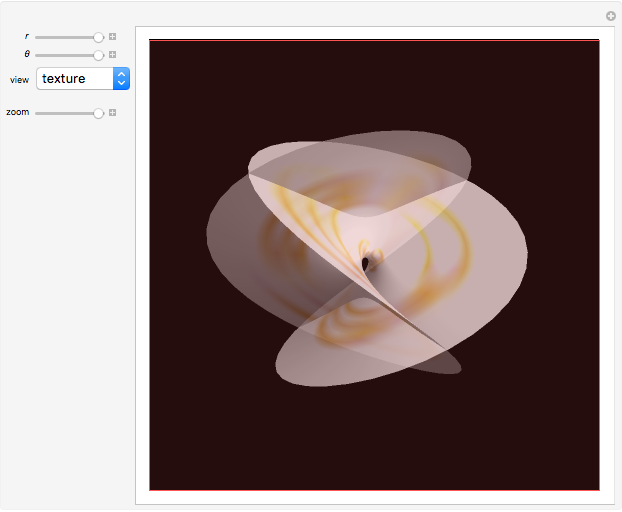
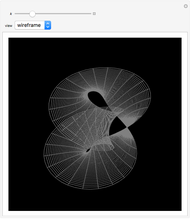
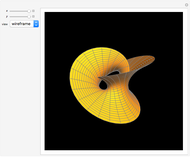
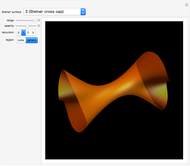
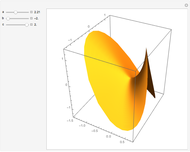
Henneberg's minimal surface (discovered by Lebrecht Henneberg in 1876) is a nonorientable surface (in fact, it contains a minimal Möbius band) defined over the unit disk, whose total curvature is  [1]. As well as other interesting surfaces, it was found by solving the Björling problem [2, 3].
[1]. As well as other interesting surfaces, it was found by solving the Björling problem [2, 3].
Contributed by: Enrique Zeleny (June 2014)
Open content licensed under CC BY-NC-SA
Snapshots
Details
The parameterization used here is
 .
.
References
[1] U. Dierkes, S. Hildebrandt, and F. Sauvigny, Minimal Surfaces, 2nd ed., Heidelberg, NY: Springer, 2010.
[2] Wikipedia. "Björling Problem." (Dec 30, 2013) en.wikipedia.org/wiki/Bj% C3 % B6rling_problem.
[3] K.-W. Fung, "Minimal Surfaces as Isotropic Curves in  : Associated Minimal Surfaces and the Björling's Problem," Bachelor's thesis, Department of Mathematics, Massachusetts Institute of Technology, 2004. ocw.mit.edu/courses/mathematics/18-994-seminar-in-geometry-fall-2004/projects/main1.pdf.
: Associated Minimal Surfaces and the Björling's Problem," Bachelor's thesis, Department of Mathematics, Massachusetts Institute of Technology, 2004. ocw.mit.edu/courses/mathematics/18-994-seminar-in-geometry-fall-2004/projects/main1.pdf.
Permanent Citation