Hydrogen-Like Continuum Eigenstates

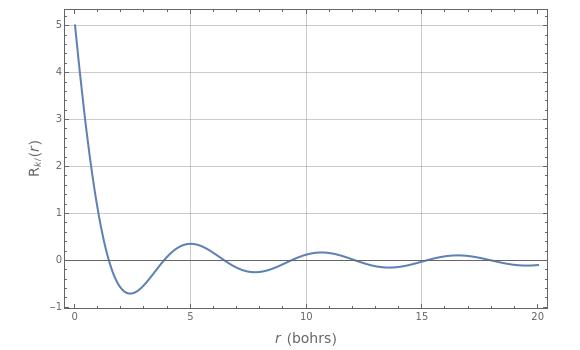
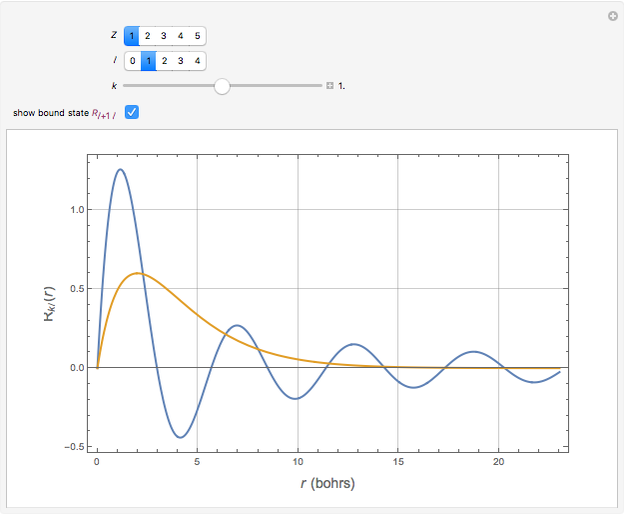
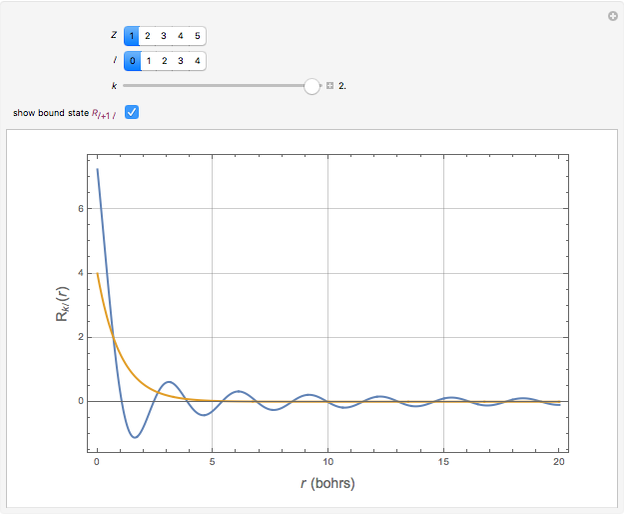
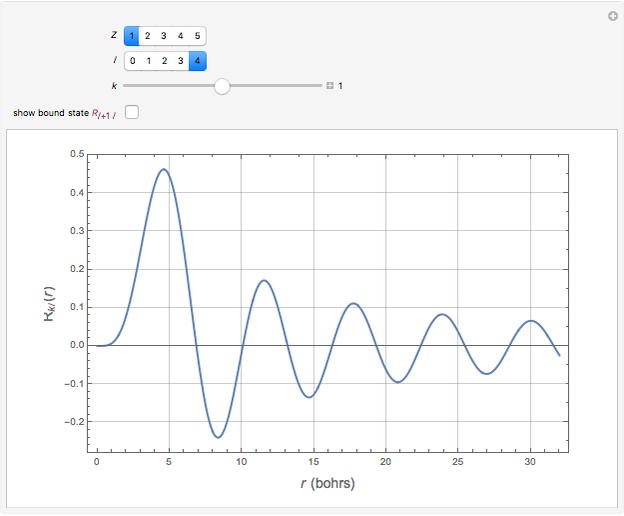
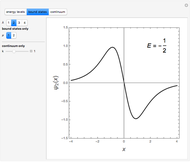
The positive-energy continuum states of a hydrogen-like system are described by the eigenfunctions with corresponding eigenvalues , (). are the same spherical harmonics that occur for the bound states. In atomic units , the radial equation can be written . The solutions with the appropriate analytic and boundary conditions have the form . These functions are deltafunction-normalized, such that . They have the same functional forms (apart from normalization constants) as the discrete eigenfunctions under the substitution .

You can plot the continuum function for various choices of , ℓ, and . The asymptotic form for large approaches a spherical wave of the form . The terms in the argument of cos, in addition to , represent the phase shift with respect to the free particle. Coulomb scattering generally involves a significant number of partial waves.

A checkbox lets you compare the lowest-energy bound state with the same ℓ, that is, the wavefunctions for , , , ⋯ (magnified by a factor of for better visualization). The solution, obtained as the limit of the discrete function as or of the continuum function as , has the form of a Bessel function: .