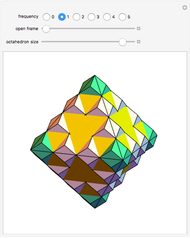
Icosahedron Fractal

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
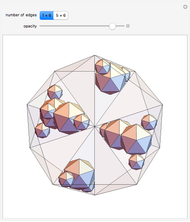
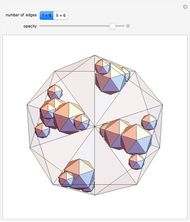
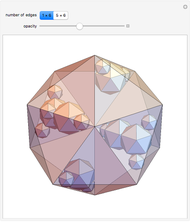
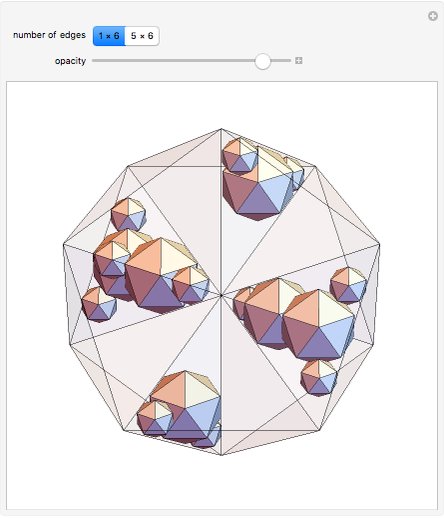
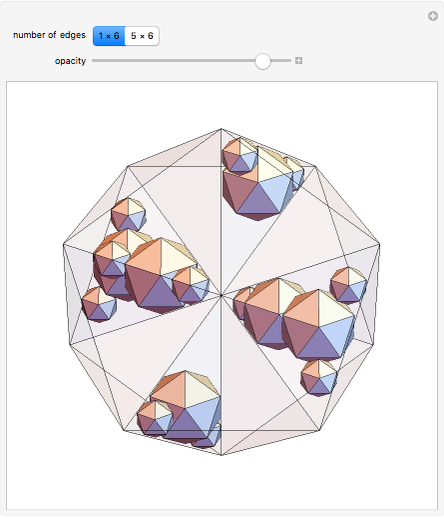
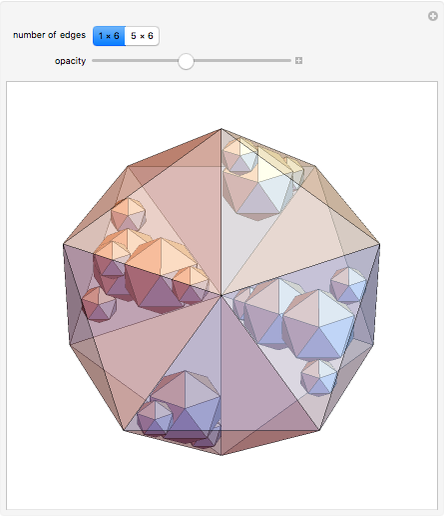
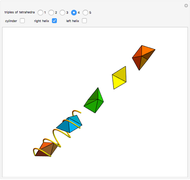
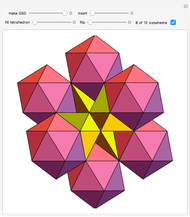
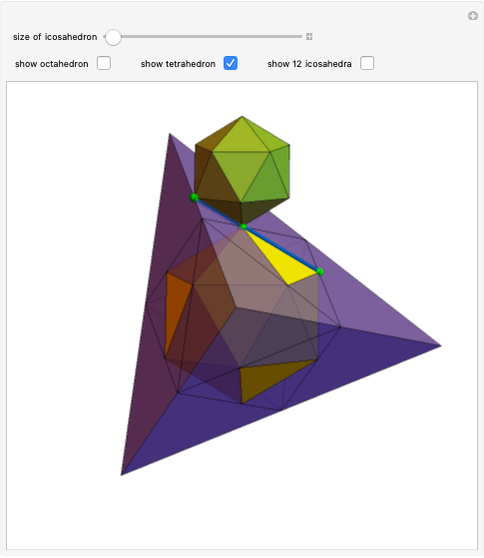
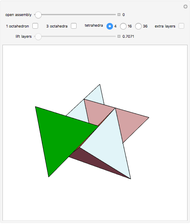
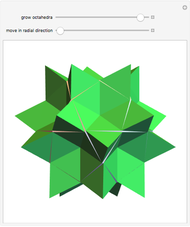
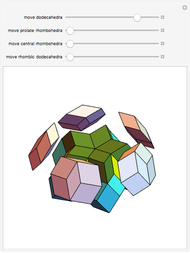
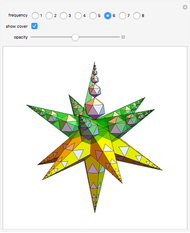
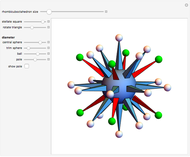
A double tower of icosahedra is aligned along each edge of a larger enveloping icosahedron. A tower stacks gradually diminishing icosahedra on the face of an icosahedron. The rate of reduction is 1/2, and each tower could theoretically have an infinite number of icosahedra converging to a vertex of the enveloping icosahedron. Each icosahedron in the assembly could be replaced with a fractal icosahedron to form an infinite fractal structure.
Contributed by: Sándor Kabai (April 2007)
Open content licensed under CC BY-NC-SA
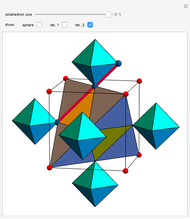
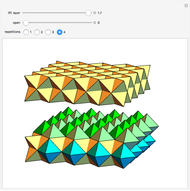
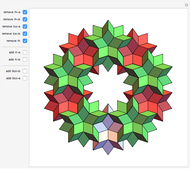
Snapshots
Details
This Demonstration can serve as a reminder of certain geometrical features—for instance, that the icosahedron has 5 6 edges, corresponding to the number of faces of the rhombic triacontahedron, and that the arrangement of 1
6 edges, corresponding to the number of faces of the rhombic triacontahedron, and that the arrangement of 1 6 edges corresponds to the faces of the cube. The assembly is a good illustration of the selfâ€Âsimilarity property of fractals. It also shows a geometrical example of how an infinite set of volumes can have a finite boundary. Related schoolroom exercises could include the calculation of the height of a tower, the volume of a tower, and the proportions of icosahedra.
6 edges corresponds to the faces of the cube. The assembly is a good illustration of the selfâ€Âsimilarity property of fractals. It also shows a geometrical example of how an infinite set of volumes can have a finite boundary. Related schoolroom exercises could include the calculation of the height of a tower, the volume of a tower, and the proportions of icosahedra.
Permanent Citation
"Icosahedron Fractal"
http://demonstrations.wolfram.com/IcosahedronFractal/
Wolfram Demonstrations Project
Published: April 27 2007