Kinetic Order of Degradation Reactions

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
Many chemical and biochemical degradation reactions follow fixed-order kinetics, including orders zero, one, or  , where
, where  need not be an integer. With the exception of first-order kinetics, which is characterized by exponential decay, the diminishing concentration versus time relationship can be described by a single formula over a range that is determined by the reactant's initial concentration,
need not be an integer. With the exception of first-order kinetics, which is characterized by exponential decay, the diminishing concentration versus time relationship can be described by a single formula over a range that is determined by the reactant's initial concentration,  , the reaction’s (temperature-dependent) rate constant,
, the reaction’s (temperature-dependent) rate constant,  , and the kinetic order,
, and the kinetic order,  . This Demonstration identifies the formula’s applicability range and lets you simulate and monitor the progress of degradation reactions following any kinetic order in the range 0–2.5, with different rate constants,
. This Demonstration identifies the formula’s applicability range and lets you simulate and monitor the progress of degradation reactions following any kinetic order in the range 0–2.5, with different rate constants,  , and starting at different initial concentrations.
, and starting at different initial concentrations.
Contributed by: Mark D. Normand and Micha Peleg (July 2013)
Open content licensed under CC BY-NC-SA
Snapshots
Details
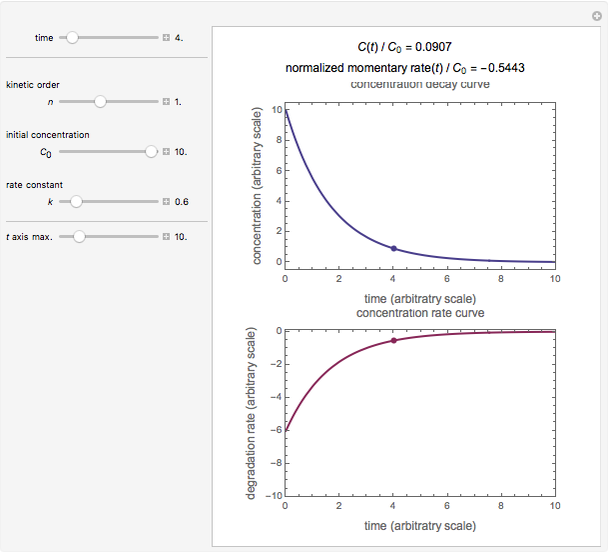
Snapshot 1: zero-order kinetics
Snapshot 2: first-order kinetics
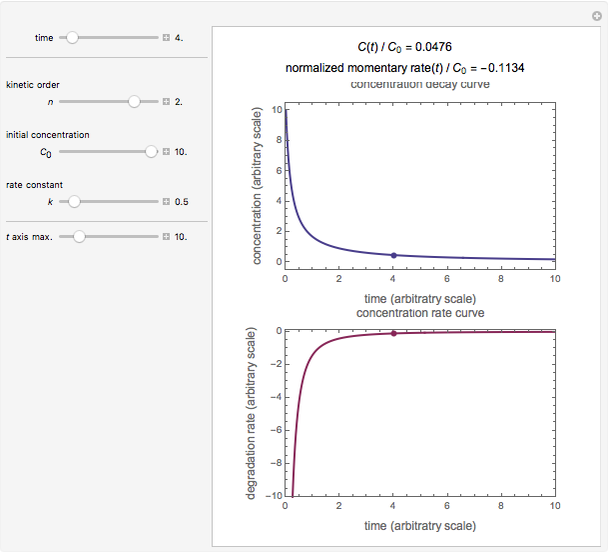
Snapshot 3: second-order kinetics
Snapshot 4: fractional-order kinetics ( )
)
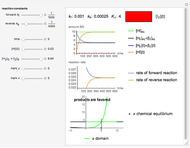
Isothermal degradation of chemical reactions and biological decay processes frequently follow fixed-order kinetics with the exponent  in the differential rate equation
in the differential rate equation  . In this equation,
. In this equation, 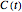 is the concentration at time
is the concentration at time  and
and  is the (temperature-dependent) rate constant in the corresponding units of concentration and time. For zero-order kinetics (
is the (temperature-dependent) rate constant in the corresponding units of concentration and time. For zero-order kinetics ( ),
),  , where
, where  is the initial concentration. For first-order kinetics (
is the initial concentration. For first-order kinetics ( ),
),  , and for any other order,
, and for any other order,  . Note that for
. Note that for  this equation reduces to
this equation reduces to  .
.
In an actual degradation process such as molecule disintegration, enzyme denaturation, or cell or organism mortality, neither the concentration nor density can have negative or complex values. Thus, the general equation for  -order kinetics is only applicable for
-order kinetics is only applicable for  ,
,  ,
,  , and
, and  combinations for which this does not happen. For
combinations for which this does not happen. For  , this means that the model is valid only for time
, this means that the model is valid only for time  , beyond which
, beyond which  , and for
, and for  , this time is
, this time is  .
.
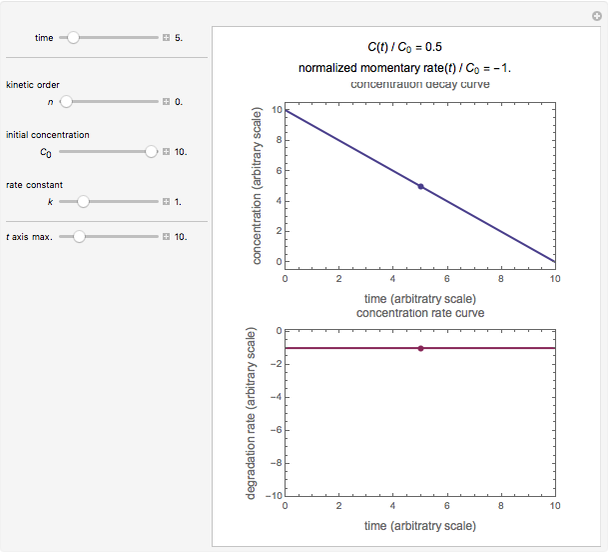
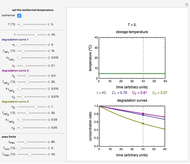
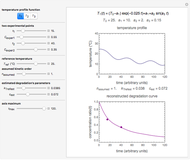
This Demonstration lets you generate degradation curves for any kinetic order  in the range of 0 to 2.5 and various combinations of
in the range of 0 to 2.5 and various combinations of  and
and  by identifying the case and using the relevant formula. You can vary all three parameters
by identifying the case and using the relevant formula. You can vary all three parameters  ,
,  , and
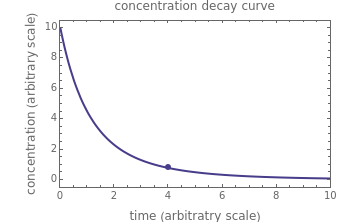
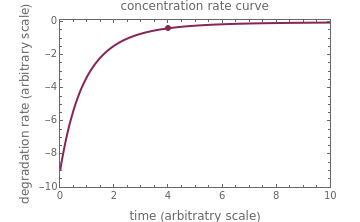
, and  , as well as the actual time and process duration. The graphic display shows the concentration decay curve (top) and corresponding degradation rate versus time curve (bottom). Also shown are the numerical values of the momentary decay ratio,
, as well as the actual time and process duration. The graphic display shows the concentration decay curve (top) and corresponding degradation rate versus time curve (bottom). Also shown are the numerical values of the momentary decay ratio,  , and the decay rate,
, and the decay rate,  , which correspond to the moving dots on the concentration and rate versus time plots.
, which correspond to the moving dots on the concentration and rate versus time plots.
The purpose of this Demonstration is to illustrate the concept of a reaction’s kinetic order. Therefore, not all possible decay rate curves represent an actual physical disintegration or degradation process.
Permanent Citation