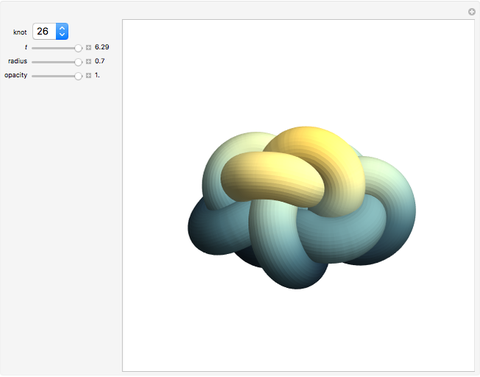
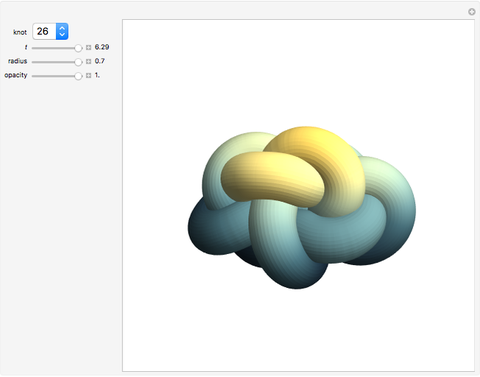
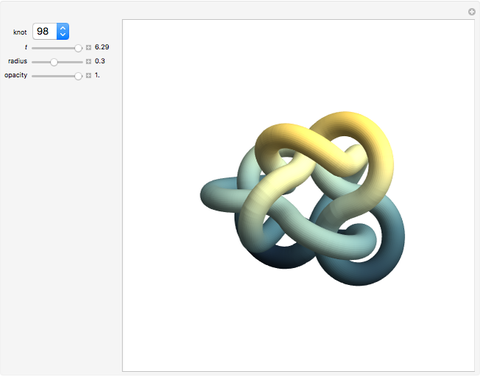
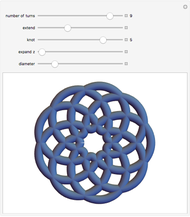
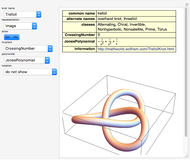
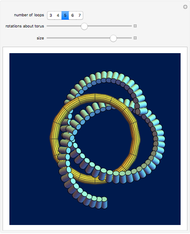
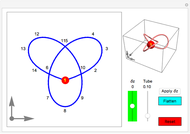
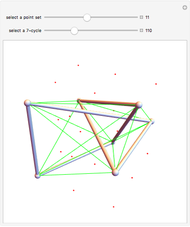
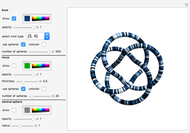
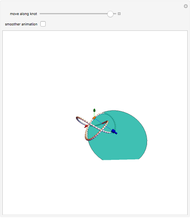
A knot is a closed-space curve that does not intersect itself—think of shoelaces, neckties, and ropes with their ends joined. Formally, knot theory is a branch of topology; there a more formal definition is that a knot is a closed piecewise linear curve in three-dimensional Euclidean space 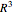. One important objective is to decide if two mathematical knots are equivalent, in the sense that one knot can be continuously transformed into the other without cuts or self-crossings. Knots have been cataloged according their number of crossings. The number of different knots grows rapidly as the number of crossings increases. Knot theory has applications mainly in statistical mechanics and quantum field theory.