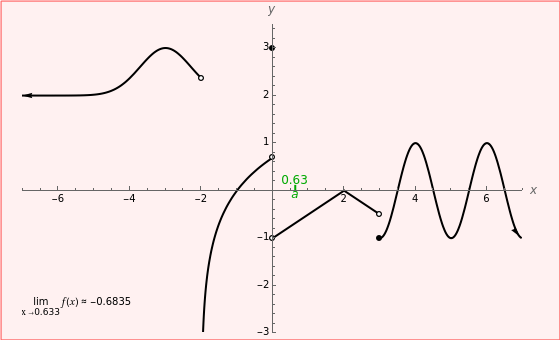
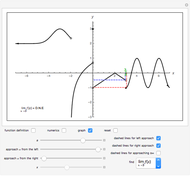
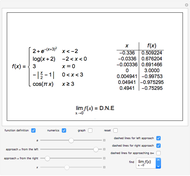
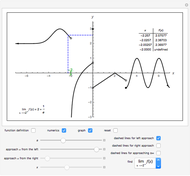
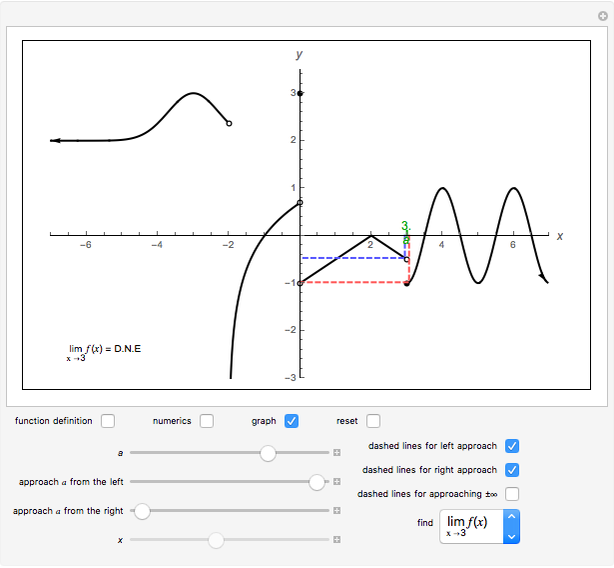
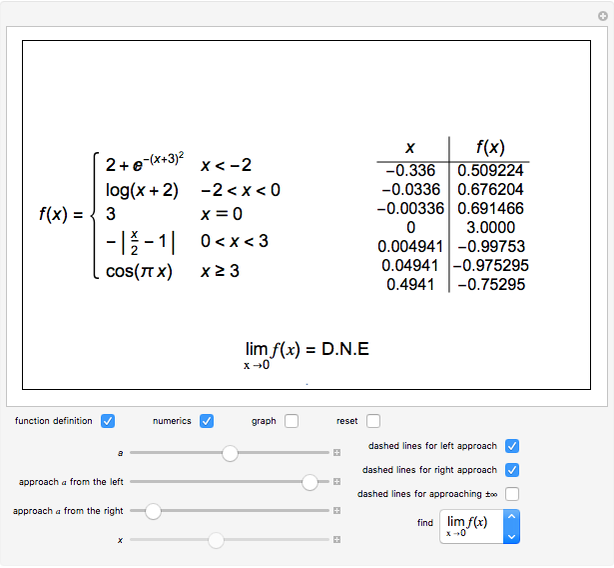
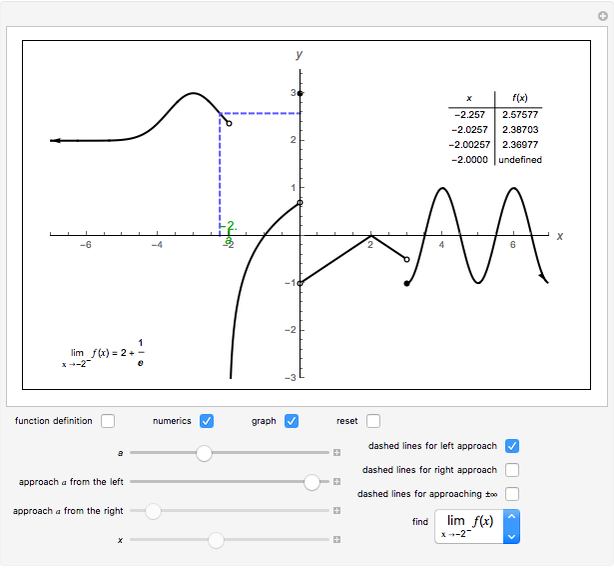
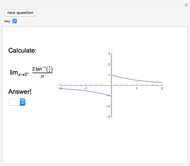
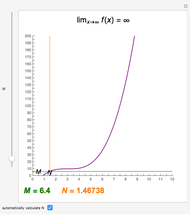
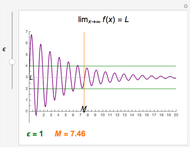
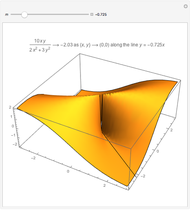
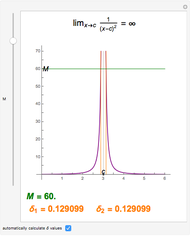
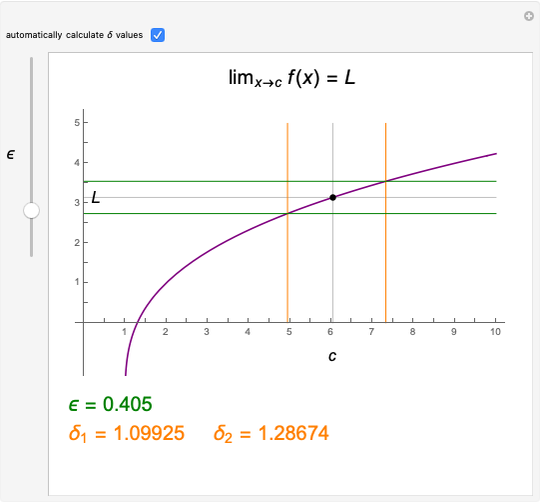
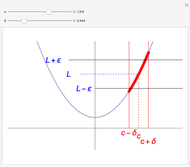
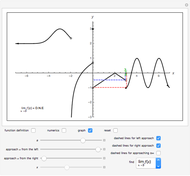
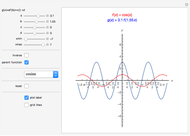
Limits are used to describe the behavior of a function near but not necessarily at particular values of the function's argument or as the argument tends to plus or minus infinity. Limits arise in fundamental concepts of calculus and analysis. In particular, limits help define continuity, differentiability, integrability, sequences, series, and infinite sums and products. Limits can be explored graphically and numerically, but in practice these tools serve as approximations to the exact analytic limit. This Demonstration explores limits at a point, one-sided limits, and limits at plus or minus infinity. If decimals are used for a, the limit will be numerical which may or may not agree with the analytic solution. You can input the exact value of a (not in decimal form) into the controls directly by clicking on the plus sign next to the slider control for a. The result will be consistent with the analytic solution.