Linear Equations: Row and Column View

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
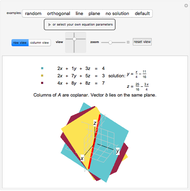
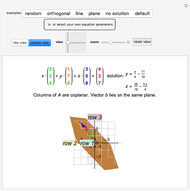
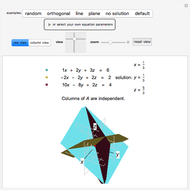
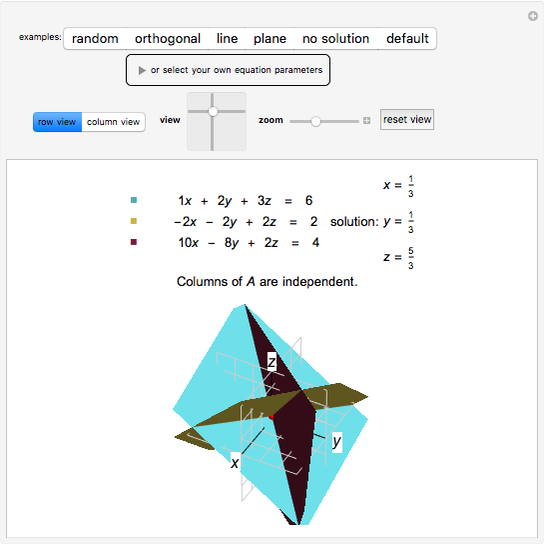
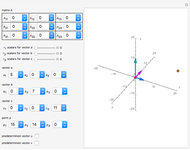
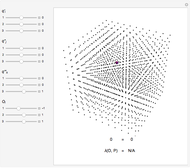
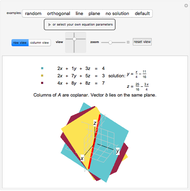
The fundamental question in linear algebra is determining if solutions exist for a set of linear equations  . There are two ways to look at this problem. The first is the row view—treating the problem as finding the intersection of a set of planes defined by the equations. The second way is the column view—treating the problem as finding the solution of a linear combination of the column vectors of
. There are two ways to look at this problem. The first is the row view—treating the problem as finding the intersection of a set of planes defined by the equations. The second way is the column view—treating the problem as finding the solution of a linear combination of the column vectors of  . This Demonstration illustrates the problem from either viewpoint.
. This Demonstration illustrates the problem from either viewpoint.
Contributed by: George V. Woodrow III (March 2011)
Inspired by: Klaus Michelsen
Open content licensed under CC BY-NC-SA
Snapshots
Details
This Demonstration was created to accompany the first chapters in G. Strang, Introduction to Linear Algebra, 3rd ed., Wellesley, MA: Wellesley–Cambridge Press, 2003.
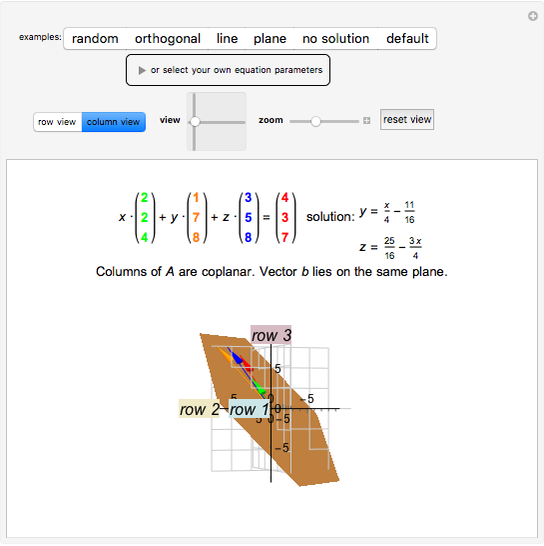
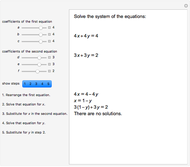
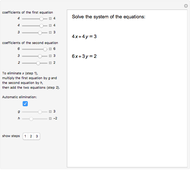
You can select examples of different types of systems of linear equations, or use the opener view and enter your own coefficients for the equations.
In the row interpretation, any solution appears in red. If there is a single solution, the point is shown by a red dot. If the planes intersect on a common line, the solution is shown as a red line. If the equations are coplanar, the solution is shown as a red plane.
In each case, look at the column view to see what the corresponding column vectors of the matrix  look like. If these column vectors lie on the same plane, the plane is shown in brown, and there is a solution only if the vector
look like. If these column vectors lie on the same plane, the plane is shown in brown, and there is a solution only if the vector  lies on the same plane. If the column vectors are collinear, the line is shown as a dashed brown line, and there is a solution only if b lies on the same line.
lies on the same plane. If the column vectors are collinear, the line is shown as a dashed brown line, and there is a solution only if b lies on the same line.
You can change the coefficients for the equations to see what happens when you have different types of solutions.
Permanent Citation