Moduli Space of Shapes of a Tetrahedron with Faces of Equal Area

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
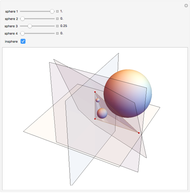
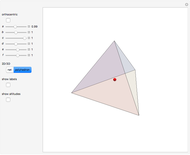
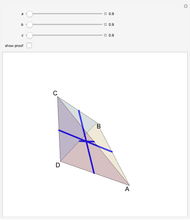
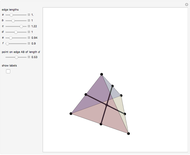
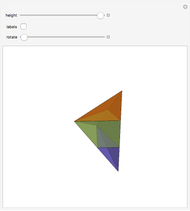
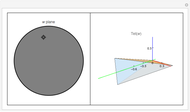
The space of shapes of a tetrahedron with fixed face areas is naturally a symplectic manifold of real dimension two. This symplectic manifold turns out to be a Kahler manifold and can be parametrized by a single complex coordinate 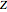 given by the cross ratio of four complex numbers obtained by stereographically projecting the unit face normals onto the complex plane. This Demonstration illustrates how this works in the simplest case of a tetrahedron
given by the cross ratio of four complex numbers obtained by stereographically projecting the unit face normals onto the complex plane. This Demonstration illustrates how this works in the simplest case of a tetrahedron 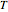 whose four face areas are equal. For convenience, the cross-ratio coordinate
whose four face areas are equal. For convenience, the cross-ratio coordinate 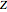 is shifted and rescaled to
is shifted and rescaled to 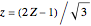 so that the regular tetrahedron corresponds to
so that the regular tetrahedron corresponds to 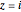 , in which case the upper half-plane is mapped conformally into the unit disc
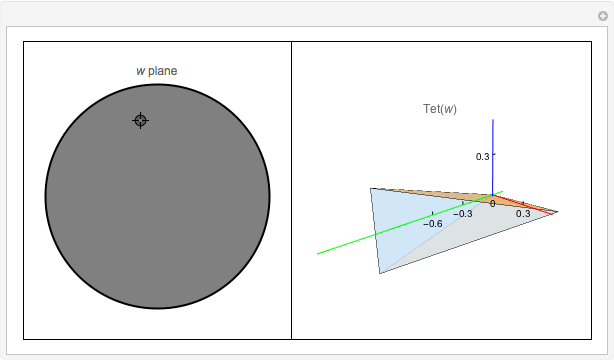
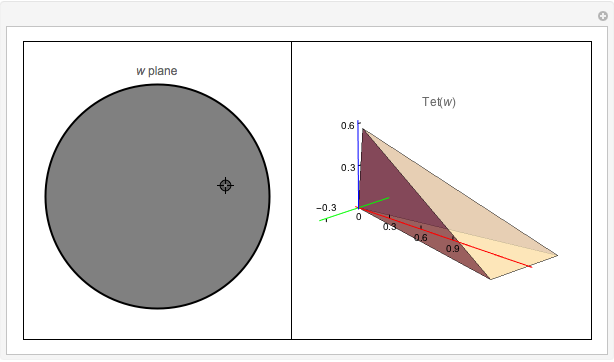
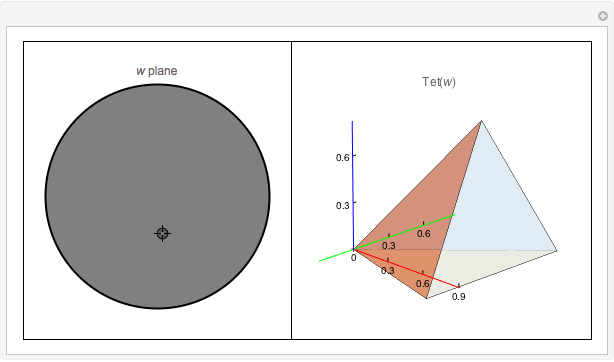
, in which case the upper half-plane is mapped conformally into the unit disc 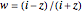 . The equi-area tetrahedron
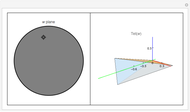
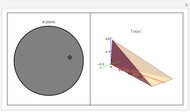
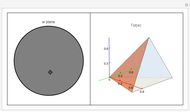
. The equi-area tetrahedron  is then drawn as a function of the unit disc coordinate
is then drawn as a function of the unit disc coordinate 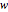 .
.
Contributed by: Kirill Krasnov (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
L. Freidel, K. Krasnov, and E. R. Livine, "Holomorphic Factorization for a Quantum Tetrahedron".