Newton-Simpson's Formula for the Volume of a Prismatoid

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
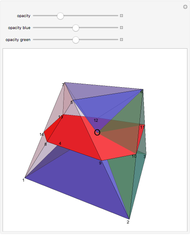
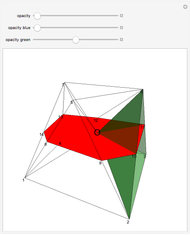
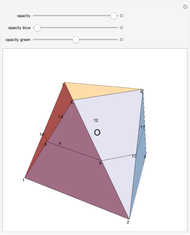
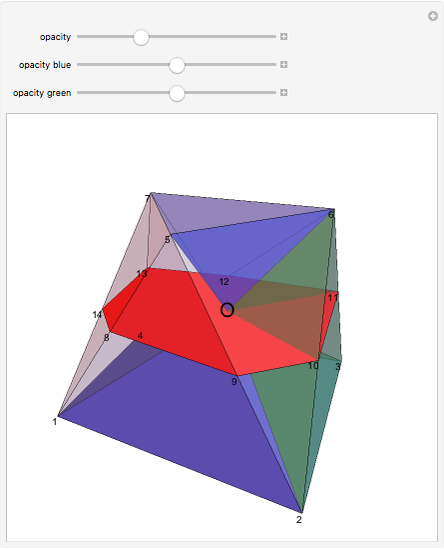
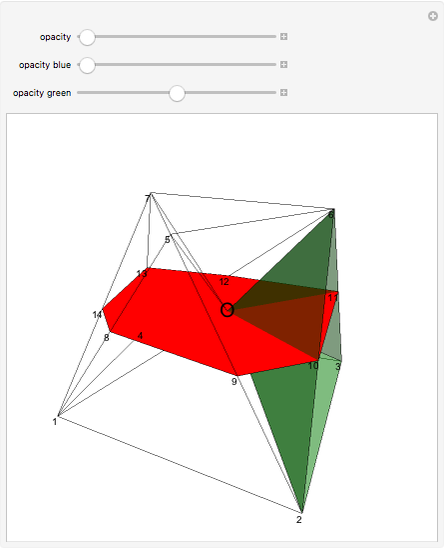
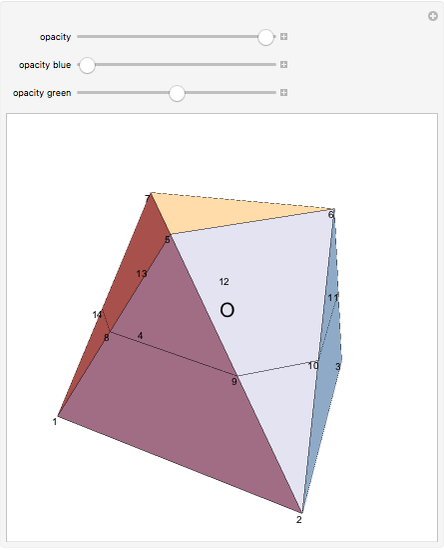
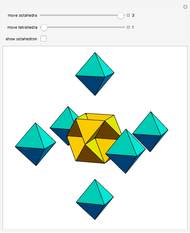
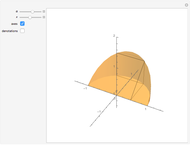
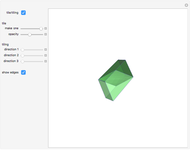
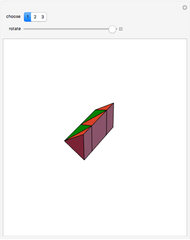
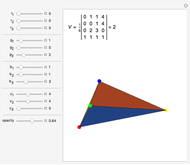
A prismatoid is the convex hull of two parallel convex polygons, a base 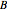 and a top
and a top 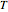 with areas
with areas 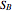 and
and 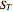 . Suppose the height of the solid is
. Suppose the height of the solid is  . Make a cross section
. Make a cross section 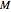 at height
at height 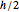 , let the point O be on
, let the point O be on 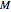 , and let the area of
, and let the area of 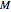 be
be 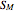 . The sum of the volumes of the two blue pyramids, the first with apex O and base
. The sum of the volumes of the two blue pyramids, the first with apex O and base 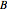 and the other with apex O and base
and the other with apex O and base 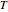 , is
, is 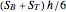 . A green pyramid
. A green pyramid 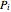 with apex O and a side face has volume equal to four times its upper part (the tetrahedron with vertices O, 12, 11, 6). But the volume of this tetrahedron is
with apex O and a side face has volume equal to four times its upper part (the tetrahedron with vertices O, 12, 11, 6). But the volume of this tetrahedron is 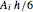 , where
, where 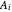 is the area of
is the area of 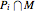 . The sum of all such areas
. The sum of all such areas 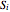 is
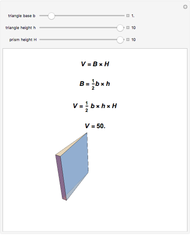
is 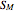 . So the volume of the prismatoid is
. So the volume of the prismatoid is 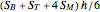 .
.
Contributed by: Izidor Hafner (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
detailSectionParagraphPermanent Citation
"Newton-Simpson's Formula for the Volume of a Prismatoid"
http://demonstrations.wolfram.com/NewtonSimpsonsFormulaForTheVolumeOfAPrismatoid/
Wolfram Demonstrations Project
Published: March 7 2011