Details

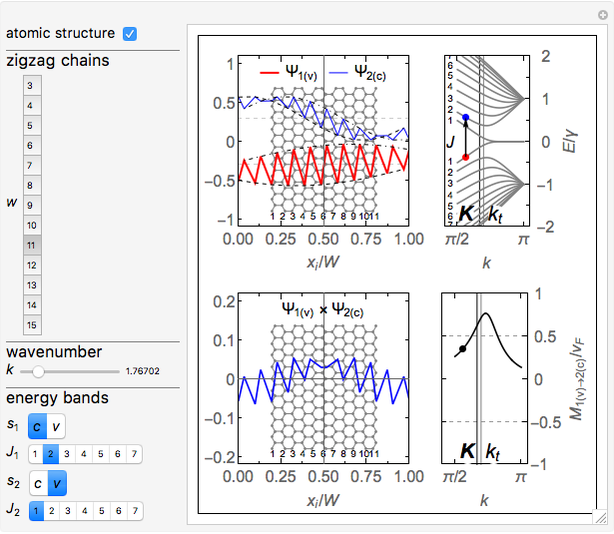
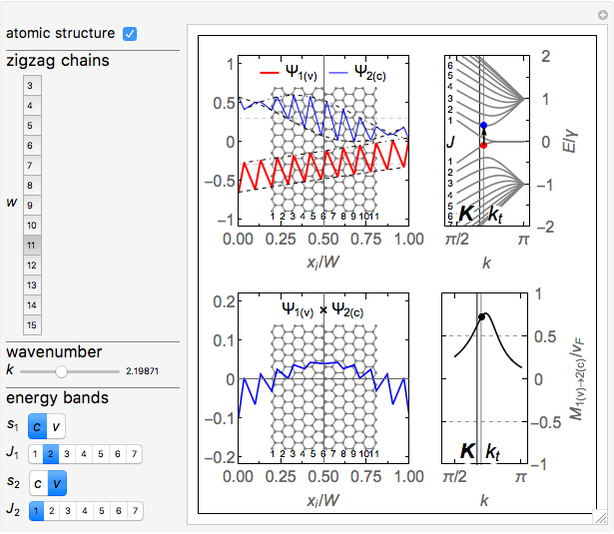
This Demonstration is based on the work in [1], which is an analytical extension of the work in [2]. All the notations are adopted from there. In particular, the energy bands of the ribbon are labeled by 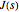, where 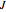labels the band number and , the band type. Therefore, 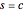stands for the conduction band, 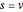for the valence band.

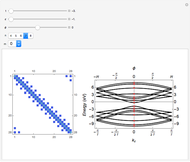
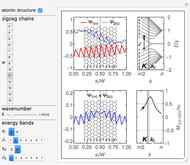
The normalized wavefunctions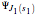 (blue) and 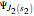(red) are plotted in the upper-left as functions of the normalized transverse coordinate 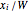, where 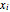is the coordinate of the 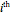atom in the ribbon unit cell and 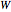is the width of the ribbon. The coordinates of the atoms from the A and B sublattices forming a hexagonal structure of a zigzag graphene nanoribbon (ZGNR) are 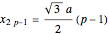and 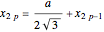, where 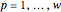, with 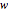being the number of zigzag chains specifying the width of the ribbon. The number of zigzag chains is 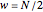, with 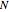being the number of carbon atoms in the zigzag ribbon unit cell. Then, the ribbon width is 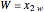. Thus, the ribbon with a certain width can be labeled as 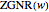. 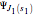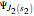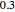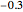The wavefunctions (blue) and (red) are offset for clarity by and , respectively.

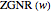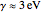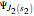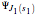The energy bands of a chosen presented in the upper-right band structure plot are normalized by the hopping integral . The red and blue points in the band structure plot represent the states with wavefunctions and , respectively.

The lower-left plot shows the 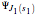and 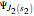wavefunctions overlapping for chosen 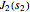and .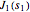

The optical matrix elements 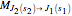for a chosen transition 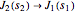are presented in the lower right plot as functions of the electron wave number . These matrix elements are velocity matrix elements normalized by the Fermi velocity of electrons in graphene, 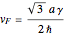, where 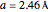is the graphene lattice constant, is the hopping integral, and is the reduced Planck's constant. The black point denotes the matrix element value for the transition depicted in the band structure plot.

The point 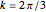corresponding to the Dirac point in graphene is marked by the vertical line labeled as 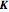in the energy band and matrix element plots. Similar marking by the vertical line is used for the transition point 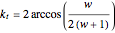, where the bulk states meet the edge states in the subbands 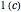and .

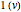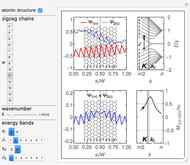
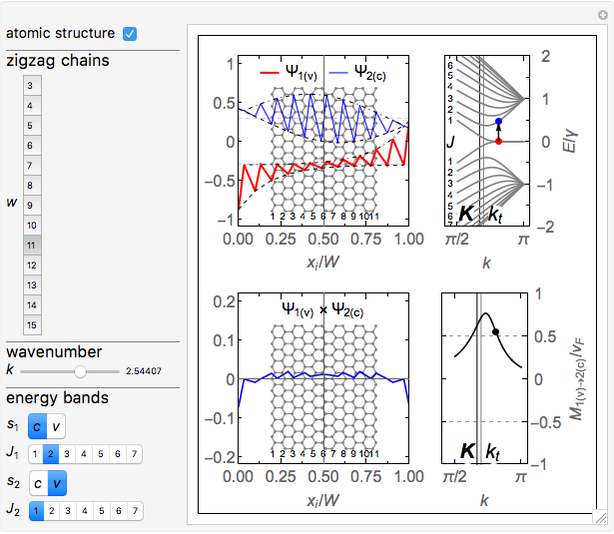
Snapshot 1: the wavefunction (red) of the bulk state in the subband 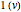of

Snapshot 2: the wavefunction (red) of at the transition point 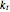, where the bulk states meet the edge states in the subband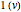

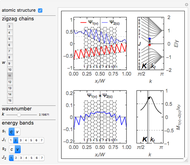
Snapshot 3: the wavefunction (red) of the subband 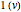edge states localized at the ribbon edges for

Snapshot 4: forbidden transition between valence and conduction subbands of

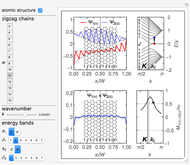
Snapshot 5: allowed transition between valence and conduction subbands of

Snapshot 6: forbidden transition between conduction subbands of

Snapshot 7: allowed transition between conduction subbands of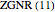

Snapshots 1–3 show the transformation of the electron wavefunction (red) as one moves from the bulk to the edge states within the 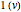subband. Snapshots 4 and 5 demonstrate the odd selection rule 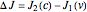for allowed transitions between the conduction and valence subbands. Snapshots 6 and 7 demonstrate the even selection rule 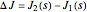for allowed transitions between the conduction (valence) subbands only.

References

[1] V. A. Saroka, M. V. Shuba and M. E. Portnoi, "Optical Selection Rules of Zigzag Graphene Nanoribbons," Physical Review B, 95(15), 2017 155438. doi:10.1103/PhysRevB.95.155438.

[2] H. C. Chung, M. H. Lee, C. P. Chang and M. F. Lin, "Exploration of Edge-Dependent Optical Selection Rules for Graphene Nanoribbons," Optics Express, 19(23), 2011 pp. 23350–23363. doi:10.1364/OE.19.023350.