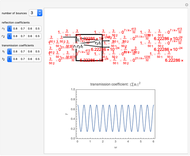
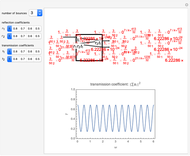
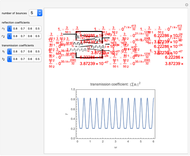
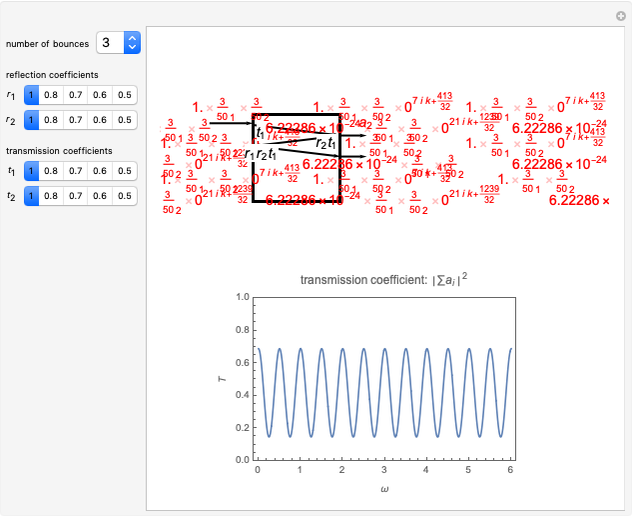
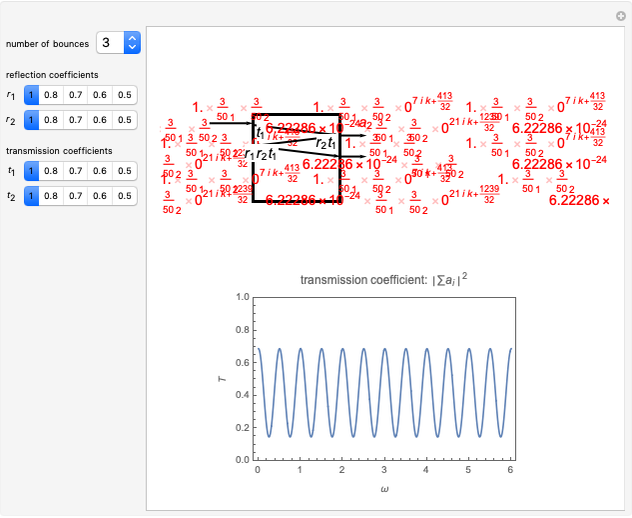
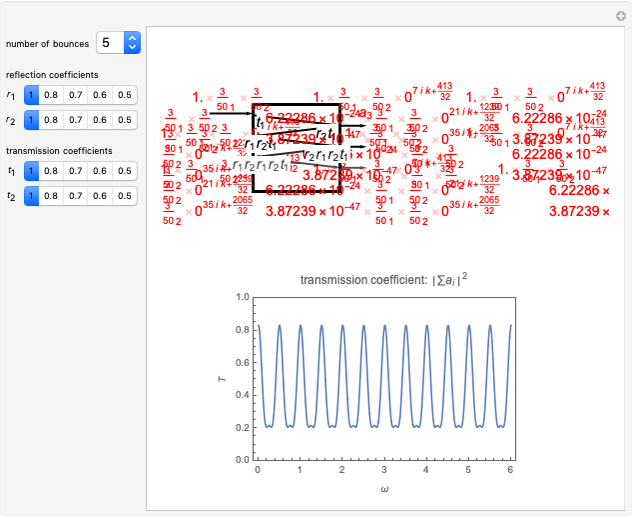
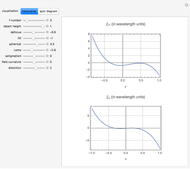
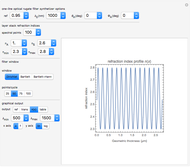
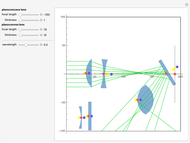
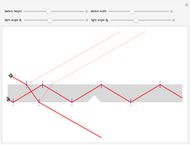
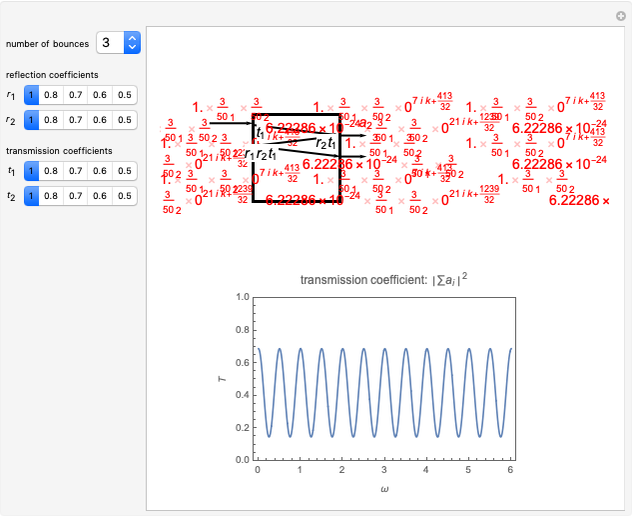
Consider a ray of light bouncing back and forth between two reflective surfaces with reflection and transmission coefficients . As the light (represented by a complex exponential with wave vector ) travels a length through the cavity, the amplitude decreases with an optical loss factor . After bounces, the amplitude is multiplied by a factor , while the reflection coefficients , are multiplied by this factor times. The phase after traveling 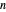lengths is equal to . The total transmission is given by the sum of rays . This is a geometric series, so , where . Finally, we arrive at the total transmission . From the plot, there will be resonant frequencies in the limit as .