Wolfram Demonstrations Project

13,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

Relating the Great Rhombicosidodecahedron to Several Other Polyhedra

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

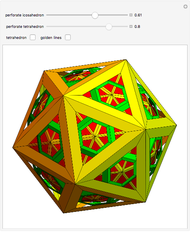
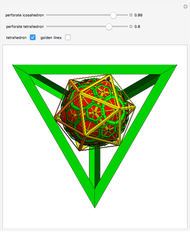
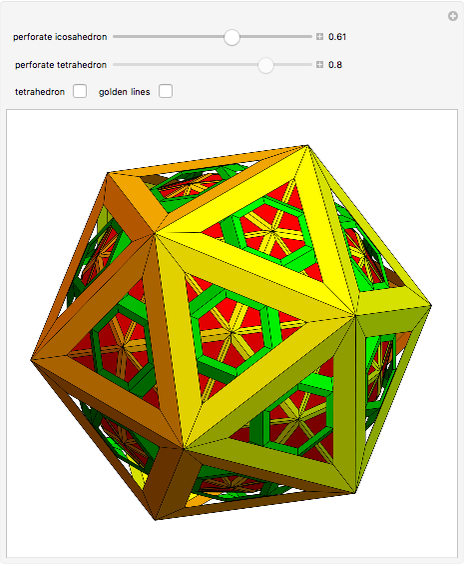
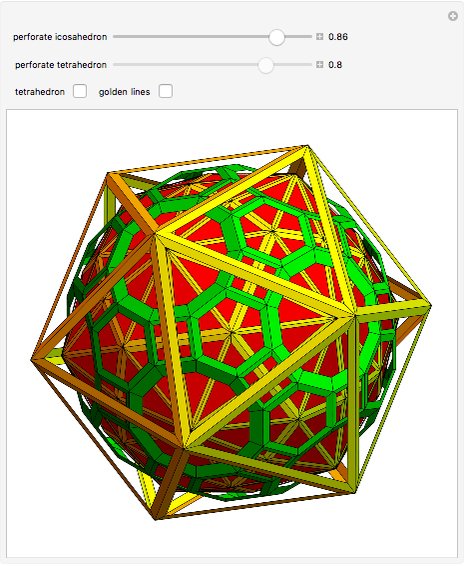
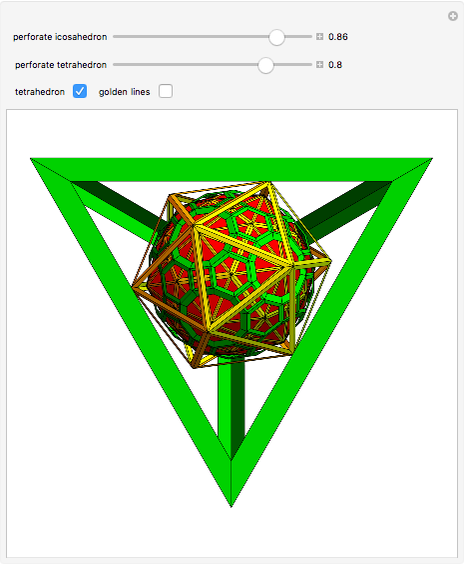
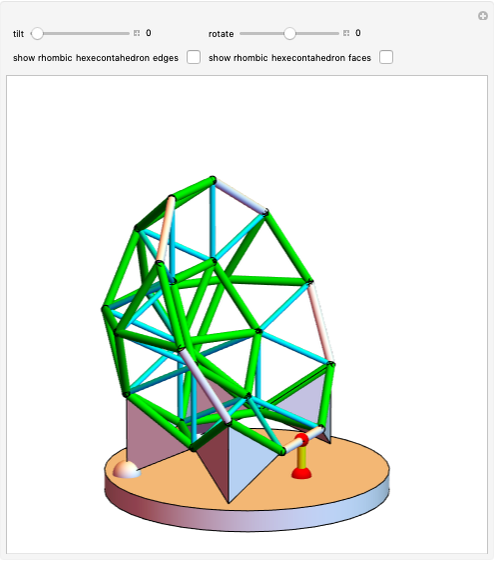
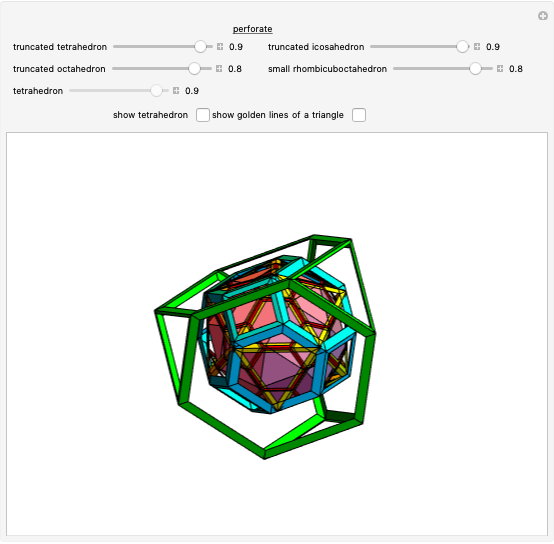
This Demonstration shows the relationship of a great rhombicosidodecahedron (an Archimedean solid) with its dual disdyakis triacontahedron (a Catalan solid), a tetrahedron, an icosahedron and the golden lines of a triangle.

Contributed by: Sándor Kabai (October 2019)
Open content licensed under CC BY-NC-SA

Details

In [1], Pugh points out that the Archimedean solids are all capable of being circumscribed by a regular tetrahedron so that four of their faces lie on the faces of that tetrahedron.

Reference

[1] A. Pugh, Polyhedra: A Visual Approach, Berkeley: University of California Press, 1976 p. 25.

Snapshots

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send