Rotating Disk Electrode: Distribution of Centers with Spillover Effect

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
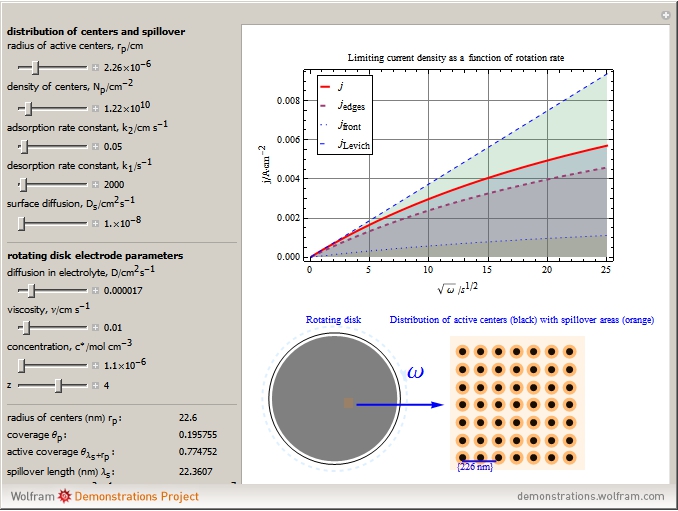
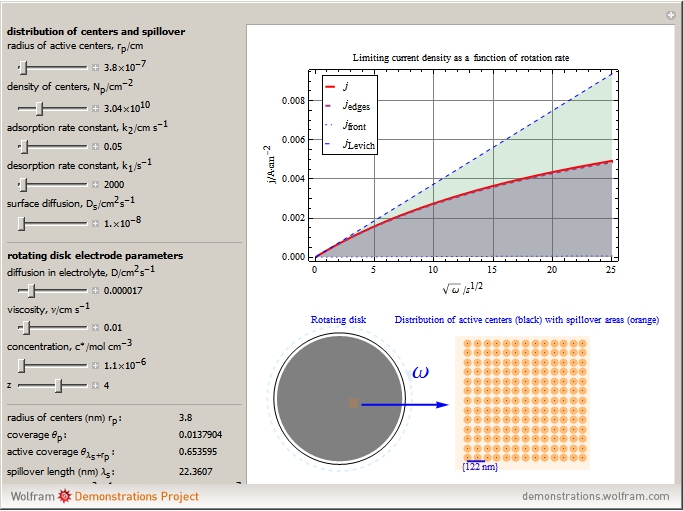
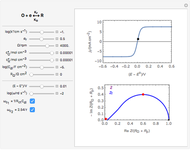
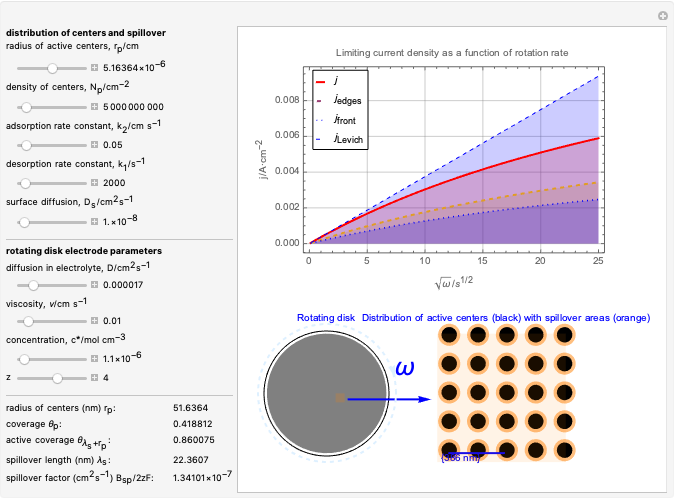
This Demonstration calculates the electrochemical current from an electrode consisting of a distribution of active centers embedded in a rotating disk in contact with liquid electrolyte. The current has two components, one due to the reacting species dissolved in the electrolyte and arriving to the front surface of the centers (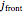 ), and the other due to the species adsorbed on inactive areas and arriving to the edges of the centers by "spillover" effect (
), and the other due to the species adsorbed on inactive areas and arriving to the edges of the centers by "spillover" effect ( ). Both contributions are calculated to yield the total current (
). Both contributions are calculated to yield the total current ( ). The result is plotted as a function of the rotation rate (
). The result is plotted as a function of the rotation rate ( ), and can be compared with the current response from a continuous disk electrode calculated using the Levich equation (
), and can be compared with the current response from a continuous disk electrode calculated using the Levich equation ( ).
).
Contributed by: Antonio M. Chaparro (January 2012)
Open content licensed under CC BY-NC-SA
Snapshots
Details
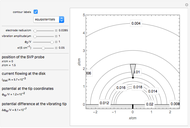
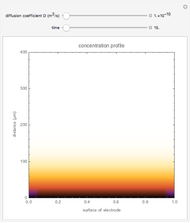
The rotating disk electrode technique is used for the study of electrochemical reactions on macroscopic electrode surfaces in contact with a liquid electrolyte. The current response under transport limiting conditions is well characterized from the solution of the convective-diffusion equation and can be used to measure kinetic parameters of the electrochemical reaction [1]. However, for electrodes composed of a distribution of micro-nano centers embedded in an inactive surface, the traditional theory for a continuous electrode may not work, since the transport mechanisms of electroactive species toward the centers are different. In particular, a model has been proposed that assumes two different transport types, one due to dissolved species arriving at the front surface of the centers and the other due to adsorbed species on the support and arriving at the edges of the centers by surface diffusion [2]. This second type is usually called the "spillover" effect and is characterized by an interaction constant ( ), desorption constant (
), desorption constant ( ), and surface diffusion coefficient (
), and surface diffusion coefficient ( ). A spillover area is generated around each center characterized by a length,
). A spillover area is generated around each center characterized by a length,  . The total current from the dispersion of active centers can be calculated as a function of the spillover parameters, the size of the centers (
. The total current from the dispersion of active centers can be calculated as a function of the spillover parameters, the size of the centers ( ), and the density (
), and the density ( ).
).
References
[1] A. J. Bard and L. R. Faulkner, Electrochemical Methods: Fundamentals and Applications, New York: John Wiley & Sons, 1980.
[2] A. M. Chaparro, "Study of Spillover Effects with the Rotating Disk Electrode," Electrochimica Acta, 58(30), 2011 pp. 691–698. doi:10.1016/j.electacta.2011.10.013.
Permanent Citation