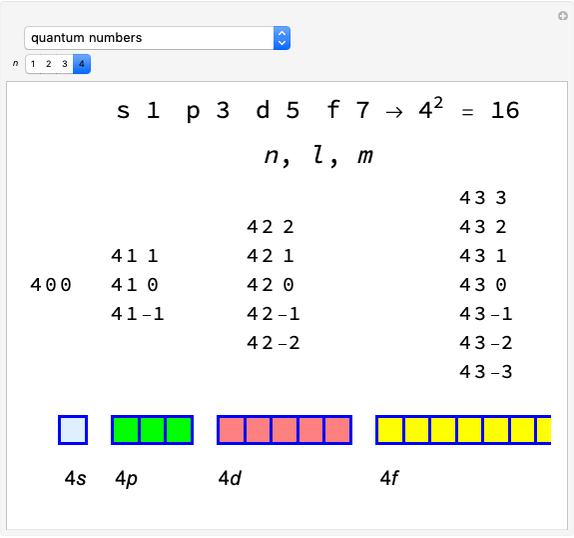
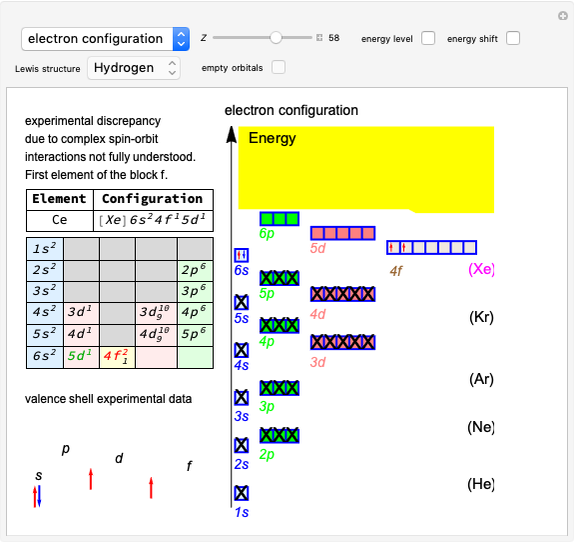
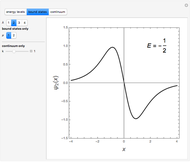
Schrödinger Equation for a Dirac Bubble Potential

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
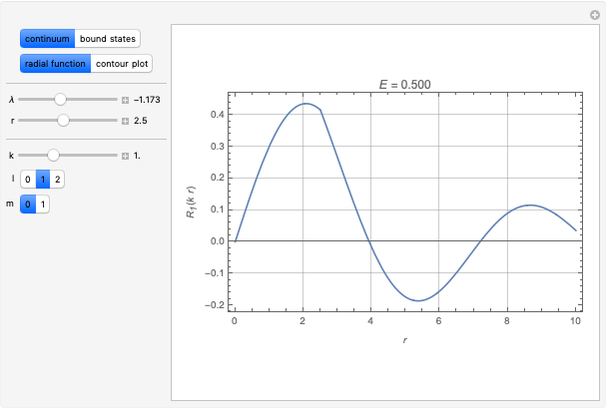
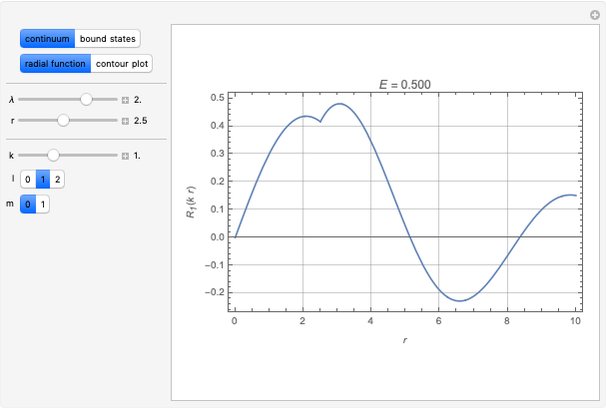
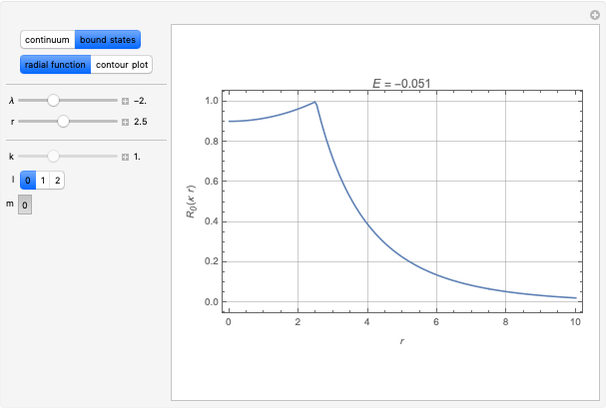
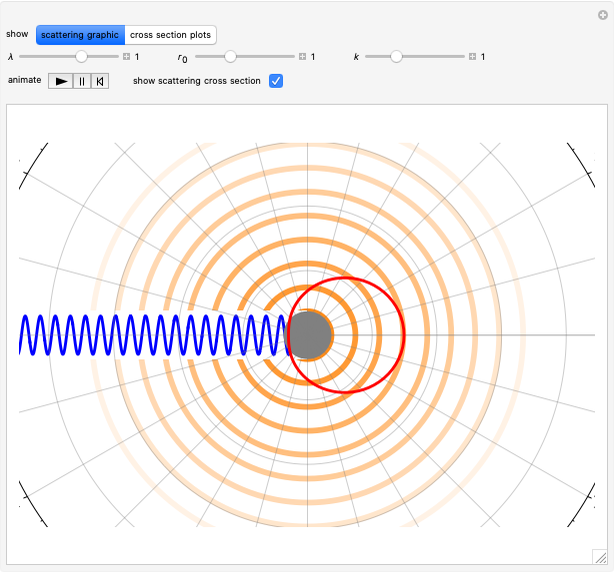
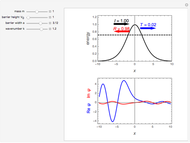
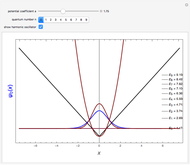
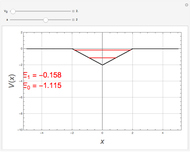
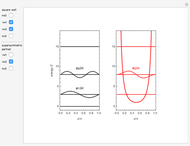
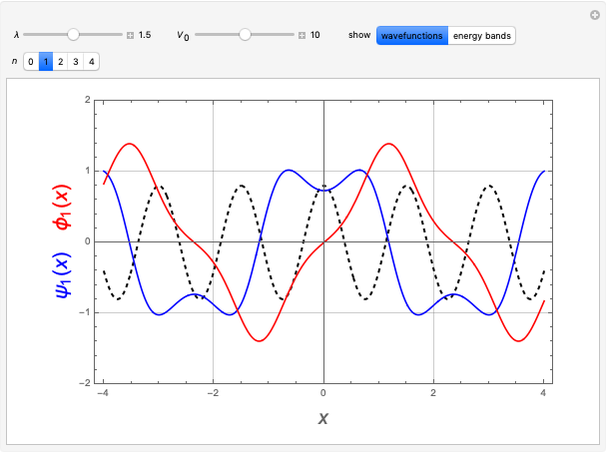
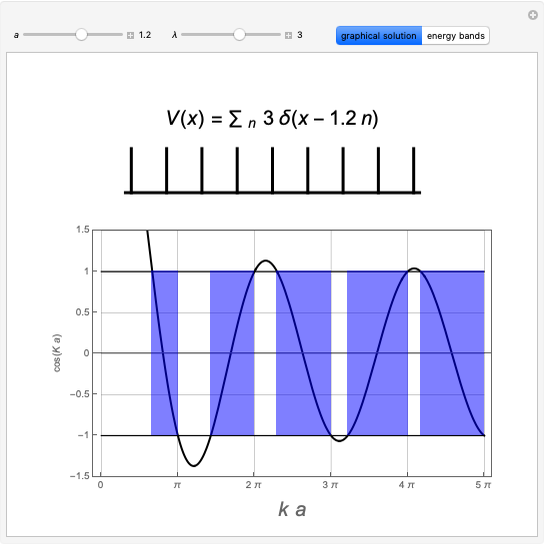
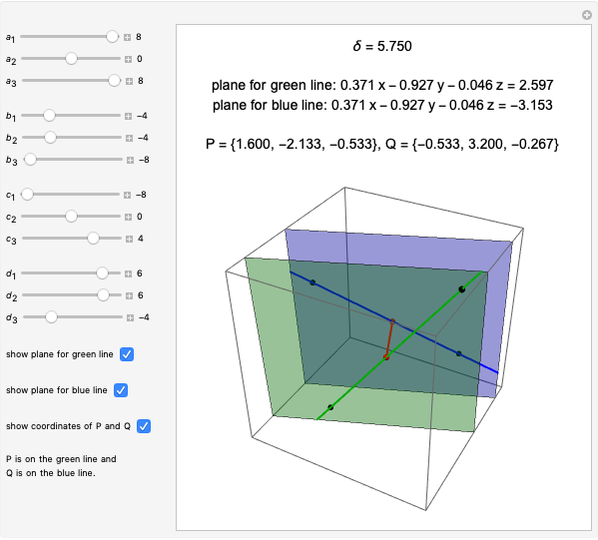
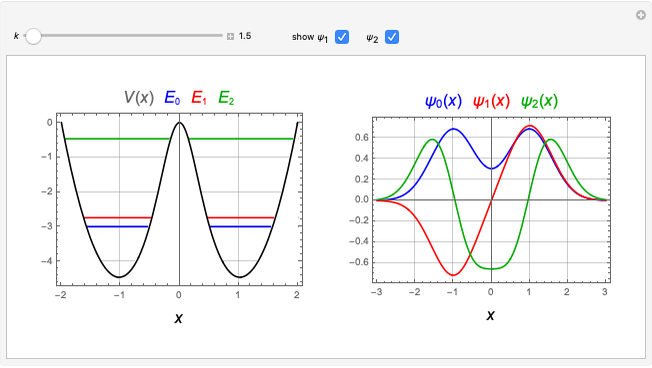
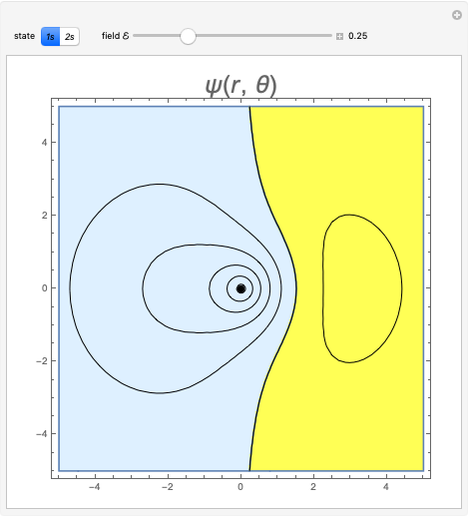
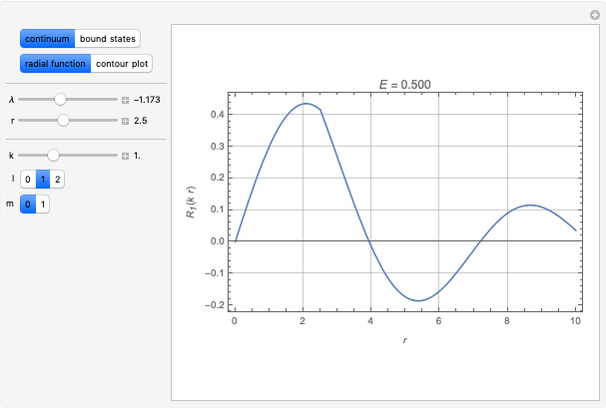
The Schrödinger equation has been solved in closed form for about 20 quantum-mechanical problems. This Demonstration describes one such example published some time ago. A particle moves in a potential that is zero everywhere except on a spherical bubble of radius  , drawn as a red circle in the contour plots. This result has been applied to model the buckminsterfullerene molecule
, drawn as a red circle in the contour plots. This result has been applied to model the buckminsterfullerene molecule  and also to approximate the interatomic potential in the helium van der Waals dimer
and also to approximate the interatomic potential in the helium van der Waals dimer  .
.
Contributed by: S. M. Blinder (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
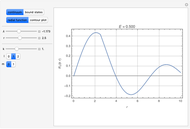
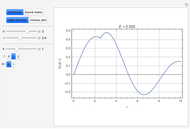
Snapshot 1: radial function of a continuum state
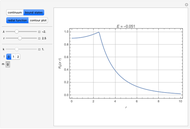
Snapshot 2: radial function for a bound state
Snapshot 3: contour plot of a continuum
Reference
[1] S. M. Blinder, "Schrödinger Equation for a Dirac Bubble Potential," Chemical Physics Letters, 64(3), 1979 pp. 485–486. doi:10.1016/0009-2614(79)80227-4.
Permanent Citation