Sensitive Dependence on Initial Conditions in a Simple Three-Body Problem

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
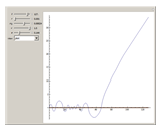
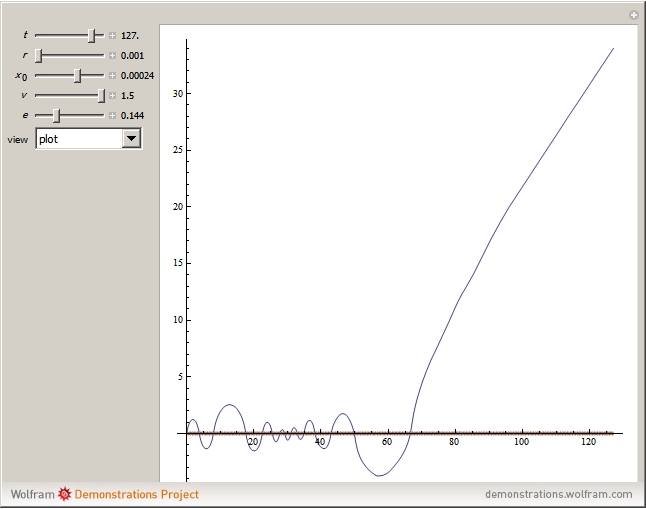
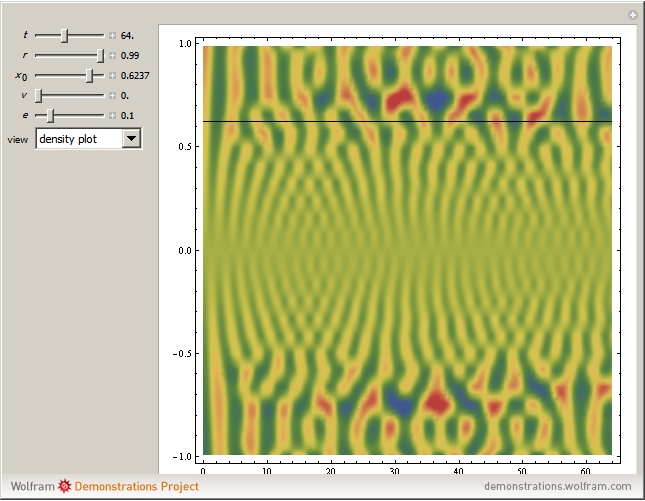
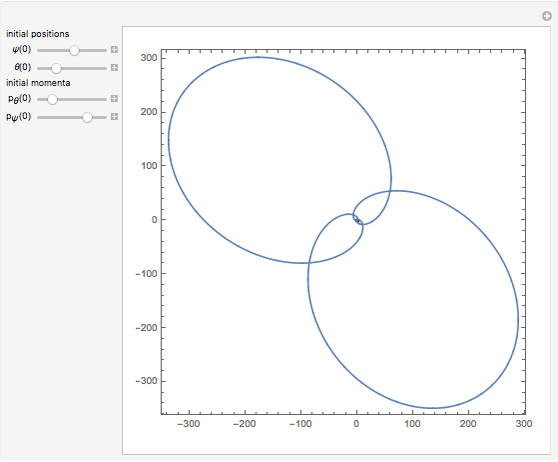
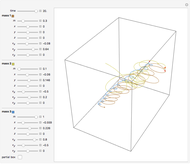
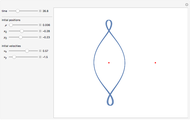
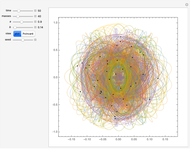
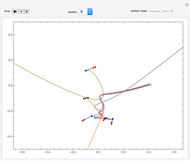
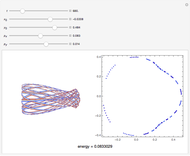
A planet moves under the gravitational influence of two stars of equal mass (very large compared with the planet), in orbits with eccentricity  around their center of gravity. The planet moves in a plane perpendicular to the plane of the stars, passing through their center of gravity. The differential equation is solved for initial position
around their center of gravity. The planet moves in a plane perpendicular to the plane of the stars, passing through their center of gravity. The differential equation is solved for initial position  in the range
in the range  and velocity
and velocity  . The system shows sensitive dependence on initial conditions, despite the simplicity of the equation of motion. This problem was studied by the Soviet mathematician Kirill Sitnikov.
. The system shows sensitive dependence on initial conditions, despite the simplicity of the equation of motion. This problem was studied by the Soviet mathematician Kirill Sitnikov.
Contributed by: Enrique Zeleny (January 2013)
Open content licensed under CC BY-NC-SA
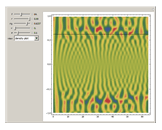
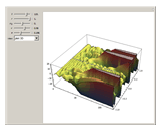
Snapshots
Details
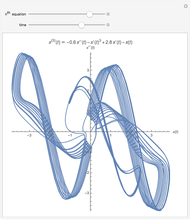
The differential equation describing the system is
 .
.
References
[1] K. Sitnikov, "The Existence of Oscillatory Motions in the Three-Body Problem," Doklady Akademii Nauk SSSR (Physics), 133, 1960 pp. 303–306.
[2] B. Casselman, "What Is the Length of a Year on Planet Sitnikov?" (Dec 26, 2012) www.ams.org/samplings/feature-column/fcarc-sitnikov.
Permanent Citation