Details

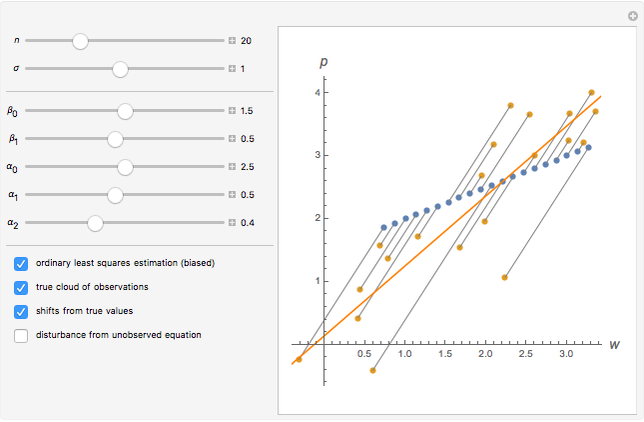
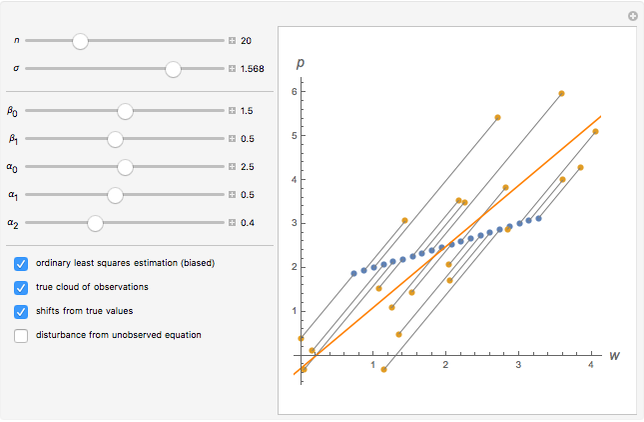
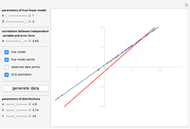
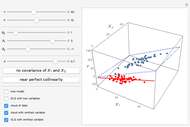
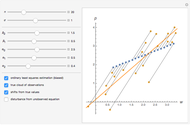
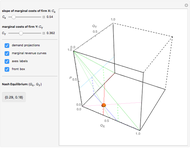
Consider a system of simultaneous equations where each observation gives one point as its solution and which is shifted by error terms. Even if there are many observations, the cloud of points does not allow us to understand the model. In order to identify the relationship between and (both endogenous variables), we need to have at least one exogenous variable ( in our case), so we simulate several values of . If there are no error terms and in the model, we would get the blue points that reflect the true relationship between and . If the error terms and do not suffer from endogeneity, we could use ordinary least squares to fit the model of the form

.

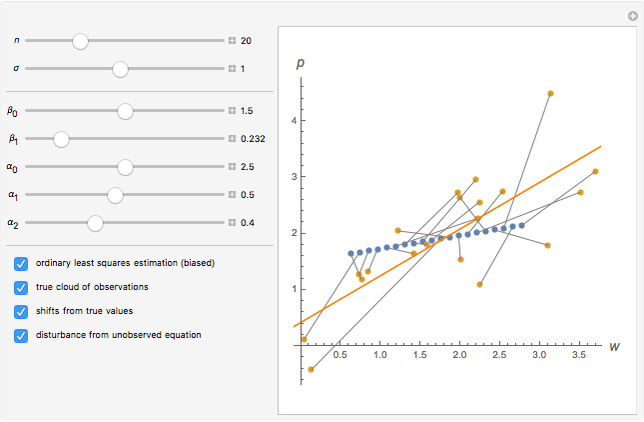
The problem is that such is not the case. To simplify matters and make the visualization more appealing, we keep the error term in the unobserved equation by default (the bottom checkbox changes this setting).

We may express algebraically in reduced form from the system of simultaneous equations and see that it depends on :

.

As a result, each observation shifts to the value of horizontally. At the very same time, shifts to the value of vertically. Because changes from observation to observation randomly, we see such a pattern on the plot:

.

We may divide the vertical to horizontal shift and find the slope of shifts, which is the same for all observations as cancels out. Indeed:

.

This effect is shown on the plot. Use the slider to see the shift effect. Use the checkboxes to turn on and off different plot elements and study their behavior.

In order to estimate the model, you can use either instrumental variable (IV) or control function (CF) approaches. For instance, can be regressed on , which is a natural instrument for this toy example. In practice, finding and substantiating relevant instruments is a challenging task.

References

[1] Wikipedia. "Endogeneity (Econometrics)." (Mar 30, 2018) en.wikipedia.org/wiki/Endogeneity_(econometrics).

[2] C. Dougherty, Introduction to Econometrics, 5th ed., Oxford, UK: Oxford University Press, 2016 pp. 345–350.