Solitons from the Korteweg-de Vries Equation

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
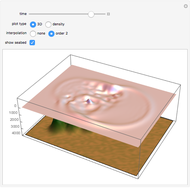
John Scott Russell, a Scottish naval engineer, reported in 1834 on his observation of a remarkable solitary water wave moving a considerable distance down a narrow channel. Korteweg and de Vries (1895) developed a theory to describe weakly nonlinear wave propagation in shallow water. The standard form of the Korteweg-de Vries (KdV) equation is usually written  (in some references with
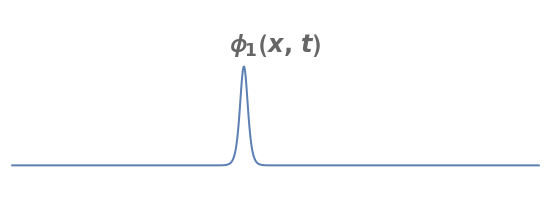
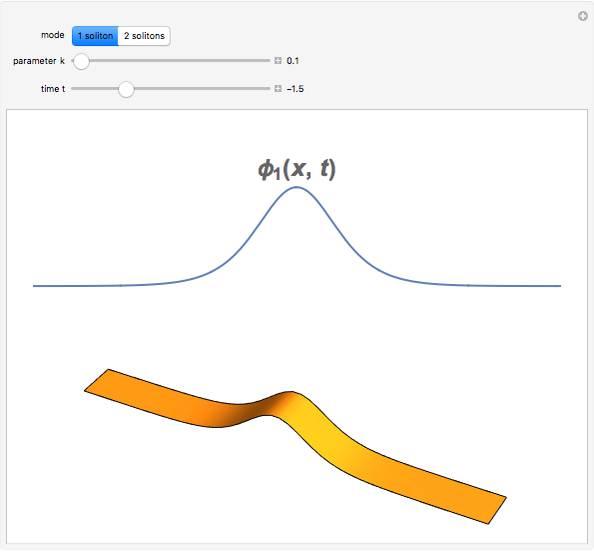
(in some references with  6). Kruskal and Zabusky (1965) discovered that the KdV equation admits analytic solutions representing what they called "solitons"—propagating pulses or solitary waves that maintain their shape and can pass through one another. These are evidently waves that behave like particles! Several detailed analyses suggest that the coherence of solitons can be attributed to a compensation of nonlinear and dispersive effects. A 1-soliton solution to the KdV equation can be written
6). Kruskal and Zabusky (1965) discovered that the KdV equation admits analytic solutions representing what they called "solitons"—propagating pulses or solitary waves that maintain their shape and can pass through one another. These are evidently waves that behave like particles! Several detailed analyses suggest that the coherence of solitons can be attributed to a compensation of nonlinear and dispersive effects. A 1-soliton solution to the KdV equation can be written  . This represents a wavepacket with amplitude
. This represents a wavepacket with amplitude  and wave velocity
and wave velocity  , depending on a parameter
, depending on a parameter  , one of the constants of integration.
, one of the constants of integration.
Contributed by: S. M. Blinder (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
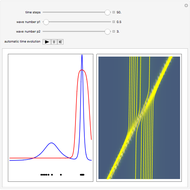
For nice animations of soliton propagation and collisions, use autorun with the time variable in slow motion.
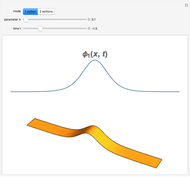
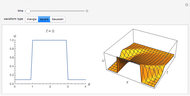
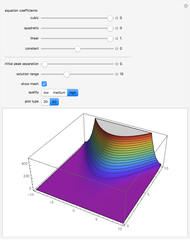
Snapshot 1. The shape and speed of a soliton depends on the parameter  .
.
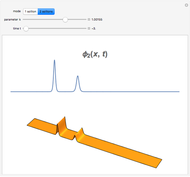
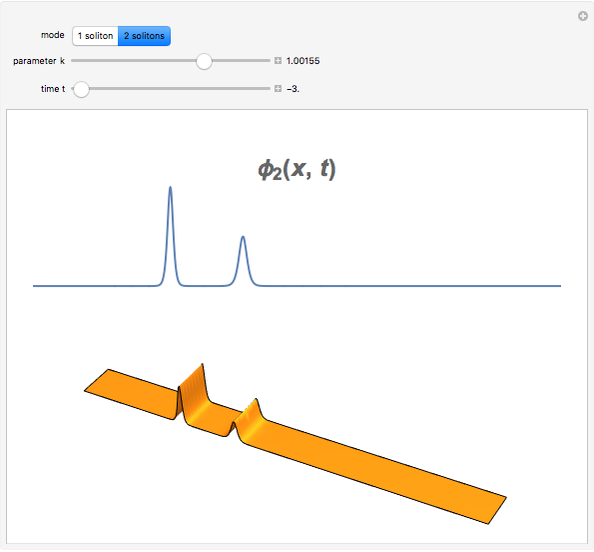
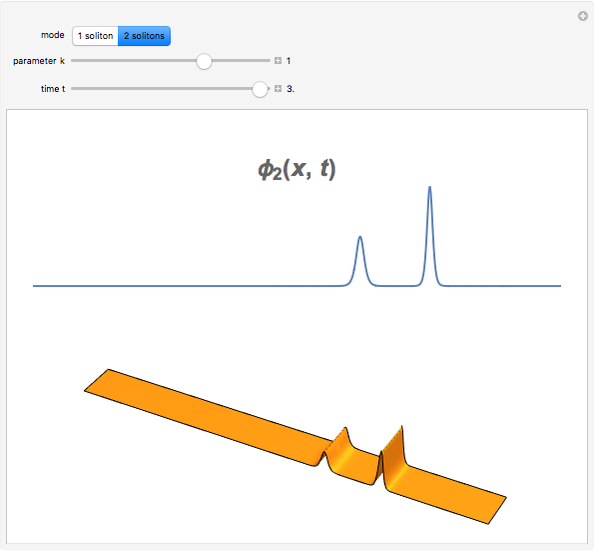
Snapshot 2. Two solitons moving to the right approach one another.
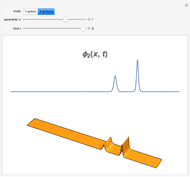
Snapshot 3. And the larger one apparently overtakes and passes the smaller one. Actually, they are exchanging shapes and speeds.
Permanent Citation