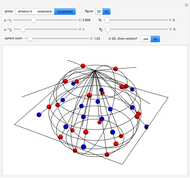
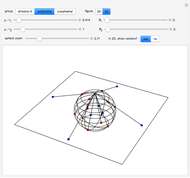
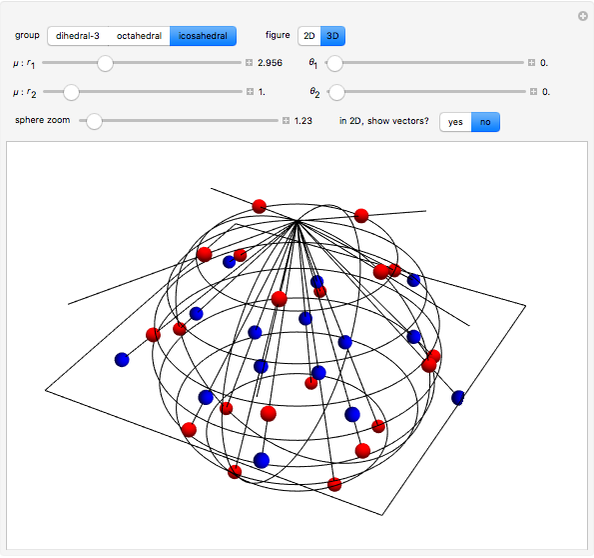
Stereographic Projection of Some Double Groups

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
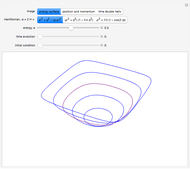
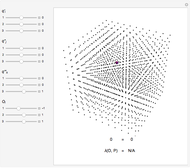
Stereographic projection provides geometric insight into the double cover  . Each rotation of the sphere corresponds to exactly two linear transformations of homogeneous coordinates
. Each rotation of the sphere corresponds to exactly two linear transformations of homogeneous coordinates  ,
,  . The projection remains a bijection because the Möbius transformation of a complex plane coordinate
. The projection remains a bijection because the Möbius transformation of a complex plane coordinate  retains only the relative sign of
retains only the relative sign of  ,
,  [1]. Taking a linear perspective, you can view points in the plane as the cosets of inversion by a
[1]. Taking a linear perspective, you can view points in the plane as the cosets of inversion by a  rotation. Plotting complex vectors
rotation. Plotting complex vectors  ,
,  off each plane coordinate
off each plane coordinate  reveals the hidden coset structure, which sometimes gets overlooked. The double groups, also called binary groups, contribute a foundational element in the analysis of quintic equations [2] and for quantum mechanics [3]. Furthermore, there is an aesthetic value in these dynamic images, in which variation of parameters appears to create a whirling dance of the points across the plane.
reveals the hidden coset structure, which sometimes gets overlooked. The double groups, also called binary groups, contribute a foundational element in the analysis of quintic equations [2] and for quantum mechanics [3]. Furthermore, there is an aesthetic value in these dynamic images, in which variation of parameters appears to create a whirling dance of the points across the plane.
Contributed by: Brad Klee (March 2016)
Open content licensed under CC BY-NC-SA
Snapshots
Details
A Möbius transformation takes a complex number  into another complex number
into another complex number  ,
,
 ,
,
where the  are complex. The equivalent linear transformation is
are complex. The equivalent linear transformation is
 ,
,
 .
.
Notice that inversion

transforms  without changing
without changing  .
.
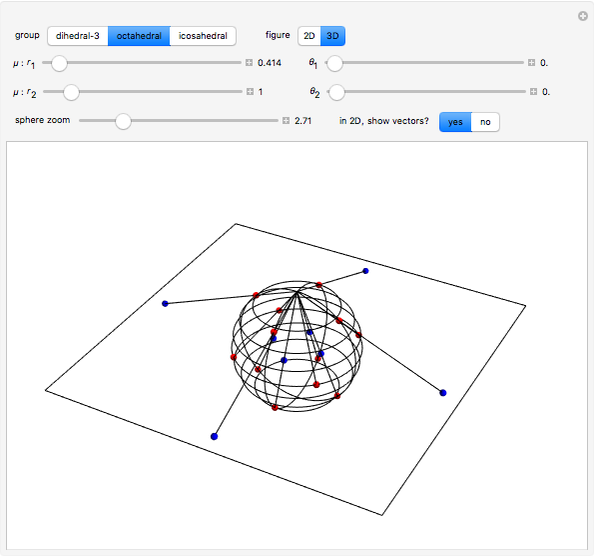
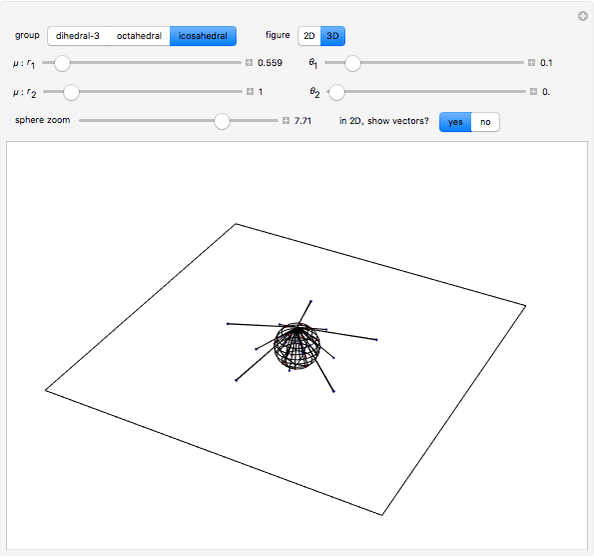
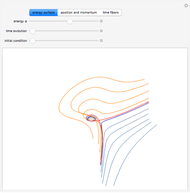
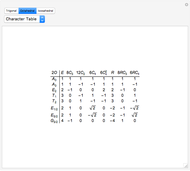
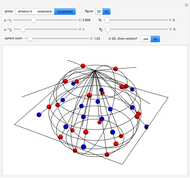
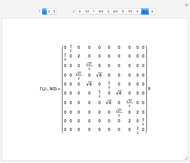
This Demonstration displays a two-dimensional representation of a finite rotational group  ,
,  , from the usual three-dimensional, real-space representation of
, from the usual three-dimensional, real-space representation of  . As shown by Klein [2], the two-dimensional representation always contains inversion, so there are two opposing vectors
. As shown by Klein [2], the two-dimensional representation always contains inversion, so there are two opposing vectors  plotted at each point
plotted at each point  .
.
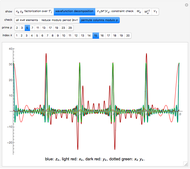
Starting with some point  , we compute a set of points
, we compute a set of points  and the corresponding
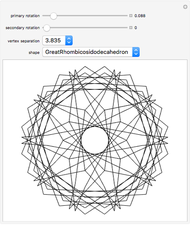
and the corresponding  . These points lift to the surface of the Riemann sphere. Stereographic projection enables us to explore solid geometry in a plane. Playing with the basic parameters
. These points lift to the surface of the Riemann sphere. Stereographic projection enables us to explore solid geometry in a plane. Playing with the basic parameters  ,
,  ,
,  , and
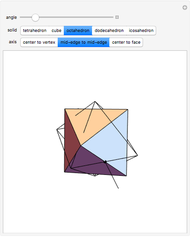
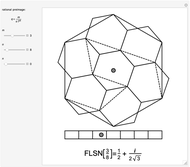
, and  , it is easy to see that points in the plane and on the sphere sometimes coalesce. When multiple points coincide, familiar polyhedra from solid geometry are formed by taking the convex hull of points. The following can be easily proved:
, it is easy to see that points in the plane and on the sphere sometimes coalesce. When multiple points coincide, familiar polyhedra from solid geometry are formed by taking the convex hull of points. The following can be easily proved:
If either  or
or  , the sphere figures of octahedral and icosahedral symmetry are the octahedron and icosahedron, respectively.
, the sphere figures of octahedral and icosahedral symmetry are the octahedron and icosahedron, respectively.
In dihedral-3 symmetry,

determines an "accidental" octahedron. Surprisingly, in the octahedral kaleidoscope, a point of the same magnitude

determines a cube.
For icosahedral symmetry, the point

determines a dodecahedron.
From numerical exploration, it seems possible that points
 ,
,
 ,
,

determine the cuboctahedron, icosahedron, and icosidodecahedron in the respective symmetry groups. The validity of these symbolic relations can be checked explicitly by trigonometry in a plane determined by one particular projective ray and the vertical axis of the Riemann sphere.
References
[1] S. L. Altmann, Rotations, Quaternions, and Double Groups, New York: Dover Publications, 2005.
[2] F. Klein, Vorlesungen über das Ikosaeder: und die Auflösung der Gleichungen vom fünften Grade, Liepzig: Teubner, 1884.
[3] H. Bethe, "Termaufspaltung in Kristallen," Annalen der Physik, 395(2), 1929 pp. 133–208. doi:10.1002/andp.19293950202.
Permanent Citation